Exam 7: RL and RC Circuits
Exam 1: Voltage, Current, Power, and Sources10 Questions
Exam 2: Circuit Laws10 Questions
Exam 3: Circuit Analysis Methods20 Questions
Exam 4: Circuit Theorems13 Questions
Exam 5: Operational Amplifier Circuits7 Questions
Exam 6: Capacitors and Inductors10 Questions
Exam 7: RL and RC Circuits14 Questions
Exam 8: RLC Circuits8 Questions
Exam 9: Phasors and Impedances8 Questions
Exam 10: Analysis of Phasor Transformed Circuits15 Questions
Exam 11: AC Power5 Questions
Exam 12: Three-Phase Systems6 Questions
Exam 13: Magnetically Coupled Circuits6 Questions
Exam 14: The Laplace Transform19 Questions
Exam 15: Circuits Analysis in the S-Domain26 Questions
Select questions type
The switch in the circuit shown below has been opened for a long time before it is closed at . Find the current through the inductor for . Assume .
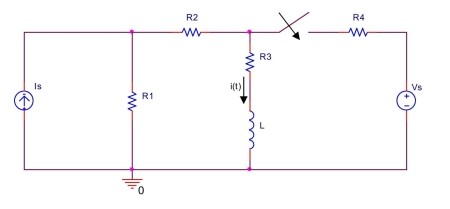
Free
(Essay)
4.7/5  (37)
(37)
Correct Answer:
From Is,
From V
switch 1 in the circuit shown below has been closed for a long time before it is opened at t = 0 and the switch 2 has been opened for a long time before it is closed at t = 0. Find the voltage across the capacitor for . Assume , .

Free
(Essay)
4.7/5  (36)
(36)
Correct Answer:
The switch in the circuit shown below has been opened for a long time before it is closed at . Find the current through the inductor for . Assume .
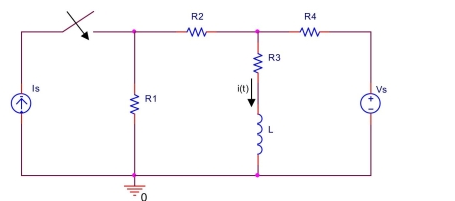
Free
(Essay)
4.7/5  (38)
(38)
Correct Answer:
From ,
From ,
the switch in the circuit shown below has been closed for a long time before it is opened at t . Find the current through the inductor for . Assume , . 
(Essay)
4.7/5  (27)
(27)
switch in the circuit shown below has been opened for a long time before it is closed at t . Find the voltage across the capacitor for . Assume .
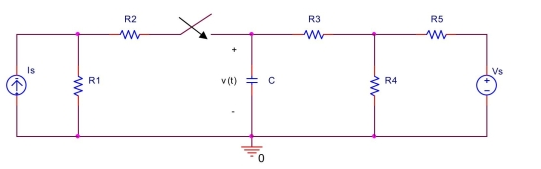
(Essay)
4.8/5  (30)
(30)
The switch in the circuit shown below has been closed for a long time before it is opened at t . Find the voltage across the capacitor for . Assume .
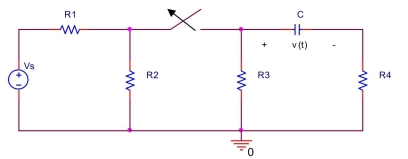
(Essay)
4.8/5  (40)
(40)
the switch in the circuit shown below has been closed for a long time before it is opened at t . Find the voltage across the capacitor for . Assume . 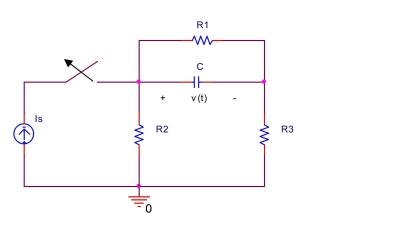
(Essay)
4.7/5  (35)
(35)
the switch in the circuit shown below has been closed for a long time before it is opened at t . Find the voltage across the capacitor for . Assume .
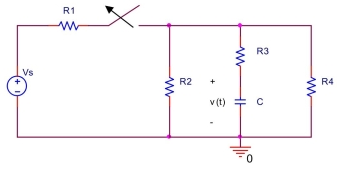
(Essay)
4.9/5  (42)
(42)
The switch in the circuit shown below has been closed for a long time before it is opened at . Find the current through the inductor for . Assume .
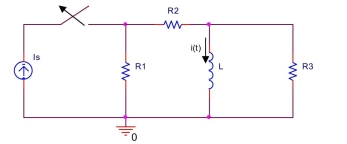
(Essay)
4.8/5  (28)
(28)
The switch in the circuit shown below has been closed for a long time before it is opened at t . Find the voltage across the capacitor for . Assume . 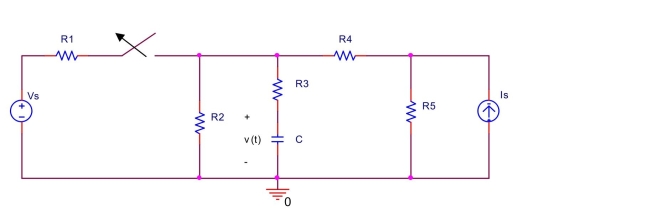
(Essay)
4.9/5  (44)
(44)
The switch in the circuit shown below has been closed for a long time before it is opened at t . Find the voltage across the capacitor for . Assume .
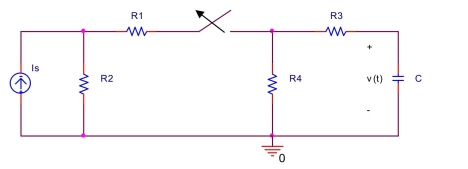
(Essay)
4.7/5  (37)
(37)
the switch in the circuit shown below has been closed for a long time before it is opened at t . Find the voltage across the capacitor for . Assume .
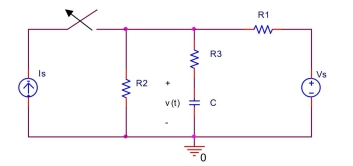
(Essay)
4.8/5  (38)
(38)
The switch in the circuit shown below has been closed for a long time before it is opened at . Find the current through the inductor for . Assume .
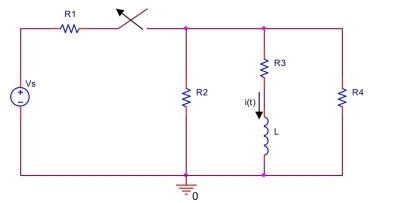
(Essay)
4.9/5  (28)
(28)
The switch in the circuit shown below has been closed for a long time before it is opened at . Find the current through the inductor for . Assume . 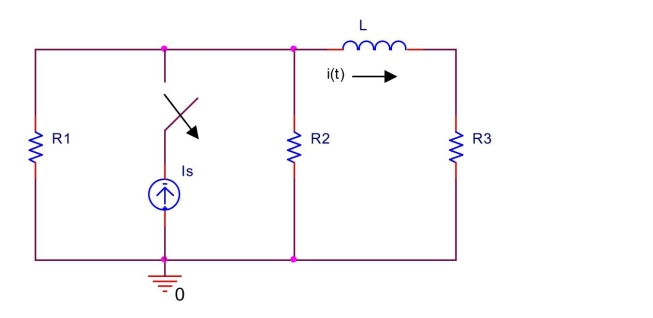
(Essay)
4.8/5  (38)
(38)
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)