Exam 7: Random Variables and Discrete Probability Distributions

The covariance between two investments of a portfolio is equal to the sum of the variances of the investments.

Number of Birds Alana and Eva are sisters.Let X denote the number of birds that Alana may have in the next two years,and let Y denote the number of birds Eva may have during the same period.The marginal probability distributions of X and Y are shown below. ​ ​ -{Number of Birds Narrative} Find the probability distribution of the random variable XY.

The expected return of a portfolio of two investments will be equal to the sum of the expected returns of the two investments plus twice the covariance between the investments.

The length of time for which an apartment in a large complex remains vacant is a discrete random variable.

If n = 20 and p = 0.70,then the standard deviation of the binomial distribution is

The number of accidents that occur annually on a busy stretch of highway is an example of:

Gym Visits Let X represent the number of times a student visits a gym in a one month period.Assume that the probability distribution of X is as follows: ​ ​ -{Gym Visits Narrative} What is the probability that the student visits the gym at least once in a month?

Number of Birds Alana and Eva are sisters.Let X denote the number of birds that Alana may have in the next two years,and let Y denote the number of birds Eva may have during the same period.The marginal probability distributions of X and Y are shown below. ​ ​ -{Number of Birds Narrative} Compute the covariance between X and Y.

Mobile Phones Sales After analyzing sales data,the owner of a Mobile Phone store produced the following joint probability distribution of the number of iPhones (X)and Blackberries (Y)sold daily. ​ ​ -{Mobile Phones Sales Narrative} Find the marginal probability distribution of the number of iPhones sold daily.

Given a binomial random variable with n = 20 and p = 0.60,find the following probabilities using the binomial table. a.P(X ≤ 13) b.P(X ≥ 15) c.P(X = 17) d.P(11 ≤ X ≤ 14) e.P(11 < X < 14)

If X and Y are two variables with σ_x = 3.8,σ_y = 4.2,and COV(X,Y)= −0.25,then V(X + Y)= 31.58.

Car Sales The joint probability distribution of variables X and Y is shown in the table below.Rebecca and Rachel are car salespeople.Let X denote the number of cars that Rebecca will sell in a month,and let Y denote the number of cars Rachel will sell in a month. ​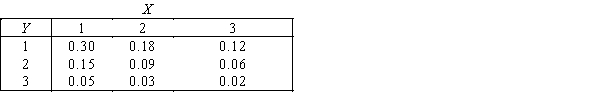 ​ -{Car Sales Narrative} Develop the probability distribution of X + Y.

Elizabeth's Portfolio ​ Elizabeth has decided to form a portfolio by putting 30% of her money into stock 1 and 70% into stock 2.She assumes that the expected returns will be 10% and 18%,respectively,and that the standard deviations will be 15% and 24%,respectively. ​ ​ -{Elizabeth's Portfolio Narrative} Find the expected mean of the portfolio.

The variance of a binomial distribution for which n = 100 and p = 0.20 is:

Shopping Outlet A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below. ​ ​ -{Shopping Outlet Narrative} Find the variance and standard deviation of the number of stores entered.

The ____________________ and the ____________________ both measure the relationship between two random variables X and Y.

Montana Highways A recent survey in Montana revealed that 60% of the vehicles traveling on highways,where speed limits are posted at 70 miles per hour,were exceeding the limit.Suppose you randomly record the speeds of ten vehicles traveling on US 131 where the speed limit is 70 miles per hour.Let X denote the number of vehicles that were exceeding the limit. ​ ​ -{Montana Highways Narrative} Find the standard deviation of number of vehicles that are traveling on Montana highways and exceeding the speed limit.

The dean of students conducted a survey on campus.Grade point average (GPA)is an example of a(n)____________________ random variable.

The largest value that a Poisson random variable X can have is n.

Shopping Outlet A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below. ​ ​ -{Shopping Outlet Narrative} Calculate the expected value of Y directly from the probability distribution of Y.