Exam 15: Inference With Regression Models

A real estate analyst believes that the three main factors that influence an apartment's rent in a college town are the number of bedrooms, the number of bathrooms, and the apartment's square footage. For 40 apartments, she collects data on the rent (y, in $), the number of bedrooms (x₁), the number of bathrooms (x₂), and its square footage (x₃). She estimates the following model: Rent = β₀ + β₁Bedroom + β₂Bath + β₃Sqft + ε. The following table shows a portion of the regression results. 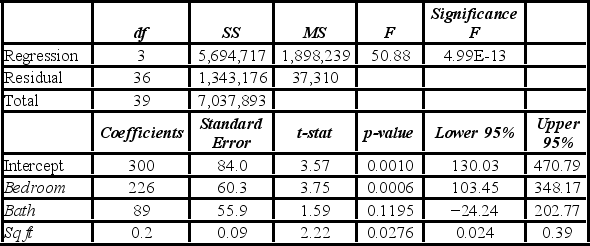Which of the following are the hypotheses to test if the explanatory variables Bath and Sqft are jointly significant in explaining Rent?

A marketing analyst wants to examine the relationship between sales (in $1,000s) and advertising (in $100s) for firms in the food and beverage industry and so collects monthly data for 25 firms. He estimates the model: Sales = β₀ + β₁ Advertising + ε. The following table shows a portion of the regression results. When testing whether the slope coefficient differs from 3, the value of the test statistic is ________.

When estimating y = β₀ + β₁x₁ + β₂x₂ + β₃x₃ + ε, you wish to test H₀: β₁ = β₂ versus H_A: β₁ ≠ β₂. The value of the test statistic is F_(1,30) = 7.75 and its associated p-value is 0.0092. At the 1% significance level, the conclusion is to ________.

The restricted model is a reduced model where the coefficients that are restricted under the null hypothesis are estimated.

Which of the following is the correct expression for computing a 100(1 - α)% prediction interval for an individual value of y?

A researcher analyzes the factors that may influence amusement park attendance. She estimates the following model: Attendance = β₀ + β₁Price + β₂Temperature + β₃Rides + ε, where Attendance is the daily attendance (in 1,000s), Price is the gate price (in $), Temperature is the average daily temperature (in °F), and Rides is the number of rides at the amusement park. A portion of the regression results is shown in the accompanying table. 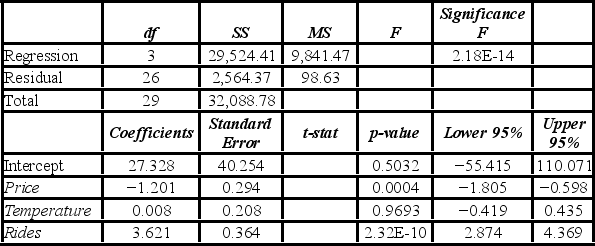When testing whether Rides is significant at the 1% significance level, she ________.

Serial correlation is typically observed in ________.

If the confidence interval does not contain the value zero, then the ________ variable associated with the regression coefficient is significant.

A researcher analyzes the factors that may influence amusement park attendance. She estimates the following model: Attendance = β₀ + β₁Price + β₂Temperature + β₃Rides + ε, where Attendance is the daily attendance (in 1,000s), Price is the gate price (in $), Temperature is the average daily temperature (in °F), and Rides is the number of rides at the amusement park. A portion of the regression results is shown in the accompanying table. 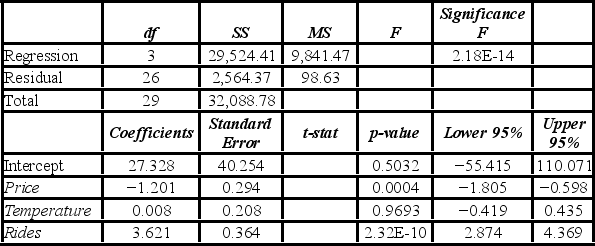Which of the following are the hypotheses to test if the explanatory variables Temperature and Rides are jointly significant in explaining Attendance?

A real estate analyst believes that the three main factors that influence an apartment's rent in a college town are the number of bedrooms, the number of bathrooms, and the apartment's square footage. For 40 apartments, she collects data on the rent (y, in $), the number of bedrooms (x₁), the number of bathrooms (x₂), and its square footage (x₃). She estimates the following model: Rent = β₀ + β₁Bedroom + β₂Bath + β₃Sqft + ε. The following table shows a portion of the regression results. 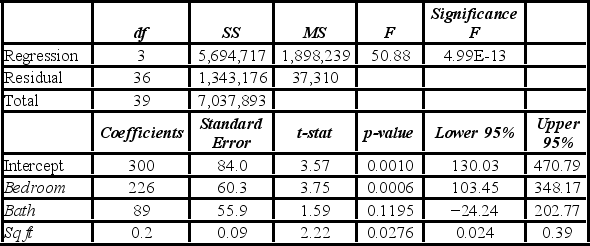When testing whether the explanatory variables are jointly significant at the 5% level, she ________.

It is common to refer to the interval estimate for an individual value of y as the ________ interval.

In regression, the two types of interval estimates concerning y are called ________.

Refer to below regression results. 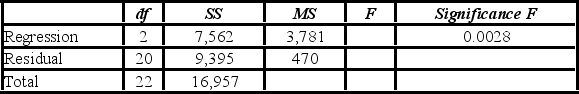When testing the overall significance of the regression model at the 5% level given a critical value of F_0.05,(2.20) = 3.49, the decision is to ________.

A manager at a local bank analyzed the relationship between monthly salary (y, in $) and length of service (x, measured in months) for 30 employees. The following table summarizes a portion of the regression results: a. Specify the competing hypotheses to determine whether Service is significant in explaining Salary. B) At the 5% significance level, is Service significant? Explain.

A researcher analyzes the factors that may influence amusement park attendance. She estimates the following model: Attendance = β₀ + β₁Price + β₂Temperature + β₃Rides + ε, where Attendance is the daily attendance (in 1,000s), Price is the gate price (in $), Temperature is the average daily temperature (in °F), and Rides is the number of rides at the amusement park. A portion of the regression results is shown in the accompanying table. 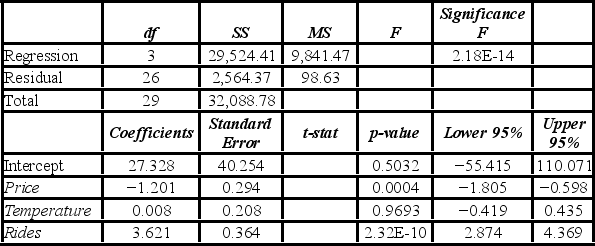Which of the following is the value of the test statistic for testing the joint significance of the linear regression model?

A simple linear regression, Sales = β₀ + β₁ Advertising + ε, is estimated using cross-sectional data from 10 firms. The resulting residuals e, along with the values of the explanatory variable Advertising (x, in $100s), are shown in the accompanying table. 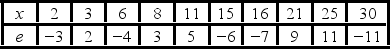a. Graph the residuals e against Advertising (x) and look for any discernible pattern. B) Which assumption is being violated? Discuss its consequences and suggest a possible remedy.

The term BLUE stands for Best Linear Unbiased Estimator.

A marketing analyst wants to examine the relationship between sales (in $1,000s) and advertising (in $100s) for firms in the food and beverage industry and so collects monthly data for 25 firms. He estimates the model: Sales = β₀ + β₁ Advertising + ε. The following table shows a portion of the regression results. Which of the following are the competing hypotheses used to test whether Advertising is significant in predicting Sales?

A researcher analyzes the factors that may influence amusement park attendance. She estimates the following model: Attendance = β₀ + β₁ Price + β₂ Temperature + β₃ Rides + ε, where Attendance is the daily attendance (in 1,000s), Price is the gate price (in $), Temperature is the average daily temperature (in ^oF), and Rides is the number of rides at the amusement park. A portion of the regression results is shown in the accompanying table. 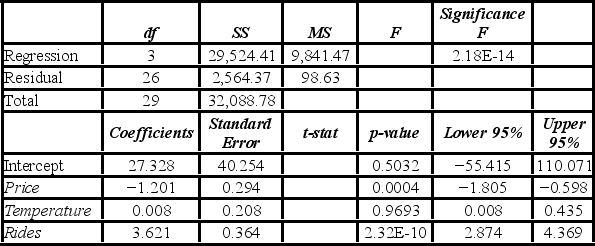When testing whether Price is significant in explaining Attendance, the value of the test statistic is ________.

A real estate analyst believes that the three main factors that influence an apartment's rent in a college town are the number of bedrooms, the number of bathrooms, and the apartment's square footage. For 40 apartments, she collects data on the rent (y, in $), the number of bedrooms (x₁), the number of bathrooms (x₂), and its square footage (x₃). She estimates the following model: Rent = β₀ + β₁Bedroom + β₂Bath + β₃Sqft + ε. The following table shows a portion of the regression results. 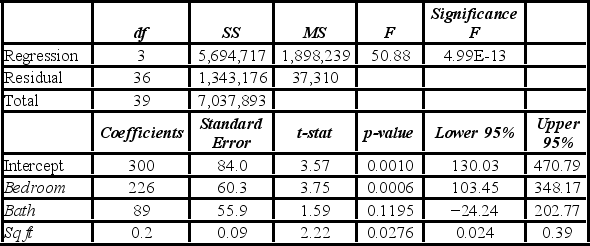When testing whether the explanatory variables jointly influence the response variable, the null hypothesis is ________.