Exam 4: Introduction to Probability
Exam 1: Data and Statistics85 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Displays112 Questions
Exam 3: Descriptive Statistics: Numerical Measures139 Questions
Exam 4: Introduction to Probability129 Questions
Exam 5: Discrete Probability Distributions150 Questions
Exam 6: Continuous Probability Distributions144 Questions
Exam 7: Sampling and Sampling Distributions119 Questions
Exam 8: Interval Estimation118 Questions
Exam 9: Hypothesis Tests118 Questions
Exam 10: Inference About Means and Proportions With Two Populations127 Questions
Exam 11: Inferences About Population Variances113 Questions
Exam 12: Tests of Goodness of Fit, Independence and Multiple Proportions76 Questions
Exam 13: Experimental Design and Analysis of Variance125 Questions
Exam 14: Simple Linear Regression103 Questions
Exam 15: Multiple Regression109 Questions
Exam 16: Regression Analysis: Model Building82 Questions
Exam 17: Time Series Analysis and Forecasting80 Questions
Exam 18: Nonparametric Methods83 Questions
Exam 19: Statistical Methods for Quality Control75 Questions
Exam 20: Decision Analysis71 Questions
Exam 21: Sample Survey68 Questions
Select questions type
Through a telephone survey, a low-interest bank credit card is offered to 400 households. The responses are as follows. 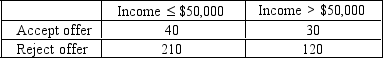 a. Develop a joint probability table and show the marginal probabilities..
b. What is the probability of a household whose income exceeds $50,000 and who rejects the offer?
c. If income is < $50,000, what is the probability the offer will be accepted?
d. If the offer is accepted, what is the probability that income exceeds $50,000?
a. Develop a joint probability table and show the marginal probabilities..
b. What is the probability of a household whose income exceeds $50,000 and who rejects the offer?
c. If income is < $50,000, what is the probability the offer will be accepted?
d. If the offer is accepted, what is the probability that income exceeds $50,000?
Free
(Essay)
4.8/5  (34)
(34)
Correct Answer:
a. 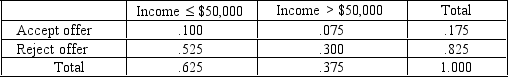 b. .3
b. .3
c. .16
d. .4286
If A and B are mutually exclusive events with P(A) = 0.3 and P(B) = 0.5, then P(A B) =
Free
(Multiple Choice)
4.8/5  (41)
(41)
Correct Answer:
C
Assume you are taking two courses this semester (A and B). Based on your opinion, you believe the probability that you will pass course A is 0.835; the probability that you will pass both courses is 0.276. You further believe the probability that you will pass at least one of the courses is 0.981.
a.What is the probability that you will pass course B?
b.Is the passing of the two courses independent events? Use probability information to justify your answer.
c.Are the events of passing the courses mutually exclusive? Explain.
d.What method of assigning probabilities did you use?
Free
(Essay)
4.9/5  (37)
(37)
Correct Answer:
a.0.422
b.No, P(A|B) = 0.654 P(A)
c.No, the probability of their intersection is not zero.d.the subjective method
If a penny is tossed four times and comes up heads all four times, the probability of heads on the fifth trial is
(Multiple Choice)
4.9/5  (43)
(43)
Events A and B are mutually exclusive. Which of the following statements is also true?
(Multiple Choice)
4.9/5  (41)
(41)
In a recent survey in a Statistics class, it was determined that only 60% of the students attend class on Fridays. From past data it was noted that 98% of those who went to class on Fridays pass the course, while only 20% of those who did not go to class on Fridays passed the course.
a.What percentage of students is expected to pass the course?
b.Given that a person passes the course, what is the probability that he/she attended classes on Fridays?
(Short Answer)
4.8/5  (34)
(34)
A lottery is conducted using three urns. Each urn contains chips numbered from 0 to 9. One chip is selected at random from each urn. The total number of sample points in the sample space is
(Multiple Choice)
4.9/5  (29)
(29)
A very short quiz has one multiple-choice question with five possible choices (a, b, c, d, and e) and one true or false question. Assume you are taking the quiz but do not have any idea what the correct answer is to either question, but you mark an answer anyway.
a.What is the probability that you have given the correct answer to both questions?
b.What is the probability that only one of the two answers is correct?
c.What is the probability that neither answer is correct?
d.What is the probability that only your answer to the multiple-choice question is correct?
e.What is the probability that you have only answered the true or false question correctly?
(Short Answer)
4.9/5  (40)
(40)
If a coin is tossed three times, the likelihood of obtaining three heads in a row is
(Multiple Choice)
4.7/5  (29)
(29)
There are two more assignments in a class before its end, and if you get an A on at least one of them, you will get an A for the semester. Your subjective assessment of your performance is 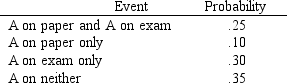 a. What is the probability of getting an A on the paper?
b. What is the probability of getting an A on the exam?
c. What is the probability of getting an A in the course?
d. Are the grades on the assignments independent?
a. What is the probability of getting an A on the paper?
b. What is the probability of getting an A on the exam?
c. What is the probability of getting an A in the course?
d. Are the grades on the assignments independent?
(Short Answer)
4.7/5  (40)
(40)
In an experiment, events A and B are mutually exclusive. If P(A) = 0.6, then the probability of B
(Multiple Choice)
4.9/5  (34)
(34)
Given that event E has a probability of 0.25, the probability of the complement of event E
(Multiple Choice)
4.8/5  (27)
(27)
All the employees of ABC Company are assigned ID numbers. The ID number consists of the first letter of an employee's last name, followed by four numbers.
a.How many possible different ID numbers are there?
b.How many possible different ID numbers are there for employees whose last name starts with an "A"?
(Short Answer)
4.9/5  (34)
(34)
Assume your favorite football team has 2 games left to finish the season. The outcome of each game can be win, lose or tie. The number of possible outcomes is
(Multiple Choice)
4.7/5  (33)
(33)
Assume you have applied for two scholarships, a Merit scholarship (M) and an Athletic scholarship (A). The probability that you receive an Athletic scholarship is 0.18. The probability of receiving both scholarships is 0.11. The probability of getting at least one of the scholarships is 0.3.
a.What is the probability that you will receive a Merit scholarship?
b.Are events A and M mutually exclusive? Why or why not? Explain.
c.Are the two events, A and M, independent? Explain, using probabilities.
d.What is the probability of receiving the Athletic scholarship given that you have been awarded the Merit scholarship?
e.What is the probability of receiving the Merit scholarship given that you have been awarded the Athletic scholarship?
(Essay)
4.9/5  (39)
(39)
In the two upcoming basketball games, the probability that UTC will defeat Marshall is 0.63, and the probability that UTC will defeat Furman is 0.55. The probability that UTC will defeat both opponents is 0.3465.
a.What is the probability that UTC will defeat Furman given that they defeat Marshall?
b.What is the probability that UTC will win at least one of the games?
c.What is the probability of UTC winning both games?
d.Are the outcomes of the games independent? Explain and substantiate your answer.
(Essay)
4.9/5  (32)
(32)
If A and B are independent events with P(A) = 0.05 and P(B) = 0.65, then P(A|B) =
(Multiple Choice)
4.8/5  (37)
(37)
The following probability model describes the number of snowstorms for Washington, D.C. for a given year:  The probability of 7 or more snowstorms in a year is 0.
a. What is the probability of more than 2 but less than 5 snowstorms?
b. Given this a particularly cold year (in which 2 snowstorms have already been observed), what is the conditional probability that 4 or more snowstorms will be observed?
c. If at the beginning of winter there is a snowfall, what is the probability of at least one more snowstorm before winter is over?
The probability of 7 or more snowstorms in a year is 0.
a. What is the probability of more than 2 but less than 5 snowstorms?
b. Given this a particularly cold year (in which 2 snowstorms have already been observed), what is the conditional probability that 4 or more snowstorms will be observed?
c. If at the beginning of winter there is a snowfall, what is the probability of at least one more snowstorm before winter is over?
(Short Answer)
4.9/5  (28)
(28)
Of five letters (A, B, C, D, and E), two letters are to be selected at random. How many possible selections are there?
(Multiple Choice)
4.7/5  (40)
(40)
Showing 1 - 20 of 129
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)