Exam 12: Tests of Goodness of Fit, Independence and Multiple Proportions
Exam 1: Data and Statistics85 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Displays112 Questions
Exam 3: Descriptive Statistics: Numerical Measures139 Questions
Exam 4: Introduction to Probability129 Questions
Exam 5: Discrete Probability Distributions150 Questions
Exam 6: Continuous Probability Distributions144 Questions
Exam 7: Sampling and Sampling Distributions119 Questions
Exam 8: Interval Estimation118 Questions
Exam 9: Hypothesis Tests118 Questions
Exam 10: Inference About Means and Proportions With Two Populations127 Questions
Exam 11: Inferences About Population Variances113 Questions
Exam 12: Tests of Goodness of Fit, Independence and Multiple Proportions76 Questions
Exam 13: Experimental Design and Analysis of Variance125 Questions
Exam 14: Simple Linear Regression103 Questions
Exam 15: Multiple Regression109 Questions
Exam 16: Regression Analysis: Model Building82 Questions
Exam 17: Time Series Analysis and Forecasting80 Questions
Exam 18: Nonparametric Methods83 Questions
Exam 19: Statistical Methods for Quality Control75 Questions
Exam 20: Decision Analysis71 Questions
Exam 21: Sample Survey68 Questions
Select questions type
Exhibit 12-2
Last school year, the student body of a local university consisted of 30% freshmen, 24% sophomores, 26% juniors, and 20% seniors. A sample of 300 students taken from this year's student body showed the following number of students in each classification.  We are interested in determining whether or not there has been a significant change in the classifications between the last school year and this school year.
-Refer to Exhibit 12-2. The expected frequency of seniors is
We are interested in determining whether or not there has been a significant change in the classifications between the last school year and this school year.
-Refer to Exhibit 12-2. The expected frequency of seniors is
Free
(Multiple Choice)
4.8/5  (33)
(33)
Correct Answer:
A
Dr. Ross' diet pills are supposed to cause significant weight loss. The following table shows the results of a recent study where some individuals took the diet pills and some did not. 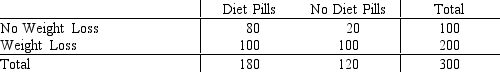 With 95% confidence, test to see if losing weight is dependent on taking the diet pills.
With 95% confidence, test to see if losing weight is dependent on taking the diet pills.
Free
(Short Answer)
4.9/5  (31)
(31)
Correct Answer:
Chi-square = 25 > 5.99; is dependent
Exhibit 12-1
When individuals in a sample of 150 were asked whether or not they supported capital punishment, the following information was obtained.  We are interested in determining whether or not the opinions of the individuals (as to Yes, No, and No Opinion) are uniformly distributed.
-Refer to Exhibit 12-1. The expected frequency for each group is
We are interested in determining whether or not the opinions of the individuals (as to Yes, No, and No Opinion) are uniformly distributed.
-Refer to Exhibit 12-1. The expected frequency for each group is
Free
(Multiple Choice)
4.8/5  (36)
(36)
Correct Answer:
D
Exhibit 12-3
In order to determine whether or not a particular medication was effective in curing the common cold, one group of patients was given the medication, while another group received sugar pills. The results of the study are shown below.  We are interested in determining whether or not the medication was effective in curing the common cold.
-Refer to Exhibit 12-3. The expected frequency of those who received medication and were cured is
We are interested in determining whether or not the medication was effective in curing the common cold.
-Refer to Exhibit 12-3. The expected frequency of those who received medication and were cured is
(Multiple Choice)
4.9/5  (33)
(33)
Exhibit 12-1
When individuals in a sample of 150 were asked whether or not they supported capital punishment, the following information was obtained.  We are interested in determining whether or not the opinions of the individuals (as to Yes, No, and No Opinion) are uniformly distributed.
-Refer to Exhibit 12-1. The number of degrees of freedom associated with this problem is
We are interested in determining whether or not the opinions of the individuals (as to Yes, No, and No Opinion) are uniformly distributed.
-Refer to Exhibit 12-1. The number of degrees of freedom associated with this problem is
(Multiple Choice)
4.8/5  (39)
(39)
Exhibit 12-1
When individuals in a sample of 150 were asked whether or not they supported capital punishment, the following information was obtained.  We are interested in determining whether or not the opinions of the individuals (as to Yes, No, and No Opinion) are uniformly distributed.
-Refer to Exhibit 12-1. The conclusion of the test is that the
We are interested in determining whether or not the opinions of the individuals (as to Yes, No, and No Opinion) are uniformly distributed.
-Refer to Exhibit 12-1. The conclusion of the test is that the
(Multiple Choice)
4.8/5  (23)
(23)
Exhibit 12-6
The following shows the number of individuals in a sample of 300 who indicated they support the new tax proposal.  We are interested in determining whether or not the opinions of the individuals of the three groups are uniformly distributed.
-The personnel department of a large corporation reported sixty resignations during the last year. The following table groups these resignations according to the season in which they occurred:
We are interested in determining whether or not the opinions of the individuals of the three groups are uniformly distributed.
-The personnel department of a large corporation reported sixty resignations during the last year. The following table groups these resignations according to the season in which they occurred:  Test to see if the number of resignations is uniform over the four seasons.Let = 0.05.
Test to see if the number of resignations is uniform over the four seasons.Let = 0.05.
(Essay)
4.9/5  (33)
(33)
Exhibit 12-5
The table below gives beverage preferences for random samples of teens and adults. 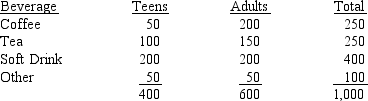 We are asked to test for independence between age (i.e., adult and teen) and drink preferences.
-Refer to Exhibit 12-5. The expected number of adults who prefer coffee is
We are asked to test for independence between age (i.e., adult and teen) and drink preferences.
-Refer to Exhibit 12-5. The expected number of adults who prefer coffee is
(Multiple Choice)
4.8/5  (29)
(29)
Five hundred randomly selected automobile owners were questioned on the main reason they had purchased their current automobile. The results are given below.  a.State the null and alternative hypotheses for a contingency table test.
b.State the decision rule, using a .10 level of significance.
c.Calculate the chi-square test statistic.
d.Give your conclusion for this test.
a.State the null and alternative hypotheses for a contingency table test.
b.State the decision rule, using a .10 level of significance.
c.Calculate the chi-square test statistic.
d.Give your conclusion for this test.
(Essay)
4.9/5  (41)
(41)
Exhibit 12-4
In the past, 35% of the students at ABC University were in the Business College, 35% of the students were in the Liberal Arts College, and 30% of the students were in the Education College. To see whether or not the proportions have changed, a sample of 300 students was taken. Ninety of the sample students are in the Business College, 120 are in the Liberal Arts College, and 90 are in the Education College.
-Refer to Exhibit 12-4. The expected frequency for the Business College is
(Multiple Choice)
4.8/5  (42)
(42)
Exhibit 12-4
In the past, 35% of the students at ABC University were in the Business College, 35% of the students were in the Liberal Arts College, and 30% of the students were in the Education College. To see whether or not the proportions have changed, a sample of 300 students was taken. Ninety of the sample students are in the Business College, 120 are in the Liberal Arts College, and 90 are in the Education College.
-Refer to Exhibit 12-4. The calculated value for the test statistic equals
(Multiple Choice)
4.8/5  (28)
(28)
Exhibit 12-5
The table below gives beverage preferences for random samples of teens and adults. 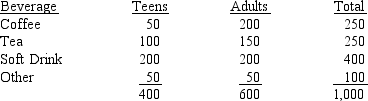 We are asked to test for independence between age (i.e., adult and teen) and drink preferences.
-Refer to Exhibit 12-5. The result of the test is that the
We are asked to test for independence between age (i.e., adult and teen) and drink preferences.
-Refer to Exhibit 12-5. The result of the test is that the
(Multiple Choice)
5.0/5  (37)
(37)
The data below represents the fields of specialization for a randomly selected sample of undergraduate students. Test to determine whether there is a significant difference in the fields of specialization between regions of the country. Use a .05 level of significance. 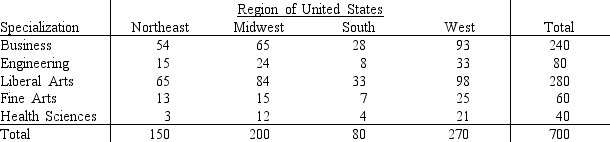 a.State the critical value of the chi-square random variable for this test of independence of categories.
b.Calculate the value of the test statistic.
c.What is the conclusion for this test?
a.State the critical value of the chi-square random variable for this test of independence of categories.
b.Calculate the value of the test statistic.
c.What is the conclusion for this test?
(Essay)
5.0/5  (45)
(45)
Exhibit 12-5
The table below gives beverage preferences for random samples of teens and adults. 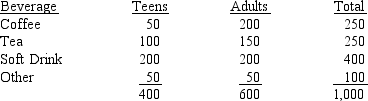 We are asked to test for independence between age (i.e., adult and teen) and drink preferences.
-Refer to Exhibit 12-5. The calculated value for this test for independence is
We are asked to test for independence between age (i.e., adult and teen) and drink preferences.
-Refer to Exhibit 12-5. The calculated value for this test for independence is
(Multiple Choice)
4.8/5  (29)
(29)
The following table shows the results of a study on smoking and three illnesses. We are interested in determining if the proportions smokers in the three categories are different from each other.  a.Provide the null and the alternative hypotheses.
b.Determine the expected frequencies.
c.Compute the sample proportions.
d.Compute the critical values (CVij).
e.Give your conclusions by providing numerical reasoning.
a.Provide the null and the alternative hypotheses.
b.Determine the expected frequencies.
c.Compute the sample proportions.
d.Compute the critical values (CVij).
e.Give your conclusions by providing numerical reasoning.
(Essay)
5.0/5  (34)
(34)
Exhibit 12-6
The following shows the number of individuals in a sample of 300 who indicated they support the new tax proposal. 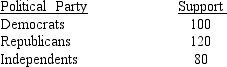 We are interested in determining whether or not the opinions of the individuals of the three groups are uniformly distributed.
-Before the presidential debates, it was expected that the percentages of registered voters in favor of various candidates to be as follows.
We are interested in determining whether or not the opinions of the individuals of the three groups are uniformly distributed.
-Before the presidential debates, it was expected that the percentages of registered voters in favor of various candidates to be as follows.  After the presidential debates, a random sample of 1200 voters showed that 540 favored the Democratic candidate; 480 were in favor of the Republican candidate; 40 were in favor of the Independent candidate, and 140 were undecided. At a 5% level of significance, test to see if the proportion of voters has changed.
After the presidential debates, a random sample of 1200 voters showed that 540 favored the Democratic candidate; 480 were in favor of the Republican candidate; 40 were in favor of the Independent candidate, and 140 were undecided. At a 5% level of significance, test to see if the proportion of voters has changed.
(Essay)
4.7/5  (35)
(35)
Exhibit 12-6
The following shows the number of individuals in a sample of 300 who indicated they support the new tax proposal. 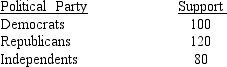 We are interested in determining whether or not the opinions of the individuals of the three groups are uniformly distributed.
-The number of categories of outcomes per trial for a multinomial probability distribution is
We are interested in determining whether or not the opinions of the individuals of the three groups are uniformly distributed.
-The number of categories of outcomes per trial for a multinomial probability distribution is
(Multiple Choice)
4.9/5  (33)
(33)
Exhibit 12-6
The following shows the number of individuals in a sample of 300 who indicated they support the new tax proposal. 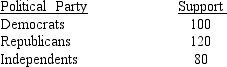 We are interested in determining whether or not the opinions of the individuals of the three groups are uniformly distributed.
-Refer to Exhibit 12-6. The expected frequency for each group is
We are interested in determining whether or not the opinions of the individuals of the three groups are uniformly distributed.
-Refer to Exhibit 12-6. The expected frequency for each group is
(Multiple Choice)
4.8/5  (32)
(32)
Before the start of the Winter Olympics, it was expected that the percentages of medals awarded to the top contenders to be as follows.  Midway through the Olympics, of the 120 medals awarded, the following distribution was observed.
Midway through the Olympics, of the 120 medals awarded, the following distribution was observed.  We want to test to see if there is a significant difference between the expected and actual awards given.
a.Compute the test statistic.
b.Using the p-value approach, test to see if there is a significant difference between the expected and the actual values. Let = .05.
c.At 95% confidence, test for a significant difference using the critical value approach.
We want to test to see if there is a significant difference between the expected and actual awards given.
a.Compute the test statistic.
b.Using the p-value approach, test to see if there is a significant difference between the expected and the actual values. Let = .05.
c.At 95% confidence, test for a significant difference using the critical value approach.
(Essay)
4.8/5  (31)
(31)
Showing 1 - 20 of 76
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)