Exam 15: Multiple Regression
Exam 1: Data and Statistics85 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Displays112 Questions
Exam 3: Descriptive Statistics: Numerical Measures139 Questions
Exam 4: Introduction to Probability129 Questions
Exam 5: Discrete Probability Distributions150 Questions
Exam 6: Continuous Probability Distributions144 Questions
Exam 7: Sampling and Sampling Distributions119 Questions
Exam 8: Interval Estimation118 Questions
Exam 9: Hypothesis Tests118 Questions
Exam 10: Inference About Means and Proportions With Two Populations127 Questions
Exam 11: Inferences About Population Variances113 Questions
Exam 12: Tests of Goodness of Fit, Independence and Multiple Proportions76 Questions
Exam 13: Experimental Design and Analysis of Variance125 Questions
Exam 14: Simple Linear Regression103 Questions
Exam 15: Multiple Regression109 Questions
Exam 16: Regression Analysis: Model Building82 Questions
Exam 17: Time Series Analysis and Forecasting80 Questions
Exam 18: Nonparametric Methods83 Questions
Exam 19: Statistical Methods for Quality Control75 Questions
Exam 20: Decision Analysis71 Questions
Exam 21: Sample Survey68 Questions
Select questions type
In a multiple regression model, the error term is assumed to be a random variable with a mean of
Free
(Multiple Choice)
4.7/5  (31)
(31)
Correct Answer:
A
The following regression model has been proposed to predict sales at a furniture store. 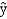 = 10 - 4x1 + 7x2 + 18x3
where
x1 = competitor's previous day's sales (in $1,000s)
x2 = population within 1 mile (in 1000s)
x3 = 1 if any form of advertising was used, 0 if otherwise
= 10 - 4x1 + 7x2 + 18x3
where
x1 = competitor's previous day's sales (in $1,000s)
x2 = population within 1 mile (in 1000s)
x3 = 1 if any form of advertising was used, 0 if otherwise 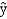 = sales (in $1,000s)
a.Fully interpret the meaning of the coefficient of x3.
b.Predict sales (in dollars) for a store with competitor's previous day's sale of $3,000, a population of 10,000 within 1 mile, and six radio advertisements.
= sales (in $1,000s)
a.Fully interpret the meaning of the coefficient of x3.
b.Predict sales (in dollars) for a store with competitor's previous day's sale of $3,000, a population of 10,000 within 1 mile, and six radio advertisements.
Free
(Essay)
4.9/5  (29)
(29)
Correct Answer:
a.When advertising was used, sales were higher by $18,000.b.$86,000
A variable that cannot be measured in terms of how much or how many but instead is assigned values to represent categories is called
Free
(Multiple Choice)
4.9/5  (39)
(39)
Correct Answer:
D
In multiple regression analysis, the correlation among the independent variables is termed
(Multiple Choice)
4.8/5  (38)
(38)
Exhibit 15-5
Below you are given a partial Excel output based on a sample of 25 observations.  -Refer to Exhibit 15-5. The estimated regression equation is
-Refer to Exhibit 15-5. The estimated regression equation is
(Multiple Choice)
4.9/5  (34)
(34)
Shown below is a partial Excel output from a regression analysis. 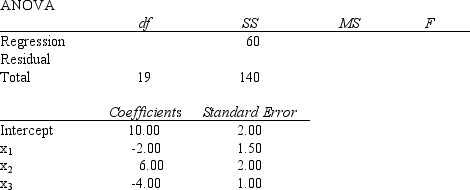 a.Use the above results and write the regression equation.
b.Compute the coefficient of determination and fully interpret its meaning.
c.Is the regression model significant? Perform an F test and let = 0.05.
d.At = 0.05, test to see if there is a relation between x1 and y.
e.At = 0.05, test to see if there is a relation between x3 and y.
a.Use the above results and write the regression equation.
b.Compute the coefficient of determination and fully interpret its meaning.
c.Is the regression model significant? Perform an F test and let = 0.05.
d.At = 0.05, test to see if there is a relation between x1 and y.
e.At = 0.05, test to see if there is a relation between x3 and y.
(Essay)
4.8/5  (32)
(32)
The adjusted multiple coefficient of determination is adjusted for
(Multiple Choice)
4.9/5  (30)
(30)
Exhibit 15-5
Below you are given a partial Excel output based on a sample of 25 observations.  -Refer to Exhibit 15-5. Carry out the test of significance for the parameter 1 at the 5% level. The null hypothesis should be
-Refer to Exhibit 15-5. Carry out the test of significance for the parameter 1 at the 5% level. The null hypothesis should be
(Multiple Choice)
4.9/5  (30)
(30)
Below you are given a partial ANOVA table based on a sample of 12 observations relating the number of personal computers sold by a computer shop per month (y), unit price (x1 in $1,000) and the number of advertising spots (x2) they used on a local television station.  a.At = 0.05 level of significance, test to determine if the model is significant. That is, determine if there exists a significant relationship between the independent variables and the dependent variable.
b.Determine the multiple coefficient of determination.
c.Determine the adjusted multiple coefficient of determination.
a.At = 0.05 level of significance, test to determine if the model is significant. That is, determine if there exists a significant relationship between the independent variables and the dependent variable.
b.Determine the multiple coefficient of determination.
c.Determine the adjusted multiple coefficient of determination.
(Essay)
4.9/5  (38)
(38)
Exhibit 15-8
The following estimated regression model was developed relating yearly income (y in $1,000s) of 30 individuals with their age (x1) and their gender (x2) (0 if male and 1 if female). 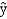 = 30 + 0.7x1 + 3x2
Also provided are SST = 1,200 and SSE = 384.
-A regression model involved 18 independent variables and 200 observations. The critical value of t for testing the significance of each of the independent variable's coefficients will have
= 30 + 0.7x1 + 3x2
Also provided are SST = 1,200 and SSE = 384.
-A regression model involved 18 independent variables and 200 observations. The critical value of t for testing the significance of each of the independent variable's coefficients will have
(Multiple Choice)
4.8/5  (31)
(31)
As the goodness of fit for the estimated multiple regression equation increases,
(Multiple Choice)
4.8/5  (39)
(39)
In a multiple regression model, the variance of the error term is assumed to be
(Multiple Choice)
4.8/5  (35)
(35)
The following regression model has been proposed to predict sales at a fast food outlet.  = 18 - 2x1 + 7x2 + 15x3
where
x1 = the number of competitors within 1 mile
x2 = the population within 1 mile (in 1,000s)
x3 = 1 if drive-up windows are present, 0 otherwise
= 18 - 2x1 + 7x2 + 15x3
where
x1 = the number of competitors within 1 mile
x2 = the population within 1 mile (in 1,000s)
x3 = 1 if drive-up windows are present, 0 otherwise 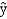 = sales (in $1,000s)
a.What is the interpretation of 15 (the coefficient of x3) in the regression equation?
b.Predict sales for a store with 2 competitors, a population of 10,000 within one mile, and one drive-up window (give the answer in dollars).
c.Predict sales for the store with 2 competitors, a population of 10,000 within one mile, and no drive-up window (give the answer in dollars).
= sales (in $1,000s)
a.What is the interpretation of 15 (the coefficient of x3) in the regression equation?
b.Predict sales for a store with 2 competitors, a population of 10,000 within one mile, and one drive-up window (give the answer in dollars).
c.Predict sales for the store with 2 competitors, a population of 10,000 within one mile, and no drive-up window (give the answer in dollars).
(Essay)
4.9/5  (37)
(37)
Exhibit 15-8
The following estimated regression model was developed relating yearly income (y in $1,000s) of 30 individuals with their age (x1) and their gender (x2) (0 if male and 1 if female). 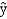 = 30 + 0.7x1 + 3x2
Also provided are SST = 1,200 and SSE = 384.
-The least squares criterion is
= 30 + 0.7x1 + 3x2
Also provided are SST = 1,200 and SSE = 384.
-The least squares criterion is
(Multiple Choice)
4.8/5  (37)
(37)
Exhibit 15-8
The following estimated regression model was developed relating yearly income (y in $1,000s) of 30 individuals with their age (x1) and their gender (x2) (0 if male and 1 if female). 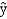 = 30 + 0.7x1 + 3x2
Also provided are SST = 1,200 and SSE = 384.
-The difference between the observed value of the dependent variable and the value predicted by using the estimated regression equation is the
= 30 + 0.7x1 + 3x2
Also provided are SST = 1,200 and SSE = 384.
-The difference between the observed value of the dependent variable and the value predicted by using the estimated regression equation is the
(Multiple Choice)
4.9/5  (32)
(32)
Exhibit 15-7
A regression model involving 4 independent variables and a sample of 15 periods resulted in the following sum of squares.SSR = 165
SSE = 60
-Refer to Exhibit 15-7. The test statistic from the information provided is
(Multiple Choice)
4.8/5  (35)
(35)
Exhibit 15-6
Below you are given a partial Excel output based on a sample of 16 observations. 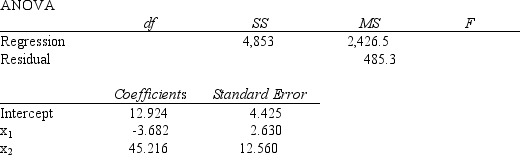 -A multiple regression model has the form
-A multiple regression model has the form 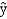 = 5 + 6x + 7w As x increases by 1 unit (holding w constant), y is expected to
= 5 + 6x + 7w As x increases by 1 unit (holding w constant), y is expected to
(Multiple Choice)
4.9/5  (43)
(43)
Exhibit 15-5
Below you are given a partial Excel output based on a sample of 25 observations.  -Refer to Exhibit 15-5. The interpretation of the coefficient on x1 is that
-Refer to Exhibit 15-5. The interpretation of the coefficient on x1 is that
(Multiple Choice)
4.8/5  (35)
(35)
Exhibit 15-2
A regression model between sales (y in $1,000), unit price (x1 in dollars) and television advertisement (x2 in dollars) resulted in the following function: 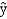 = 7 - 3x1 + 5x2
For this model SSR = 3500, SSE = 1500, and the sample size is 18.
-Refer to Exhibit 15-2. The coefficient of the unit price indicates that if the unit price is
= 7 - 3x1 + 5x2
For this model SSR = 3500, SSE = 1500, and the sample size is 18.
-Refer to Exhibit 15-2. The coefficient of the unit price indicates that if the unit price is
(Multiple Choice)
4.9/5  (39)
(39)
Showing 1 - 20 of 109
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)