Exam 16: Vector Calculus
Exam 1: Functions and Limits117 Questions
Exam 2: Derivatives151 Questions
Exam 3: Applications of Differentiation153 Questions
Exam 4: Integrals95 Questions
Exam 5: Applications of Integration120 Questions
Exam 6: Inverse Functions127 Questions
Exam 7: Techniques of Integration124 Questions
Exam 8: Further Applications of Integration86 Questions
Exam 9: Differential Equations67 Questions
Exam 10: Parametric Equations and Polar Coordinates72 Questions
Exam 11: Infinite Sequences and Series158 Questions
Exam 12: Vectors and the Geometry of Space60 Questions
Exam 13: Vector Functions93 Questions
Exam 14: Partial Derivatives132 Questions
Exam 15: Multiple Integrals124 Questions
Exam 16: Vector Calculus137 Questions
Exam 17: Second-Order Differential Equations63 Questions
Exam 18: Final Exam44 Questions
Select questions type
Let  where
where 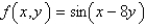 . Which of the following equations does the line segment from
. Which of the following equations does the line segment from 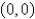 to
to 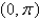 satisy?
satisy?
(Multiple Choice)
4.8/5  (38)
(38)
Evaluate the surface integral where S is the surface with parametric equations 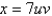 ,
,  .
. 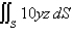
(Multiple Choice)
4.9/5  (33)
(33)
Use Stokes' Theorem to evaluate  .
. 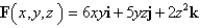 ; S is the part of the paraboloid
; S is the part of the paraboloid 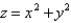 lying below the plane
lying below the plane 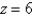 and oriented with normal pointing downward.
and oriented with normal pointing downward.
(Multiple Choice)
4.8/5  (24)
(24)
Find the mass of the surface S having the given mass density. S is part of the plane 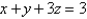 in the first octant; the density at a point P on S is equal to the square of the distance between P and the xy-plane.
in the first octant; the density at a point P on S is equal to the square of the distance between P and the xy-plane.
(Multiple Choice)
4.8/5  (37)
(37)
Find the area of the part of the cone  that is cut off by the cylinder
that is cut off by the cylinder 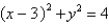
(Multiple Choice)
4.8/5  (32)
(32)
Use Stokes' Theorem to evaluate 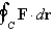 .
. 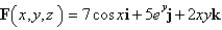 ; C is the curve obtained by intersecting the cylinder
; C is the curve obtained by intersecting the cylinder 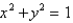 with the hyperbolic paraboloid
with the hyperbolic paraboloid 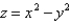 , oriented in a counterclockwise direction when viewed from above
, oriented in a counterclockwise direction when viewed from above
(Multiple Choice)
4.7/5  (33)
(33)
Set up, but do not evaluate, a double integral for the area of the surface with parametric equations 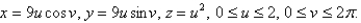
(Essay)
4.8/5  (37)
(37)
Find an equation of the tangent plane to the parametric surface represented by r at the specified point. 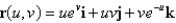 ; u = ln 9, v = 0
; u = ln 9, v = 0
(Essay)
4.7/5  (38)
(38)
Consider the vector field 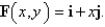 If a particle starts at the point
If a particle starts at the point 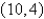 in the velocity field given by F, find an equation of the path it follows.
in the velocity field given by F, find an equation of the path it follows.
(Essay)
4.7/5  (34)
(34)
Use Green's Theorem to find the work done by the force 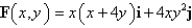 in moving a particle from the origin along the x-axis to (1, 0) then along the line segment to
(0, 1) and then back to the origin along the y-axis.
in moving a particle from the origin along the x-axis to (1, 0) then along the line segment to
(0, 1) and then back to the origin along the y-axis.
(Essay)
5.0/5  (38)
(38)
The plot of a vector field is shown below. A particle is moved 
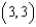

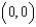 . By inspection, determine whether the work done by F on the particle is positive, negative, or zero.
. By inspection, determine whether the work done by F on the particle is positive, negative, or zero. 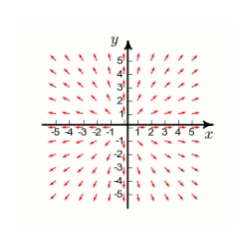
(Short Answer)
4.8/5  (44)
(44)
Evaluate the surface integral. 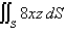 S is the part of the plane
S is the part of the plane 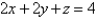 that lies in the first octant.
that lies in the first octant.
(Multiple Choice)
4.8/5  (22)
(22)
Below is given the plot of a vector field F in the xy-plane. (The z-component of F is 0.) By studying the plot, determine whether div F is positive, negative, or zero. 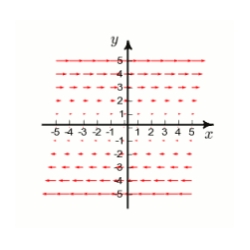
(Multiple Choice)
4.9/5  (30)
(30)
Show that F is conservative, and find a function f such that 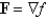 , and use the result to evaluate
, and use the result to evaluate 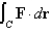 , where C is any curve from
, where C is any curve from 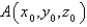 to
to 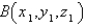 .
. 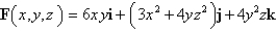 ;
; 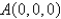 and
and 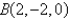
(Multiple Choice)
5.0/5  (37)
(37)
Showing 21 - 40 of 137
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)