Exam 6: Random Variables and Discrete Probability Distributions

The Sutton police department must write, on average, 6 tickets a day to keep department revenues at budgeted levels. Suppose the number of tickets written per day follows a Poisson distribution with a mean of 6.5 tickets per day. Interpret the value of the mean.

Number of Motorcycles: The probability distribution of a discrete random variable X is shown below, where X represents the number of motorcycles owned by a family. -Find the standard deviation of X.

The mean of a binomial distribution is equal to ____________________.

If n = 10 and p = 0.60, then the mean of the binomial distribution is

Find the mean and the standard deviation of Y = 2X - 1.

Classified Department Phone Calls: A classified department receives an average of 10 telephone calls each afternoon between 2 and 4 P.M. The calls occur randomly and independently of one another. -Find the probability that the department will receive 13 calls between 2 and 4 P.M. on a particular afternoon.

The dean of students conducted a survey on campus. Grade point average (GPA) is an example of a(n) ____________________ random variable.

Shopping Outlet: A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below. 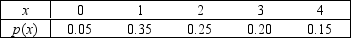-Suppose Y = 2X + 1 for each value of X. What is the probability distribution of Y?

A table, formula, or graph that shows all possible values a random variable can assume, together with their associated probabilities, is called a(n):

In the notation below, X is the random variable, E and V refer to the expected value and variance, respectively. Which of the following is false?

Find the probability that the number of arrivals between 4:00 and 5:00 P.M. is exactly two.

Sports Fans: Suppose that past history shows that 5% of college students are sports fans. A sample of 10 students is to be selected. -Find the probability that less than 1 student is a sports fan.

To find the probability that X is at least 10, you should find the probability that X is 10 or ____________________.

911 Phone Calls: 911 phone calls arrive at the rate of 30 per hour at the local call center. -If no calls are currently being processed, what is the probability that the desk employee can take four minutes break without being interrupted?

A table, formula, or graph that shows all possible values a random variable can assume, together with their associated probabilities, is referred to as discrete probability distribution.

Unsafe Levels of Radioactivity: The number of incidents at a nuclear power plant has a Poisson distribution with a mean of 6 incidents per year. -Find the probability that there will be no more than 1 incident in a year.

911 Phone Calls: 911 phone calls arrive at the rate of 30 per hour at the local call center. -Find the probability of receiving exactly eight calls in 15 minutes.

Online Bankers: An official from the securities commission estimates that 75% of all online bankers have profited from the use of insider information. Assume that 15 online bankers are selected at random from the commission's registry. -Find the probability that at least 6 have profited from insider information.

A motorcycle insurance company evaluates many numerical variables about a person before deciding on an appropriate rate for motorcycle insurance. How long a person has been a licensed rider is an example of a(n) ____________________ random variable.

A random variable is a function or rule that assigns a number to each outcome of an experiment.