Exam 6: Random Variables and Discrete Probability Distributions
Exam 1: What Is Statistics39 Questions
Exam 2: Graphical and Tabular Descriptive Techniques192 Questions
Exam 3: Numerical Descriptive Techniques215 Questions
Exam 4: Data Collection and Sampling82 Questions
Exam 5: Probability200 Questions
Exam 6: Random Variables and Discrete Probability Distributions158 Questions
Exam 7: Continuous Probability Distributions149 Questions
Exam 8: Sampling Distributions127 Questions
Exam 9: Introduction to Estimation85 Questions
Exam 10: Introduction to Hypothesis Testing178 Questions
Exam 11: Inference About a Population75 Questions
Exam 12: Inference About Comparing Two Populations, Part 183 Questions
Exam 13: Inference About Comparing Two Populations, Part 284 Questions
Exam 14: Analysis of Variance125 Questions
Exam 15: Chi-Squared Tests118 Questions
Exam 16: Simple Linear Regression and Correlation231 Questions
Exam 17: Multiple Regression143 Questions
Exam 18: Review of Statistical Inference182 Questions
Select questions type
The number of female customers out of a random sample of 100 customers arriving at a department store has a binomial distribution.
(True/False)
4.8/5  (31)
(31)
Find the probability that the number of arrivals between 3:30 and 4:00 P.M. is at least 10.
(Essay)
5.0/5  (32)
(32)
Retries: The following table contains the probability distribution for X = the number of retries necessary to successfully transmit a 1024K data package through a double satellite media.
 -What is the probability of a least one retry?
-What is the probability of a least one retry?
(Essay)
4.9/5  (38)
(38)
The binomial probability distribution is a discrete probability distribution.
(True/False)
5.0/5  (44)
(44)
The trials in a binomial experiment are ____________________, meaning the outcome of one trial does not affect the outcomes of any other trials.
(Short Answer)
4.8/5  (40)
(40)
Shopping Outlet: A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below.
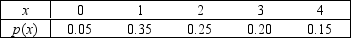 -Calculate the variance and standard deviation of Y directly from the probability distribution of Y.
-Calculate the variance and standard deviation of Y directly from the probability distribution of Y.
(Essay)
4.9/5  (35)
(35)
The number of home insurance policy holders is an example of a discrete random variable
(True/False)
4.7/5  (32)
(32)
The number of days that a microcomputer goes without a breakdown is an example of a(n) ____________________ random variable.
(Short Answer)
4.9/5  (31)
(31)
The number of accidents that occur annually on a busy stretch of highway is an example of:
(Multiple Choice)
4.9/5  (43)
(43)
The number of homeless people in Boston is an example of a discrete random variable.
(True/False)
4.8/5  (34)
(34)
Stress: Consider a binomial random variable X with n = 5 and p = 0. 40, where X represents the number of times in the final exam week a student with 18 credit hours may feel stressed.
-Find the expected number of times a student may feel stressed during the final exam week.
(Essay)
4.8/5  (23)
(23)
Sports Fans: Suppose that past history shows that 5% of college students are sports fans. A sample of 10 students is to be selected.
-Find the probability that at least 1 student is a sports fan.
(Essay)
4.9/5  (32)
(32)
Online Bankers: An official from the securities commission estimates that 75% of all online bankers have profited from the use of insider information. Assume that 15 online bankers are selected at random from the commission's registry.
-Find the variance and standard deviation of the number of Online bankers who have profited from the use of insider information.
(Essay)
4.7/5  (33)
(33)
A motorcycle insurance company evaluates many numerical variables about a person before deciding on an appropriate rate for motorcycle insurance. The distance a person rides in a year is an example of a(n) ____________________ random variable.
(Short Answer)
4.9/5  (43)
(43)
The mean of a Poisson distribution, where is the average number of successes occurring in a specified interval, is .
(True/False)
4.9/5  (35)
(35)
If X has a binomial distribution with n = 4 and p = 0.3, find P(X > 1).
(Essay)
4.9/5  (38)
(38)
If the probability of success p remains constant in a binomial distribution, an increase in n will not change the mean.
(True/False)
4.8/5  (40)
(40)
Stress: Consider a binomial random variable X with n = 5 and p = 0. 40, where X represents the number of times in the final exam week a student with 18 credit hours may feel stressed.
-Find P(2 X 4).
(Essay)
4.9/5  (35)
(35)
The variance of a binomial distribution for which n = 100 and p = 0.20 is:
(Multiple Choice)
4.9/5  (23)
(23)
Showing 81 - 100 of 158
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)