Exam 6: Random Variables and Discrete Probability Distributions

If X is a binomial random variable with n = 25, and p = 0.25, then P(X = 25) = 1.0.

The expected number of heads in 250 tosses of an unbiased coin is 125.

Stress: Consider a binomial random variable X with n = 5 and p = 0. 40, where X represents the number of times in the final exam week a student with 18 credit hours may feel stressed. -Find the variance and standard deviation.

Online Bankers: An official from the securities commission estimates that 75% of all online bankers have profited from the use of insider information. Assume that 15 online bankers are selected at random from the commission's registry. -Find the probability that all 15 have profited from insider information.

Sports Fans: Suppose that past history shows that 5% of college students are sports fans. A sample of 10 students is to be selected. -A sample of 100 students is to be selected. What is the average number that you would expect to sports fan?

The length of time for which an apartment in a large complex remains vacant is a discrete random variable.

A Poisson random variable is a(n) ____________________ random variable.

Which of the following is a continuous random variable?

Montana Highways: A recent survey in Montana revealed that 60% of the vehicles traveling on highways, where speed limits are posted at 70 miles per hour, were exceeding the limit. Suppose you randomly record the speeds of ten vehicles traveling on US 131 where the speed limit is 70 miles per hour. Let X denote the number of vehicles that were exceeding the limit. -Find the standard deviation of number of vehicles that are traveling on Montana highways and exceeding the speed limit.

In a Poisson experiment, the probability of a success in an interval is ____________________ to the size of the interval.

Sports Fans: Suppose that past history shows that 5% of college students are sports fans. A sample of 10 students is to be selected. -Find the probability that at most 1 student is a sports fan.

The binomial distribution deals with consecutive trials, each of which has two possible outcomes.

The mean of a discrete probability distribution for X is the sum of all possible values of X, divided by the number of possible values of X.

The weighted average of the possible values that a random variable X can assume, where the weights are the probabilities of occurrence of those values, is referred to as the:

The amount of milk consumed by a baby in a day is an example of a discrete random variable.

If X has a binomial distribution with n = 4 and p = 0.3, find P(X = 0).

Shopping Outlet: A shopping outlet estimates the probability distribution of the number of stores shoppers actually enter as shown in the table below. 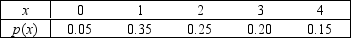-Use the laws of variance to calculate the variance and standard deviation of Y from the probability distribution of X.

911 Phone Calls: 911 phone calls arrive at the rate of 30 per hour at the local call center. -Find the probability of receiving two calls in a five-minute interval of time.

What is the probability that the student visits the gym at least once in a month?

A community college has 150 word processors. The probability that any one of them will require repair on a given day is 0.025. To find the probability that exactly 25 of the word processors will require repair, one will use what type of probability distribution?