Exam 12: B: linear Regression and Correlation

Wind Velocity and Windmills Narrative A scientist is studying the relationship between wind velocity (x in km/h) and DC output of a windmill (y). The following MINITAB output is from a regression analysis for predicting y from x. s = 0.2435 R-sq = 88.3% R-sq(adj) = 87.3% Analysis of Variance -Refer to Wind Velocity and Windmills Narrative. . What is the value of the error sum of squares?

Income and Education Narrative A professor of economics wants to study the relationship between income (y in $1,000s) and education (x in years). A random sample eight individuals is taken and the results are shown below. -Refer to Income and Education Narrative. Estimate with 95% confidence the average income of all individuals with ten years of education.

Sunshine and Skin Cancer Narrative A medical statistician wanted to examine the relationship between the amount of sunshine (x) in hours, and incidence of skin cancer (y). As an experiment, he found the number of skin cancers detected per 100,000 of population and the average daily sunshine in eight counties around the country. These data are shown below: -Refer to Sunshine and Skin Cancer Narrative. Draw a scatter diagram of the data and plot the least-squares regression line on it.

Antibiotic Potency Narrative An experiment was conducted to observe the effect of an increase in temperature on the potency of an antibiotic. Three 25 gram portions of the antibiotic were stored for equal lengths of time at each of these temperatures: 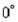C, C, 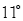C, and C. The potency readings observed at each temperature of the experimental period are listed here: 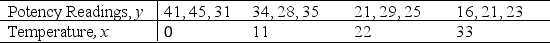-Refer to Antibiotic Potency Narrative. Use an appropriate statistical software program to construct the ANOVA table for linear regression.

Advertising and Money Spent Narrative A marketing analyst is studying the relationship between x = money spent on television advertising and y = increase in sales. One study reported the following data (in dollars) for a particular company. -Refer to Advertising and Money Spent Narrative. State and interpret the slope.

Antibiotic Potency Narrative An experiment was conducted to observe the effect of an increase in temperature on the potency of an antibiotic. Three 25 gram portions of the antibiotic were stored for equal lengths of time at each of these temperatures: 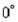C, C, 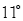C, and C. The potency readings observed at each temperature of the experimental period are listed here: 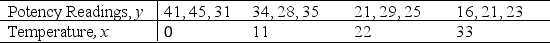-Refer to Antibiotic Potency Narrative. Do the data provide sufficient evidence to indicate that potency of an antibiotic is linearly related to the increase in temperature? Test at the 1% level of significance.

Sleep Deprivation Narrative A study was conducted to determine the effects of sleep deprivation on people's ability to solve s. The amount of sleep deprivation varied with 8, 12, 16, 20, and 24 hours without sleep. A total of ten subjects participated in the study, two at each sleep deprivation level. After his or her specified sleep deprivation period, each subject was administered a set of simple addition s, and the number of errors was recorded. These results were obtained: -Refer to Sleep Deprivation Narrative. Find a 95% confidence interval for the slope of the line.

Income and Height Narrative Do tall men earn more than short ones? An economist collected the data shown below for 25 men, where the annual income (y) in thousands of dollars and the height of the income earner (x) in cm. -Refer to Income and Height Narrative. Construct the ANOVA table for the linear regression.

Microwave Sales Narrative A microwave oven manufacturer has collected the data shown below on number of units sold (y) in the thousands of dollars and the number of ads (x) placed during the month. -Refer to Microwave Sales Narrative. Compute the standard error of the point estimate of number of units sold if there are 140 ads.

Income and Education Narrative A professor of economics wants to study the relationship between income (y in $1,000s) and education (x in years). A random sample eight individuals is taken and the results are shown below. -Refer to Income and Education Narrative. Interpret the value of the slope of the regression line.

Sales and Experience Narrative The general manager of a chain of furniture stores believes that experience is the most important factor in determining the level of success of a salesperson. To examine this belief, she records last month's sales (in $1000s) and the years of experience of ten randomly selected salespeople. These data are listed below. 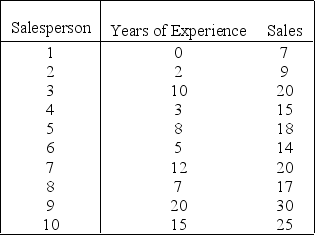-Refer to Sales and Experience Narrative. Predict with 95% confidence the monthly sales of a salesperson with ten years of experience.

Lumber Weight Narrative Let x be the weight in tonnes (1 tonne = 1000 kg) of a load of lumber and y be the time (in hours) it takes to load it onto a truck. A simple linear regression model relates x and y where the least-squares estimates of the regression parameters are b = 6.5 and a = 3.3. -Refer to Lumber Weight Narrative. What is the estimated time it takes to load 9 tonnes of lumber?

Income and Height Narrative Do tall men earn more than short ones? An economist collected the data shown below for 25 men, where the annual income (y) in thousands of dollars and the height of the income earner (x) in cm. -Refer to Income and Height Narrative. Compare the observed value of the F statistic with that of the t statistic. What is the relationship between the two values?

Vending Machines Narrative Let x be the number of vending machines and let y be the time (in hours) it takes to stock them. The data are as follows. -Refer to Vending Machines Narrative. Use a software package of your choice and report the regression analysis results.

Delivery Time Narrative Let x be the number of pieces of furniture in a delivery truck and y be the time (in hours) it takes the delivery person to deliver all the pieces of furniture. A simple linear regression analysis related x and y where the least-squares estimates of the regression parameters are a = 1.85 and b = 0.55. -Refer to Delivery Time Narrative. What is the least-squares best-fitting regression line?

SAT Scores and GPA Narrative A university admissions committee was interested in examining the relationship between a student's score on the Scholastic Aptitude Test, x, and the student's grade point average, y, at the end of the student's first year of university. The committee selected a random sample of 25 students and recorded the SAT score and GPA at the end of the first year of university for each student. Use the following output that was generated using statistical software to answer the questions below: Regression Analysis The regression equation is GPA = -1.09 + 0.00349 SAT S = 0.1463 R-Sq = 91.8% R-Sq(adj) = 91.5% Analysis of Variance 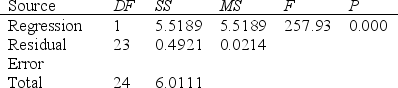Correlations (Pearson) Correlation of SAT and GPA = 0.958 -Refer to SAT Scores and GPA Narrative. Determine the coefficient of determination, and interpret its value.

Vending Machines Narrative Let x be the number of vending machines and let y be the time (in hours) it takes to stock them. The data are as follows. -Refer to Vending Machines Narrative. Estimate 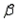using a 95% confidence interval.

Wind Velocity and Windmills Narrative A scientist is studying the relationship between wind velocity (x in km/h) and DC output of a windmill (y). The following MINITAB output is from a regression analysis for predicting y from x. s = 0.2435 R-sq = 88.3% R-sq(adj) = 87.3% Analysis of Variance -Refer to Wind Velocity and Windmills Narrative. Does a linear relationship exist between x and y? Test using = 0.05

Young Aspen Trees and Growth Narrative Let x be the number of leaves on a young aspen tree and let y be the growth of the tree (in mm). The data are as follows. 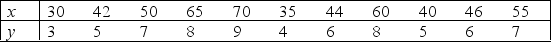-Refer to Young Aspen Trees and Growth Narrative. What is the least-squares regression line?

SAT Scores and GPA Narrative A university admissions committee was interested in examining the relationship between a student's score on the Scholastic Aptitude Test, x, and the student's grade point average, y, at the end of the student's first year of university. The committee selected a random sample of 25 students and recorded the SAT score and GPA at the end of the first year of university for each student. Use the following output that was generated using statistical software to answer the questions below: Regression Analysis The regression equation is GPA = -1.09 + 0.00349 SAT S = 0.1463 R-Sq = 91.8% R-Sq(adj) = 91.5% Analysis of Variance Correlations (Pearson) Correlation of SAT and GPA = 0.958 -Refer to SAT Scores and GPA Narrative. Use the p-value approach to test the usefulness of the linear regression model at the 0.05 level of significance.