Exam 9: Markov Chains and the Theory of Games
Exam 1: Straight Lines and Linear Functions268 Questions
Exam 2: Systems of Linear Equations and Matrices313 Questions
Exam 3: Linear Programming: a Geometric Approach214 Questions
Exam 4: Linear Programming: an Algebraic Approach115 Questions
Exam 5: Mathematics of Finance207 Questions
Exam 6: Sets and Counting196 Questions
Exam 7: Probability273 Questions
Exam 8: Probability Distributions and Statistics263 Questions
Exam 9: Markov Chains and the Theory of Games203 Questions
Select questions type
The transition matrix for a Markov process is given by State
1 2 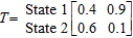 Given that the outcome state 2 has occurred, what is the probability that the next outcome of the experiment will be state 1?
Given that the outcome state 2 has occurred, what is the probability that the next outcome of the experiment will be state 1?
(Multiple Choice)
5.0/5  (32)
(32)
Consider the two-person, zero-sum matrix, strictly determined game. 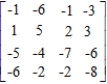
Find the value of the game.
Find the value of the game.
(Multiple Choice)
4.9/5  (31)
(31)
Find the steady-state vector for the transition matrix. 
(Multiple Choice)
4.8/5  (32)
(32)
Determine whether the given two-person, zero-sum matrix game is strictly determined.

(Essay)
4.7/5  (31)
(31)
Find X2 (the probability distribution of the system after two observations) for the distribution vector X0 and the transition matrix T.
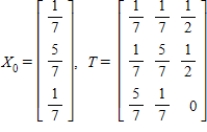
(Essay)
4.8/5  (32)
(32)
Find the expected payoff E of the game whose payoff matrix and strategies P and Q (for the row and column players, respectively) are given. 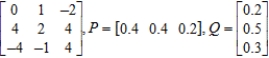
(Multiple Choice)
4.8/5  (28)
(28)
Find 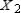 (the probability distribution of the system after two observations) for the given distribution vector
(the probability distribution of the system after two observations) for the given distribution vector 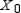 and the given transition matrix
and the given transition matrix  .
. 
(Multiple Choice)
4.9/5  (43)
(43)
Within a large metropolitan area, 20% of the commuters currently use the public transportation system, whereas the remaining 80% commute via automobile. The city has recently revitalized and expanded its public transportation system. It is expected that 6 months from now 40% of those who are now commuting to work via automobile will switch to public transportation, and 60% will continue to commute via automobile. At the same time, it is expected that 20% of those now using public transportation will commute via automobile and 80% will continue to use public transportation.
What percentage of the commuters are expected to use public transportation 6 months from now?
(Multiple Choice)
4.7/5  (34)
(34)
Consider the coin-matching game played by Richie and Chuck with the payoff matrix
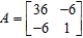
Find the optimal strategies for Richie and Chuck.
Find the optimal strategies for Richie and Chuck.

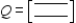
Find the value of the game.
Does it favor one player over the other?
Find the value of the game.
Does it favor one player over the other?
(Essay)
4.8/5  (36)
(36)
Diane has decided to play the following game of chance. She places a $1 bet on each repeated play of the game in which the probability of her winning $1 is 0.5. She has further decided to continue playing the game until she has either accumulated a total of $3 or has lost all her money.
What is the probability that Diane will eventually leave the game a winner if she started with a capital of $1?
What is the probability that Diane will eventually leave the game a winner if she started with a capital of $2?
Write your answer as a decimal rounded to two decimal places.
(Essay)
4.8/5  (35)
(35)
Find the steady-state vector for the transition matrix. 
(Multiple Choice)
4.9/5  (33)
(33)
Rewrite the absorbing stochastic matrix so that the absorbing states appear first, partition the resulting matrix, and identify the submatrices  and
and 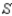 .
. 
(Multiple Choice)
4.8/5  (36)
(36)
Within a large metropolitan area, 15% of the commuters currently use the public transportation system, whereas the remaining 85% commute via automobile. The city has recently revitalized and expanded its public transportation system. It is expected that 6 months from now 10% of those who are now commuting to work via automobile will switch to public transportation, and 90% will continue to commute via automobile. At the same time, it is expected that 40% of those now using public transportation will commute via automobile, and 60% will continue to use public transportation. In the long run, what percent of the commuters will be using public transportation?
__________ %
(Short Answer)
4.7/5  (32)
(32)
Determine the maximin and minimax strategies for the two-person, zero-sum matrix game. 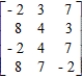
(Multiple Choice)
4.8/5  (37)
(37)
Consider the two-person, zero-sum matrix game. 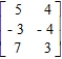
If the game is strictly determined, find the saddle point(s) of the game.
If the game is strictly determined, find the saddle point(s) of the game.
(Multiple Choice)
4.9/5  (29)
(29)
Consider the two-person, zero-sum matrix game. 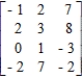
If the game is strictly determined, find the saddle point(s) of the game.
If the game is strictly determined, find the saddle point(s) of the game.
(Multiple Choice)
4.7/5  (35)
(35)
Showing 161 - 180 of 203
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)