Exam 23: Linear Programming Models
Exam 1: Operations and Productivity127 Questions
Exam 2: The Global Environment and Operations Strategy119 Questions
Exam 3: Managing Projects120 Questions
Exam 4: Forecasting Demand140 Questions
Exam 5: Product Design118 Questions
Exam 6: Quality Management and International Standards123 Questions
Exam 7: Process Design108 Questions
Exam 8: Location Decisions121 Questions
Exam 9: Layout Decisions146 Questions
Exam 10: Job Design and Work Measurement154 Questions
Exam 11: Supply Chain Management145 Questions
Exam 12: Managing Inventory163 Questions
Exam 13: Aggregate Scheduling116 Questions
Exam 14: Material Requirements Planning Mrpand Erp113 Questions
Exam 15: Scheduling for the Short-Term116 Questions
Exam 16: Jit,lean Operations,and the Toyota Production System115 Questions
Exam 17: Maintenance and Reliability Decisions111 Questions
Exam 18: Sustainability80 Questions
Exam 19: Statistical Process Control144 Questions
Exam 20: Capacity Planning96 Questions
Exam 21: Supply Chain Modeling55 Questions
Exam 22: Decision Modeling97 Questions
Exam 23: Linear Programming Models88 Questions
Exam 24: Transportation Modeling89 Questions
Exam 25: Queuing Models119 Questions
Exam 26: Learning Curves110 Questions
Exam 27: Modeling with Simulation75 Questions
Select questions type
A firm makes two products,Y and Z.Each unit of Y costs $10 and sells for $40.Each unit of Z costs $5 and sells for $25.If the firm's goal were to maximize profit,what would be the appropriate objective function?
(Multiple Choice)
4.8/5  (33)
(33)
A craftsman builds two kinds of birdhouses,one for wrens (X1),and one for bluebirds (X2).Each wren birdhouse takes four hours of labor and four units of lumber.Each bluebird house requires two hours of labor and twelve units of lumber.The craftsman has available 60 hours of labor and 120 units of lumber.Wren houses profit $6 each and bluebird houses profit $15 each.
Use the software output that follows to interpret the problem solution.Include a statement of the solution quantities (how many of which product),a statement of the maximum profit achieved by your product mix,and a statement of "resources unused" and "shadow prices." 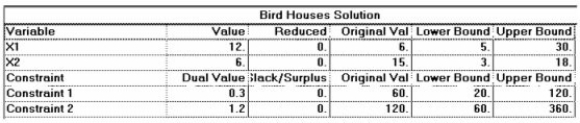
(Essay)
4.8/5  (40)
(40)
The objective of a linear programming problem is to maximize 1.50A + 1.50B,subject to 3A + 2B ≤ 600,2A + 4B ≤ 600,1A + 3B ≤ 420,and A,B ≥ 0.
a.Plot the constraints on the grid below
c.Identify the feasible region and its corner points.Show your work.
d.What is the optimal product mix for this problem? 
(Essay)
4.7/5  (42)
(42)
Identify three examples of resources that are typically constrained in a linear programming problem.
(Essay)
4.8/5  (36)
(36)
In terms of linear programming,the fact that the solution is infeasible implies that the "profit" can increase without limit.
(True/False)
4.8/5  (29)
(29)
Suppose that the feasible region of a maximization LP problem has corners of (0,0), (10,0), (5,5),and (0,7).If profit is given to be $X + $2Y what is the maximum profit the company can earn?
(Multiple Choice)
4.7/5  (40)
(40)
Rienzi Farms grows sugar cane and soybeans on its 500 acres of land.An acre of soybeans brings a $1000 contribution to overhead and profit;an acre of sugar cane has a contribution of $2000.Because of a government program no more than 200 acres may be planted in soybeans.During the planting season 1200 hours of planting time will be available.Each acre of soybeans requires 2 hours,while each acre of sugar cane requires 5 hours.The company seeks maximum contribution (profit)from its planting decision.
a.Formulate the problem as a linear program.
b.Solve using the corner-point method. 
(Essay)
4.7/5  (40)
(40)
The region that satisfies all of the constraints in linear programming is called the region of optimality.
(True/False)
4.9/5  (38)
(38)
Showing 81 - 88 of 88
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)