Exam 4: The Derivative in Graphing and Applications
Exam 1: Limits and Continuity186 Questions
Exam 2: The Derivative198 Questions
Exam 3: Topics in Deifferentiation171 Questions
Exam 4: The Derivative in Graphing and Applications656 Questions
Exam 5: Integration323 Questions
Exam 6: Applications of the Definite Integral in Geometry, Science and Engineering314 Questions
Exam 7: Principle of Integral Evaluation269 Questions
Exam 8: Mathematical Modeling With Differential Equations77 Questions
Exam 9: Infinte Series288 Questions
Exam 10: Parametric and Polar Curves; Conic Sections199 Questions
Exam 11: Three-Dimensional Space; Vectors173 Questions
Exam 12: Vector-Valued Functions147 Questions
Exam 13: Partial Derivatives194 Questions
Exam 14: Multiple Integrals117 Questions
Exam 15: Topics in Vector Calculus149 Questions
Select questions type
Answer true or false. f(x) = e6x has a horizontal asymptote at y = 0.
(True/False)
4.8/5  (29)
(29)
If f(x) = x4 - 12x2 , find the intervals where f is concave up and where f is concave down.
(Essay)
4.9/5  (41)
(41)
Verify that f(x) = x2 + 3x -1 satisfies the hypothesis of the Mean-Value Theorem over the interval [0, 1] and find all values of C that satisfy the conclusion of the theorem.
(Essay)
4.7/5  (39)
(39)
An open cylindrical trash can is to hold 19 cubic feet of material. What should be its dimensions if the cost of material used is to be a minimum? [Surface Area, S = r2 + 2 rh where r = radius and h = height.]
(Essay)
4.9/5  (41)
(41)
Given f(x) = cos2x + sin x . Use a graphing utility to estimate the absolute maximum and minimum values of f, if any, on the interval 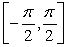 .
.
(Essay)
4.9/5  (39)
(39)
Answer true or false. A graphing utility can be used to show f(x) = |4x2| - 6 has two relative maxima on [-10, 10].
(True/False)
4.7/5  (28)
(28)
The position function of a particle is given by s = t3 - 6t2 - 8t for t 0. Find the functions for v and a.
(Short Answer)
4.9/5  (31)
(31)
Answer true or false. If f ''(-2) = -4 and f ''(2) = 4, then there must be an inflection point on (-2, 2).
(True/False)
4.8/5  (29)
(29)
Answer true or false. The triangle with the largest area that can be drawn inside a circle is an equilateral triangle.
(True/False)
4.9/5  (36)
(36)
Use a graphing utility to estimate the absolute minimum of f(x) = e x (ln x)6 on [1, 5].
(Short Answer)
4.9/5  (38)
(38)
Sketch a continuous curve having the following properties.
f(-5) = 10, f(0) = 5, f(5) = 0, f'(x) > 0 for |x| > 5,
f'(-5) = f'(5) = 0, f'(x) < 0 for x < 0, f'(x) > 0 for x > 0. 
(Essay)
4.9/5  (29)
(29)
Sketch a continuous curve having the following properties.
f(0) = 1, f(-1) = f(1) = 0; f '(x) > 0 for (- ,0) and f '(x) < 0 for (0, + ), f'(x) < 0 for (- , + ). 
(Essay)
4.7/5  (29)
(29)
Answer true or false. A graphing utility can be used to show f(x) = |3x2| - 12 has two relative maxima on [-20, 20].
(True/False)
4.9/5  (35)
(35)
Find the dimensions of the rectangular area of maximum area which can be laid out within a triangle of base 16 and altitude 4 if one side of the rectangle lies on the base of the triangle.
(Essay)
4.8/5  (36)
(36)
A rancher is going to build a 3-sided cattle enclosure with a divider down the middle as shown to the right. The cost per foot of the 3 side walls will be $4/foot, while the back wall, being taller, will be $8/foot. If the rancher wishes to enclose an area of 384 ft2, what dimensions of the enclosure will minimize his cost? 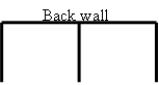
(Essay)
4.9/5  (28)
(28)
Answer true or false. The Mean-Value Theorem guarantees there is at least one c on [0, 1] such that f '(x) = 0.8 when f(x) = x.
(True/False)
4.9/5  (40)
(40)
Sketch the graph of 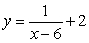 . Find any stationary points and any points of inflection. Also find any vertical and horizontal asymptotes.
. Find any stationary points and any points of inflection. Also find any vertical and horizontal asymptotes.
(Essay)
4.8/5  (29)
(29)
Showing 221 - 240 of 656
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)