Exam 4: The Derivative in Graphing and Applications
Exam 1: Limits and Continuity186 Questions
Exam 2: The Derivative198 Questions
Exam 3: Topics in Deifferentiation171 Questions
Exam 4: The Derivative in Graphing and Applications656 Questions
Exam 5: Integration323 Questions
Exam 6: Applications of the Definite Integral in Geometry, Science and Engineering314 Questions
Exam 7: Principle of Integral Evaluation269 Questions
Exam 8: Mathematical Modeling With Differential Equations77 Questions
Exam 9: Infinte Series288 Questions
Exam 10: Parametric and Polar Curves; Conic Sections199 Questions
Exam 11: Three-Dimensional Space; Vectors173 Questions
Exam 12: Vector-Valued Functions147 Questions
Exam 13: Partial Derivatives194 Questions
Exam 14: Multiple Integrals117 Questions
Exam 15: Topics in Vector Calculus149 Questions
Select questions type
Two fences, a distance of d = 16 feet apart are to be constructed so that the first fence is a height of h = 2 feet high and the second fence is higher than the first. What is the length of the shortest pole that has one end on the ground, passing over the first fence and reaches the second fence? See figure. 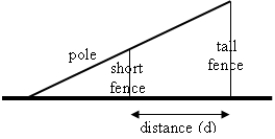
(Essay)
4.9/5  (34)
(34)
The function 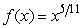 has a point of inflection with an x-coordinate of
has a point of inflection with an x-coordinate of
(Multiple Choice)
4.9/5  (33)
(33)
Sketch a continuous curve y = f(x) for x > 0 if 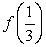 = 0, and f '(x) =
= 0, and f '(x) = 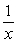 for all x > 0. Is the curve concave up or concave down?
for all x > 0. Is the curve concave up or concave down? 
(Essay)
4.8/5  (38)
(38)
Use Newton's Method to find the largest positive solution of x4 + 4x - 5 = 0. Use 4 for your initial value and calculate eight iterations.
(Multiple Choice)
4.7/5  (41)
(41)
Find the value for which f(x) = x3 -8 on [3, 7] satisfies the Mean-Value Theorem.
(Multiple Choice)
4.8/5  (31)
(31)
The equation, 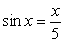 has one real solution for
has one real solution for 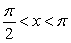 . Approximate it by Newton's Method.
. Approximate it by Newton's Method.
(Essay)
4.8/5  (37)
(37)
Does ![Does satisfy the hypothesis of the Mean-Value Theorem over the interval [0, 7]? If so, find all values of C that satisfy the conclusion of the theorem.](https://storage.examlex.com/TB6988/11ead0bc_8d34_d3b7_99a0_c3d12a3230ec_TB6988_11.jpg) satisfy the hypothesis of the Mean-Value Theorem over the interval [0, 7]? If so, find all values of C that satisfy the conclusion of the theorem.
satisfy the hypothesis of the Mean-Value Theorem over the interval [0, 7]? If so, find all values of C that satisfy the conclusion of the theorem.
(Essay)
4.7/5  (36)
(36)
Use a graphing utility to graph f(x) = 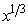 . How many points of inflection does the function have?
. How many points of inflection does the function have?
(Multiple Choice)
4.7/5  (42)
(42)
Let s(t) = sin 3t be a position function of a particle. At 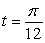 the particle's velocity is
the particle's velocity is
(Multiple Choice)
4.8/5  (35)
(35)
Answer true or false. An object that is thrown upward and reaches a height of s(t) = 100 + 160t - 16t2 for 0 t 3. The object is highest at t = 4.
(True/False)
4.8/5  (29)
(29)
Find the relative extreme values for ![Find the relative extreme values for on the interval [-10, 10] and determine where those values occur.](https://storage.examlex.com/TB6988/11ead0bc_8d25_1c45_99a0_cf2fd3f7c8b6_TB6988_11.jpg) on the interval [-10, 10] and determine where those values occur.
on the interval [-10, 10] and determine where those values occur.
(Essay)
4.8/5  (26)
(26)
The graph represents a position function. Determine what is happening to the velocity at t = 0. 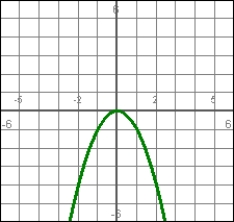
(Multiple Choice)
4.8/5  (34)
(34)
If three sides of a trapezoid are a = 8 inches long, how long should the fourth side be if the area is a maximum? [Area of a trapezoid = ![If three sides of a trapezoid are a = 8 inches long, how long should the fourth side be if the area is a maximum? [Area of a trapezoid = where a and b are the lengths of the parallel sides and h = height.]](https://storage.examlex.com/TB6988/11ead0bc_8d29_fe97_99a0_b5162cb85c6b_TB6988_11.jpg) where a and b are the lengths of the parallel sides and h = height.]
where a and b are the lengths of the parallel sides and h = height.] ![If three sides of a trapezoid are a = 8 inches long, how long should the fourth side be if the area is a maximum? [Area of a trapezoid = where a and b are the lengths of the parallel sides and h = height.]](https://storage.examlex.com/TB6988/11ead0bc_8d29_fe98_99a0_b5f350560d79_TB6988_00.jpg)
(Essay)
4.8/5  (40)
(40)
The position function of a particle is given by 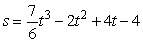 for t 0. Find the functions for v and a.
for t 0. Find the functions for v and a.
(Essay)
4.9/5  (45)
(45)
Answer true or false. If the graph on the left is a position function, the graph on the right represents the corresponding velocity function.
y = x2 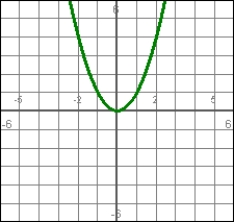
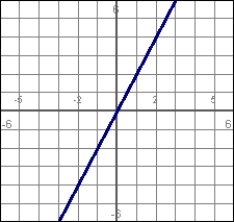
(True/False)
4.8/5  (38)
(38)
Showing 121 - 140 of 656
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)