Exam 18: Nonparametric Methods

Exhibit 19-1 Fifteen people were given two types of cereal, Brand X and Brand Y. Two people preferred Brand X and thirteen people preferred Brand Y. We want to determine whether or not customers prefer one brand over the other. -Refer to Exhibit 19-1. At 95% confidence, the null hypothesis should

Ten drivers were asked to drive two models of a car. Each car was given one gallon of gasoline. The distance that each automobile traveled on a gallon of gasoline is shown below. 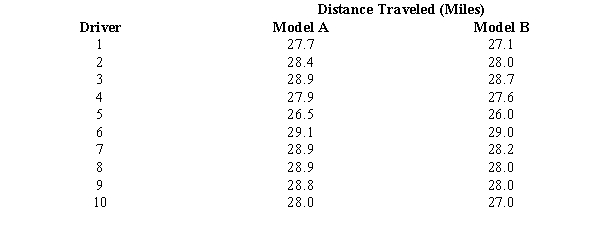At $\alpha$ = 0.05, what can be concluded about the performance of the two models?

The level of measurement that is simply a label for the purpose of identifying an item is

Exhibit 19-6 Forty-one individuals from a sample of 60 indicated they oppose legalized abortion. We are interested in determining whether or not there is a significant difference between the proportions of opponents and proponents of legalized abortion. -Refer to Exhibit 19-6. The null hypothesis should be

Exhibit 19-1 Fifteen people were given two types of cereal, Brand X and Brand Y. Two people preferred Brand X and thirteen people preferred Brand Y. We want to determine whether or not customers prefer one brand over the other. -Refer to Exhibit 19-1. The p-value for this test is

Exhibit 19-2 Students in statistics classes were asked whether they preferred a 10-minute break or to get out of class 10 minutes early. In a sample of 150 students, 40 preferred a 10-minute break, 80 preferred to get out 10 minutes early, and 30 had no preference. We want to determine if there is a difference in students' preferences. -Refer to Exhibit 19-2. The mean and the standard deviation of the sampling distribution of the number of students who preferred to get out early are

Exhibit 19-2 Students in statistics classes were asked whether they preferred a 10-minute break or to get out of class 10 minutes early. In a sample of 150 students, 40 preferred a 10-minute break, 80 preferred to get out 10 minutes early, and 30 had no preference. We want to determine if there is a difference in students' preferences. -Refer to Exhibit 19-2. The p-value for testing the hypotheses is

Exhibit 19-3 It is believed that the median yearly income in a suburb of Atlanta is $70,000. A sample of 67 residents was taken. Thirty-eight had yearly incomes above $70,000, 26 had yearly incomes below $70,000, and 3 had yearly incomes equal to $70,000. The null hypothesis to be tested is H₀: median = $70,000. -Refer to Exhibit 19-3. The null hypothesis should be

Independent random samples of ten day students and ten evening students at a university showed the following age distributions. We want to use the Mann-Whitney-Wilcoxon test to determine if there is a significant difference in the age distribution of the two groups. a.Compute the sum of the ranks (T) for the day students. b.Compute the mean $\mu$ _T. c.Compute $\sigma$ _T. d.. Use $\alpha$ = 0.05 and test for any significant differences in the age distribution of the two populations.

Exhibit 19-2 Students in statistics classes were asked whether they preferred a 10-minute break or to get out of class 10 minutes early. In a sample of 150 students, 40 preferred a 10-minute break, 80 preferred to get out 10 minutes early, and 30 had no preference. We want to determine if there is a difference in students' preferences. -Refer to Exhibit 19-2. The null hypothesis that is being tested is

Independent random samples of the scores of 10 daily quizzes from day students and 15 quizzes from evening students were gathered. It was decided to use the Mann-Whitney-Wilcoxon test to determine if there is a significant difference between the scores of the two groups of students. a.Provide the hypotheses for this test. b.Compute the mean $\mu$ _T. c.Compute the standard deviation $\sigma$ _T. d.The sum of the ranks for the day students was determined to be 184.5. Compute the test statistic Z. e.Use $\alpha$ = 0.05 and test to determine if there is a significant difference in the populations of the scores of the two groups.

Which of the following tests would not be an example of a nonparametric method?

Statistical methods that generally require very few, if any, assumptions about the population distribution are known as

Exhibit 19-3 It is believed that the median yearly income in a suburb of Atlanta is $70,000. A sample of 67 residents was taken. Thirty-eight had yearly incomes above $70,000, 26 had yearly incomes below $70,000, and 3 had yearly incomes equal to $70,000. The null hypothesis to be tested is H₀: median = $70,000. -Refer to Exhibit 19-3. The mean and the standard deviation (respectively) for this test about the median are

Exhibit 19-4 A company advertises that food preparation time can be significantly reduced with the Handy Dandy Slicer. A sample of 12 individuals prepared the ingredients for a meal with and without the slicer. You are given the preparation times below. -Refer to Exhibit 19-4. The null hypothesis should

The sales records of two branches of a department store over the last 12 months are shown below. (Sales figures are in thousands of dollars.) We want to use the Mann-Whitney-Wilcoxon test to determine if there is a significant difference in the sales of the two branches. 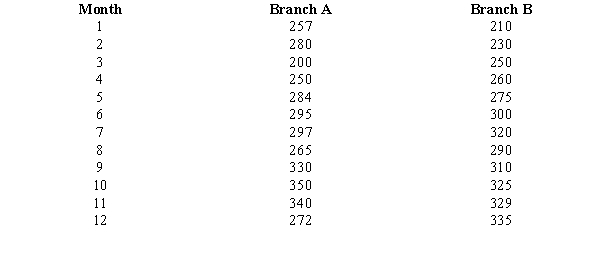a.Compute the sum of the ranks (T) for branch A. b.Compute the mean $\mu$ _T. c.Compute $\sigma$ _T. d.. Use $\alpha$ = 0.05 and test to determine if there is a significant difference in the populations of the sales of the two branches.

Exhibit 19-4 A company advertises that food preparation time can be significantly reduced with the Handy Dandy Slicer. A sample of 12 individuals prepared the ingredients for a meal with and without the slicer. You are given the preparation times below. -Refer to Exhibit 19-4. To test the null hypothesis, the appropriate probability distribution to use is

The Spearman rank-correlation coefficient for 20 pairs of data when $\Sigma$ d_i² = 50 is.

Two employers (A and B) ranked five candidates for a new position. Their rankings of the candidates are shown below. Compute the Spearman rank-correlation and test it for significance. Let $\alpha$ = 0.05.

Exhibit 19-4 A company advertises that food preparation time can be significantly reduced with the Handy Dandy Slicer. A sample of 12 individuals prepared the ingredients for a meal with and without the slicer. You are given the preparation times below. -Refer to Exhibit 19-4. The p-value for this test is