Question 1

If the coefficient of correlation is -0.7, then the percentage of the variation in y that is explained by the regression line is 70%.

Accepted Answer

A) True 
 B)False

Question 2

A financier whose specialty is investing in movie productions has observed that, in general, movies with 'big-name' stars seem to generate more revenue than those movies whose stars are less well known. To examine his belief, he records the gross revenue and the payment (in $ million) given to the two highest-paid performers in the movie for 10 recently released movies. $$\begin{array} { | c | c | c | } 
\hline \text { Movie } & \begin{array} { c } 
\text { Cost of two highest- } \
\text { paid performers } ( \$ \mathrm {~m} )
\end{array} & \begin{array} { c } 
\text { Gross revenue } \
( \$ \mathrm {~m} )
\end{array} \
\hline 1 & 5.3 & 48 \
\hline 2 & 7.2 & 65 \
\hline 3 & 1.3 & 18 \
\hline 4 & 1.8 & 20 \
\hline 5 & 3.5 & 31 \
\hline 6 & 2.6 & 26 \
\hline 7 & 8.0 & 73 \
\hline 8 & 2.4 & 23 \
\hline 9 & 4.5 & 39 \
\hline 10 & 6.7 & 58 \
\hline
\end{array}$$ Determine the coefficient of determination, and discuss what its value tells you about the two variables.

Accepted Answer

@#LAT-DLM& 0.9908, which means that 99.0

Question 3

The regression line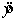  
 = 2 + 3x has been fitted to the data points (4,11), (2,7), and (1,5). The residual sum of squares will be 10.0.

Accepted Answer

A) True 
 B)False

Question 4

The manager of a fast food restaurant wants to determine how sales in a given week are related to the number of discount vouchers (#) printed in the local newspaper during the week. The number of vouchers and sales ($000s) from 10 randomly selected weeks is given below with the predicted sales. 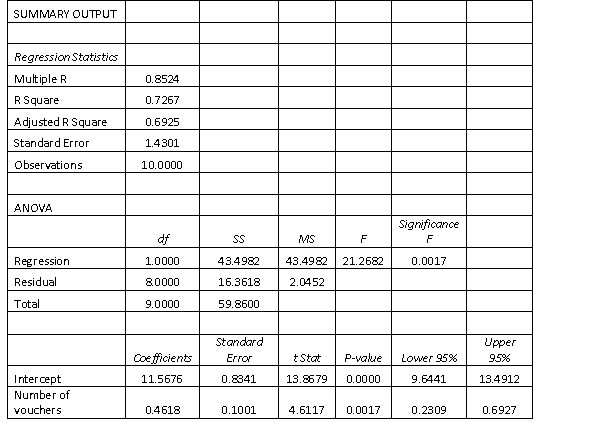  Compute the standardised residuals.

Accepted Answer

\[\begin{array} { | c | c | } 
\hline \t

Question 5

The vertical spread of the data points about the regression line is measured by the y-intercept.

Accepted Answer

A) True 
 B)False

Question 6

If the coefficient of correlation is −0.50, the percentage of the variation in the dependent variable y that is explained by the variation in the independent variable x is:

Accepted Answer

A)  − 50%. 
B)  25%. 
C)  50%. 
D)  − 25%. 
A)  − 50%. 
B)  25%. 
C)  50%. 
D)  − 25%.

Question 7

In a simple linear regression model, if r2 is 0.75, then 75% of the variation in the dependent variable y can be explained by the regression line, on the independent variable x.

Accepted Answer

A) True 
 B)False

Question 8

Which of the following statistics and procedures can be used to determine whether a linear model should be employed?

Accepted Answer

A)  The standard error of estimate. 
B)  The coefficient of determination. 
C)  The t-test of the slope. 
D)  All of these choices are correct. 
A)  The standard error of estimate. 
B)  The coefficient of determination. 
C)  The t-test of the slope. 
D)  All of these choices are correct.

Question 9

When the actual values y of a dependent variable and the corresponding predicted values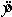  
 are the same, the standard error of the estimate will be 1.0.

Accepted Answer

A) True 
 B)False

Question 10

Given a specific value of x and confidence level, which of the following statements is correct?

Accepted Answer

A)  The confidence interval estimate of the expected value of y can be calculated but the prediction interval of y for the given value of x cannot be calculated. 
B)  The confidence interval estimate of the expected value of y will be wider than the prediction interval. 
C)  The prediction interval of y for the given value of x can be calculated but the confidence interval estimate of the expected value of y cannot be calculated. 
D)  The confidence interval estimate of the expected value of y will be narrower than the prediction interval. 
A)  The confidence interval estimate of the expected value of y can be calculated but the prediction interval of y for the given value of x cannot be calculated. 
B)  The confidence interval estimate of the expected value of y will be wider than the prediction interval. 
C)  The prediction interval of y for the given value of x can be calculated but the confidence interval estimate of the expected value of y cannot be calculated. 
D)  The confidence interval estimate of the expected value of y will be narrower than the prediction interval.

Question 11

On the least squares regression line Estimated y= 2 - 3x, the predicted value of y equals:

Accepted Answer

A)  -1.0 when x = -1.0. 
B)  1.0 when x = 1.0. 
C)  5.0 when x = -1.0. 
D)  5.0 when x = 1.0. 
A)  -1.0 when x = -1.0. 
B)  1.0 when x = 1.0. 
C)  5.0 when x = -1.0. 
D)  5.0 when x = 1.0.

Question 12

The quality of oil is measured in API gravity degrees - the higher the degrees API, the higher the quality. The table shown below is produced by an expert in the field, who believes that there is a relationship between quality and price per barrel. $$\begin{array} { | c | c | } 
\hline \text { Oil degrees API } & \text { Price per barrel (in } \$ \text { ) } \
\hline 27.0 & 12.02 \
\hline 28.5 & 12.04 \
\hline 30.8 & 12.32 \
\hline 31.3 & 12.27 \
\hline 31.9 & 12.49 \
\hline 34.5 & 12.70 \
\hline 34.0 & 12.80 \
\hline 34.7 & 13.00 \
\hline 37.0 & 13.00 \
\hline 41.0 & 13.17 \
\hline 41.0 & 13.19 \
\hline 38.8 & 13.22 \
\hline 39.3 & 13.27 \
\hline
\end{array}$$ A partial Minitab output follows.
Descriptive Statistics $$\begin{array} { | l | r | r | r | r | } 
\hline \text { Variable } & \mathrm { N } & \text { Mean } & \text { StDev } & \text { SE Mean } \
\hline \text { Degrees } & 13 & 34.60 & 4.613 & 1.280 \
\hline \text { Frice } & 13 & 12.730 & 0.457 & 0.127 \
\hline
\end{array}$$ Covariances $$\begin{array} { | l | r | r | } 
\hline & \text { Degrees } & \text { Price } \
\hline \text { Degrees } & 21.281667 & \
\hline \text { Price } & 2.026750 & 0.208833 \
\hline
\end{array}$$ Regression Analysis $$\begin{array} { | l | r | r | r | r | } 
\hline \text { Predictor } & \text { Coef } & \text { StDev } & \mathrm { T } & \mathrm { P } \
\hline \text { Constant } & 9.4349 & 0.2867 & 32.91 & 0.000 \
\hline \text { Degrees } & 0.095235 & 0.008220 & 11.59 & 0.000 \
\hline
\end{array}$$ S = 0.1314 R-Sq = 92.46% R-Sq(adj) = 91.7%
Analysis of Variance $$\begin{array} { | l | r | r | r | r | r | } 
\hline \text { Source } & \text { DF } & \text { SS } & \text { MS } & \text { F } & \text { P } \
\hline \text { Regression } & 1 & 2.3162 & 2.3162 & 134.24 & 0.000 \
\hline \text { Residual Error } & 11 & 0.1898 & 0.0173 & & \
\hline \text { Total } & 12 & 2.5060 & & & \
\hline
\end{array}$$ Conduct a test of the population coefficient of correlation to determine at the 5% significance level whether a linear relationship exists between the quality of oil and price per barrel.

Accepted Answer

@#LAT-DLM& . @#LAT-DLM& .
Rejection region: | t |

Question 13

The residuals are observations of the error variable $$\varepsilon$$ . Consequently, the minimised square of deviations is called the sum of squares for error, denoted SSE.

Accepted Answer

A) True 
 B)False

If the coefficient of correlation is -0.7, then the percentage of the variation in y that is explained by the regression line is 70%.

The regression line = 2 + 3x has been fitted to the data points (4,11), (2,7), and (1,5). The residual sum of squares will be 10.0.

The vertical spread of the data points about the regression line is measured by the y-intercept.

If the coefficient of correlation is −0.50, the percentage of the variation in the dependent variable y that is explained by the variation in the independent variable x is:

In a simple linear regression model, if r² is 0.75, then 75% of the variation in the dependent variable y can be explained by the regression line, on the independent variable x.

Which of the following statistics and procedures can be used to determine whether a linear model should be employed?

When the actual values y of a dependent variable and the corresponding predicted values are the same, the standard error of the estimate will be 1.0.

Given a specific value of x and confidence level, which of the following statements is correct?

On the least squares regression line Estimated y= 2 - 3x, the predicted value of y equals:

The residuals are observations of the error variable $\varepsilon$ . Consequently, the minimised square of deviations is called the sum of squares for error, denoted SSE.

What Is Statistics

Types of Data, Data Collection and Sampling

Graphical Descriptive Methods Nominal Data

Graphical Descriptive Techniques Numerical Data

Numerical Descriptive Measures

Probability

Random Variables and Discrete Probability Distributions

Continuous Probability Distributions

Statistical Inference: Introduction

Sampling Distributions

Estimation: Describing a Single Population

Estimation: Comparing Two Populations

Hypothesis Testing: Describing a Single Population

Hypothesis Testing: Comparing Two Populations

Inference About Population Variances

Analysis of Variance

Additional Tests for Nominal Data: Chi-Squared Tests

Multiple Regression

Model Building

Nonparametric Techniques

Statistical Inference: Conclusion

Time-Series Analysis and Forecasting

Index Numbers

Decision Analysis

Filters

Exam 18: Simple Linear Regression and Correlation

If the coefficient of correlation is -0.7, then the percentage of the variation in y that is explained by the regression line is 70%.

The regression line = 2 + 3x has been fitted to the data points (4,11), (2,7), and (1,5). The residual sum of squares will be 10.0.

The vertical spread of the data points about the regression line is measured by the y-intercept.

If the coefficient of correlation is −0.50, the percentage of the variation in the dependent variable y that is explained by the variation in the independent variable x is:

In a simple linear regression model, if r2 is 0.75, then 75% of the variation in the dependent variable y can be explained by the regression line, on the independent variable x.

Which of the following statistics and procedures can be used to determine whether a linear model should be employed?

When the actual values y of a dependent variable and the corresponding predicted values are the same, the standard error of the estimate will be 1.0.

Given a specific value of x and confidence level, which of the following statements is correct?

On the least squares regression line Estimated y= 2 - 3x, the predicted value of y equals:

The residuals are observations of the error variable ε\varepsilonε . Consequently, the minimised square of deviations is called the sum of squares for error, denoted SSE.

What Is Statistics

Types of Data, Data Collection and Sampling

Graphical Descriptive Methods Nominal Data

Graphical Descriptive Techniques Numerical Data

Numerical Descriptive Measures

Probability

Random Variables and Discrete Probability Distributions

Continuous Probability Distributions

Statistical Inference: Introduction

Sampling Distributions

Estimation: Describing a Single Population

Estimation: Comparing Two Populations

Hypothesis Testing: Describing a Single Population

Hypothesis Testing: Comparing Two Populations

Inference About Population Variances

Analysis of Variance

Additional Tests for Nominal Data: Chi-Squared Tests

Multiple Regression

Model Building

Nonparametric Techniques

Statistical Inference: Conclusion

Time-Series Analysis and Forecasting

Index Numbers

Decision Analysis

Filters

In a simple linear regression model, if r² is 0.75, then 75% of the variation in the dependent variable y can be explained by the regression line, on the independent variable x.

The residuals are observations of the error variable $\varepsilon$ . Consequently, the minimised square of deviations is called the sum of squares for error, denoted SSE.