Question 1

Determine the area under the z curve to the right of -1.82 ​

Accepted Answer

A)    
B)    
C)    
D)    
E)    
A)    
B)    
C)    
D)    
E)

Question 2

A box contains four slips of paper marked 1, 2, 3, and 4. Two slips are selected without replacement. List the possible values for the following random variable. x= number of slips marked "1" ​

Accepted Answer

A)  0,1 
B)  0,1,2 
C) 2 
D) 0 
E) 1 
A)  0,1 
B)  0,1,2 
C) 2 
D) 0 
E) 1

Question 3

The famous physicist, Ernest Rutherford, was a pioneer in the study of radioactivity using electricity. In one experiment he observed the number of particles reaching a counter during time 1700 intervals of 7.5 seconds each. The number of intervals that had 0 - 4 particles reaching the counter is given in the table below.   Let the random variable x = number of particles counted in a 7.5 second time period.   ​
Probability histogram   ​
b)Using the estimated probabilities in part (a), estimate the following:
i)P(x = 1), the probability that 1 particle was counted in 7.5 seconds.
ii)P(x < 3), the probability that fewer than 3 particles were counted.
iii)P(x ≥ 3), the probability that at least 3 particles were counted.

Accepted Answer

a)Probability Distribution:
Hi

Question 4

Ron rolls a six-sided dice until the number six is shown. What is the probability that he rolls a dice at least three times? What is the probability that he rolls a dice at most four times?

Accepted Answer

A) 0.3056; 0.5177. 
B) 0.3056; 0.4823. 
C) 0.6944; 0.5177. 
D) 0.6944; 0.4823. 
E) 0.5787; 0.4213. 
A) 0.3056; 0.5177. 
B) 0.3056; 0.4823. 
C) 0.6944; 0.5177. 
D) 0.6944; 0.4823. 
E) 0.5787; 0.4213.

Question 5

For a discrete random variable x,   .

Accepted Answer

A) True 
 B)False

Question 6

Suppose that the random variable 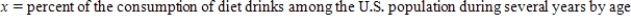  has the probability distribution described by the following density curve. ​   ​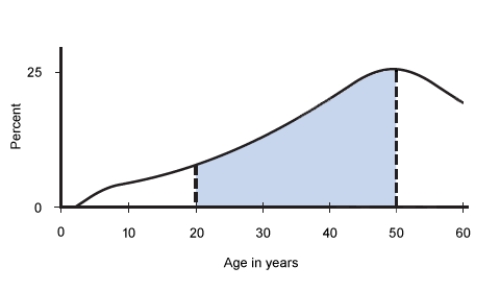
What probability is represented by the shaded area? Suppose the shaded area 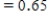  . Interpret this probability in the context of this problem.

Accepted Answer

A)    .About 65% of U.S.population consuming diet drinks are people aged less than 20 years. 
B)    .About 65% of U.S.population consuming diet drinks are people aged 50 years and over. 
C)    .About 65% of U.S.population consuming diet drinks are people aged between 20 and 50 years. 
D)    .About 0.65% of U.S.population consuming diet drinks are people aged between 20 and 50 years. 
E)    .About 35% of U.S.population consuming diet drinks are people aged between 20 and 50 years. 
A)    .About 65% of U.S.population consuming diet drinks are people aged less than 20 years. 
B)    .About 65% of U.S.population consuming diet drinks are people aged 50 years and over. 
C)    .About 65% of U.S.population consuming diet drinks are people aged between 20 and 50 years. 
D)    .About 0.65% of U.S.population consuming diet drinks are people aged between 20 and 50 years. 
E)    .About 35% of U.S.population consuming diet drinks are people aged between 20 and 50 years.

Question 7

Suppose that 73% of the visitors to a certain barbershop have a beard. Let the random variable x denote the number of coming visitors that have a beard until the visitor without beard comes. What is the probability that at most three visitors with beard must come? What is the probability that more than five visitors with beard must come?

Accepted Answer

A)    ;   
B)    ;   
C)    ;   
D)    ;   
E)    ;   
A)    ;   
B)    ;   
C)    ;   
D)    ;   
E)    ;

Question 8

Seventy-five percent of the computers sold by a certain store are laptops. Among 150 randomly selected computer purchases, what is the approximate probability that at most 125 are laptops? What is the approximate probability that between 100 and 120 (inclusive) are laptops? ​

Accepted Answer

A)    ;   . 
B)    ;   . 
C)    ;   . 
D)    ;   . 
E)    ;   . 
A)    ;   . 
B)    ;   . 
C)    ;   . 
D)    ;   . 
E)    ;   .

Question 9

Suppose that fund-raisers at a university call recent graduates to request donations for campus outreach programs. They report the following information for last year's graduates:   Consider the variable x = amount of donation for a person selected at random from the population of last year's graduates of this university.What is   ?

Accepted Answer

A) 0.10 
B) 0.50 
C) 0.55 
D) 0.75 
E) 0.90 
A) 0.10 
B) 0.50 
C) 0.55 
D) 0.75 
E) 0.90

Question 10

In a major study by the statistics classes at Washington High School, city parking spaces were examined for compliance with the requirement to put money in the parking meters. Overall, the students found that 76% of metered parking places had meters that had not expired, and 24% had meters that were expired. If the traffic officer in charge of ticketing cars with expired meters checks meters at random, what is the probability he or she will find an expired meter before the 3rd one?

Accepted Answer

P(expired before 3^rd)

Question 11

Suppose x is a continuous random variable with the probability distribution shouwn below. 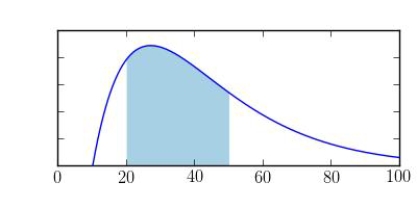  What probability is represented by the shaded area?

Accepted Answer

A)    
B)    
C)    
D)    
E) none of these 
A)    
B)    
C)    
D)    
E) none of these

Question 12

A normal probability plot suggests that a normal probability model is plausible if there is no obvious pattern in the scatter of points.

Accepted Answer

A) True 
 B)False

Question 13

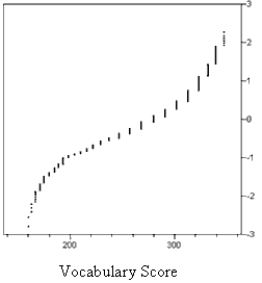
The graphs below are normal probability plots of the vocabulary and math concept scores on a standardized exam for a large sample of students. One of the score distributions is approximately normal. Identify the score distribution that is approximately normal and explain the reason for your choice.
​

Accepted Answer

The Math Concepts Score appears to be no

Question 14

The distribution of all values of a random variable is called a normal distribution.

Accepted Answer

A) True 
 B)False

Question 15

For a variable z that has a standard normal distribution,
a)What is the probability that z < −2.45?
b)What is the probability that z < +1.67?
c)What is the probability that z is between −1.43 and +1.43?
d)What value of z separates the smaller 10% of the standard normal distribution from the larger 90%?
e)What values of −z and +z separate the middle 95% of the standard normal distribution from the extreme 5%?

Accepted Answer

a)0.0071
b)0.9525
c)

Question 16

A business has five customer service telephone lines. Let x denote the number of lines in use at any given time. Suppose that the probability distribution of x is as follows:   Find the standard deviation of x. Round your answer to two decimal places.

Accepted Answer

A) 2.5 
B) 1.33 
C) 0.98 
D) 1.22 
E) 1.25 
A) 2.5 
B) 1.33 
C) 0.98 
D) 1.22 
E) 1.25

Question 17

Which of the following random variables are discrete? I). the volume of a balloon in liters
II)) the number of helium atoms in a balloon
II)) the number of balloons at a birthday party

Accepted Answer

A) I only 
B) II only 
C) III only 
D) II and III only 
E) I, II, and III 
A) I only 
B) II only 
C) III only 
D) II and III only 
E) I, II, and III

Question 18

Determine the following areas under the standard normal (z) curve.
a)The area under the z curve to the left of 1.56
b)The area under the z curve to the left of −2.13
c)The area under the z curve to the right of 0.88
d)The area under the z curve to the right of −1.23
e)The area under the z curve between −2 and 3
f)The area under the z curve between −2.4 and −1.4

Accepted Answer

a)0.9406
b)0.0166
c)

Question 19

Fifty-five percent of the students at Thomas Jefferson High School use "lunch cards" to charge their lunch, rather than bringing money to school. Assuming that the method of payment (cash or lunch card) for one student is independent of the method of payment for the next student in line, what is the probability of observing the following sequence for the first five students through the lunch line?
Cash, Card, Cash, Card, Cash

Accepted Answer

P(Cash, Card, Cash,

Question 20

In a certain very large city, the Department of Transportation (D.O.T.) has organized a complex system of bus transportation. In an advertising campaign, citizens are encouraged to use the new "GO-D.O.T!" system and head for the nearest bus stop to be transported to and from the central city. Suppose that at one of the bus stops the amount of time (in minutes) that a commuter must wait for a bus is a uniformly distributed random variable, T. The possible values of T run from 0 minutes to 20 minutes.
a)Sketch the probability distribution of T.
b)What is the probability that a randomly selected commuter will spend more than 7 minutes waiting for GO-D.O.T?

Accepted Answer

a)Density curve is a rectangle

Determine the area under the z curve to the right of -1.82

A box contains four slips of paper marked 1, 2, 3, and 4. Two slips are selected without replacement. List the possible values for the following random variable. x= number of slips marked "1"

Ron rolls a six-sided dice until the number six is shown. What is the probability that he rolls a dice at least three times? What is the probability that he rolls a dice at most four times?

For a discrete random variable x, .

Suppose that the random variable has the probability distribution described by the following density curve. What probability is represented by the shaded area? Suppose the shaded area . Interpret this probability in the context of this problem.

Seventy-five percent of the computers sold by a certain store are laptops. Among 150 randomly selected computer purchases, what is the approximate probability that at most 125 are laptops? What is the approximate probability that between 100 and 120 (inclusive) are laptops?

Suppose x is a continuous random variable with the probability distribution shouwn below. What probability is represented by the shaded area?

A normal probability plot suggests that a normal probability model is plausible if there is no obvious pattern in the scatter of points.

The graphs below are normal probability plots of the vocabulary and math concept scores on a standardized exam for a large sample of students. One of the score distributions is approximately normal. Identify the score distribution that is approximately normal and explain the reason for your choice.

The distribution of all values of a random variable is called a normal distribution.

A business has five customer service telephone lines. Let x denote the number of lines in use at any given time. Suppose that the probability distribution of x is as follows: Find the standard deviation of x. Round your answer to two decimal places.

Which of the following random variables are discrete? I). the volume of a balloon in liters II)) the number of helium atoms in a balloon II)) the number of balloons at a birthday party

Collecting Data in Reasonable Ways

Graphical Methods for Describing Data Distributions

Numerical Methods for Describing Data Distributions

Describing Bivariate Numerical Data

Probability

An Overview of Statistical Inference - Learning From Data

Sampling Variability and Sampling Distributions

Estimating a Population Proportion

Asking and Answering Questions About a Population Proportion

Asking and Answering Questions About the Difference Between Two Proportions

Asking and Answering Questions About a Population Mean

Asking and Answering Questions About the Difference Between Two Means

Learning From Categorical Data

Understanding Relationships - Numerical Data Part 2

Asking and Answering Questions About More Than Two Means

Filters

Exam 6: Random Variables and Probability Distributions

Determine the area under the z curve to the right of -1.82 ​

A box contains four slips of paper marked 1, 2, 3, and 4. Two slips are selected without replacement. List the possible values for the following random variable. x= number of slips marked "1" ​

Ron rolls a six-sided dice until the number six is shown. What is the probability that he rolls a dice at least three times? What is the probability that he rolls a dice at most four times?

For a discrete random variable x, .

Suppose that the random variable has the probability distribution described by the following density curve. ​ ​ What probability is represented by the shaded area? Suppose the shaded area . Interpret this probability in the context of this problem.

Seventy-five percent of the computers sold by a certain store are laptops. Among 150 randomly selected computer purchases, what is the approximate probability that at most 125 are laptops? What is the approximate probability that between 100 and 120 (inclusive) are laptops? ​

Suppose x is a continuous random variable with the probability distribution shouwn below. What probability is represented by the shaded area?

A normal probability plot suggests that a normal probability model is plausible if there is no obvious pattern in the scatter of points.

The distribution of all values of a random variable is called a normal distribution.

A business has five customer service telephone lines. Let x denote the number of lines in use at any given time. Suppose that the probability distribution of x is as follows: Find the standard deviation of x. Round your answer to two decimal places.

Which of the following random variables are discrete? I). the volume of a balloon in liters II)) the number of helium atoms in a balloon II)) the number of balloons at a birthday party

Collecting Data in Reasonable Ways

Graphical Methods for Describing Data Distributions

Numerical Methods for Describing Data Distributions

Describing Bivariate Numerical Data

Probability

An Overview of Statistical Inference - Learning From Data

Sampling Variability and Sampling Distributions

Estimating a Population Proportion

Asking and Answering Questions About a Population Proportion

Asking and Answering Questions About the Difference Between Two Proportions

Asking and Answering Questions About a Population Mean

Asking and Answering Questions About the Difference Between Two Means

Learning From Categorical Data

Understanding Relationships - Numerical Data Part 2

Asking and Answering Questions About More Than Two Means

Filters

Determine the area under the z curve to the right of -1.82

A box contains four slips of paper marked 1, 2, 3, and 4. Two slips are selected without replacement. List the possible values for the following random variable. x= number of slips marked "1"

Suppose that the random variable has the probability distribution described by the following density curve. What probability is represented by the shaded area? Suppose the shaded area . Interpret this probability in the context of this problem.

Seventy-five percent of the computers sold by a certain store are laptops. Among 150 randomly selected computer purchases, what is the approximate probability that at most 125 are laptops? What is the approximate probability that between 100 and 120 (inclusive) are laptops?