Exam 16: Polygons
Exam 1: Reasoning About Quantities34 Questions
Exam 2: Numeration Systems96 Questions
Exam 3: Understanding Whole Number Operations66 Questions
Exam 4: Some Conventional Ways of Computing17 Questions
Exam 5: Using Numbers in Sensible Ways38 Questions
Exam 6: Meanings for Fractions85 Questions
Exam 7: Computing With Fractions54 Questions
Exam 8: Multiplicative Comparisons and Multiplicative Reasoning19 Questions
Exam 9: Ratios, Rates, Proportions, and Percents33 Questions
Exam 10: Integers and Other Number Systems24 Questions
Exam 11: Number Theory57 Questions
Exam 12: What Is Algebra28 Questions
Exam 13: A Quantitative Approach to Algebra and Graphing18 Questions
Exam 14: Understanding Change: Relationships Among Time, Distance, and Rate10 Questions
Exam 15: Further Topics in Algebra and Change55 Questions
Exam 16: Polygons75 Questions
Exam 17: Polyhedra51 Questions
Exam 18: Symmetry17 Questions
Exam 19: Tessellations9 Questions
Exam 20: Similarity47 Questions
Exam 21: Curves, Constructions, and Curved Surfaces17 Questions
Exam 22: Transformation Geometry24 Questions
Exam 23: Measurement Basics21 Questions
Exam 24: Area, Surface Area, and Volume27 Questions
Exam 25: Counting Units Fast: Measurement Formulas31 Questions
Exam 26: Special Topics in Measurement21 Questions
Exam 27: Quantifying Uncertainty39 Questions
Exam 28: Determining More Complicated Probabilities37 Questions
Exam 29: Introduction to Statistics and Sampling7 Questions
Exam 30: Representing and Interpreting Data With One Variable32 Questions
Exam 31: Dealing With Multiple Data Sets or With Multiple Variables8 Questions
Exam 32: Variability in Samples21 Questions
Exam 33: Special Topics in Probability16 Questions
Select questions type
If possible, sketch an example of each description. If it is not possible, explain why. Use tick marks and hidden edges and label equal angles to make your intent clear.
A) a trapezoid with exactly two right angles
B) a kite with equal diagonals
(Essay)
4.8/5  (37)
(37)
Sketch, if possible, an obtuse isosceles triangle that has an angle with 30°. If such a triangle exists, give the measures of the other angles. If such a triangle is impossible, explain why.
(Short Answer)
4.8/5  (31)
(31)
Create a Venn diagram or a hierarchy diagram for only the following terms: quadrilateral, square, rectangle, polygon, and rhombi.
(Essay)
4.9/5  (43)
(43)
A) How many different arrangements of three identical rhombi are possible in which each rhombus matches up edge to edge with at least one other rhombus? Two arrangements are considered the same if one of the arrangements can be flipped and/or rotated to obtain the other arrangement. Sketch all possible arrangements.
B) Explain a counting strategy you used to justify that you have found all the different arrangements.
(Essay)
4.8/5  (28)
(28)
Tell whether each statement is always true, sometimes true, or never true. Justify your choice.
A) The diagonals of a parallelogram bisect each other.
B) The diagonals of a kite bisect each other.
(Essay)
4.9/5  (32)
(32)
Which shape will have ALL of the properties that every isosceles trapezoid has?
(Multiple Choice)
4.8/5  (28)
(28)
Sketch an example, if it is possible, of each shape described. If any are not possible tosketch, explain why.
A) an isosceles triangle that is not an acute triangle
B) a quadrilateral with two 90° angles that is not a rectangle (Be sure to mark the 90° angles.)
C) a kite that is also a rectangle
D) a triangular right prism (Show every hidden edge as a dashed segment.)
(Essay)
4.7/5  (28)
(28)
Arrange (only) the following terms in a hierarchical diagram, with the most general at the top: kite, square, polygon, trapezoid, and rectangle.
(Short Answer)
4.8/5  (31)
(31)
Which, if either, of the following is IMPOSSIBLE for x, y, and z in the sketch below? (The drawing is not to scale.)
I. x = 100, y = 110, z = 150
II. x = 80, y = 130, z = 150 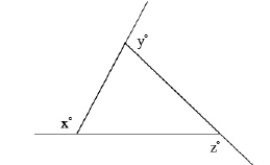
(Multiple Choice)
4.8/5  (38)
(38)
Which statement is TRUE for every rhombus?
I. The diagonals of a rhombus must be equal.
II. The sides of a rhombus must be equal.
(Multiple Choice)
4.9/5  (32)
(32)
For each shape, sketch an example if it is possible. If it is not possible, say so and explain why.
A) a trapezoid with exactly one right angle
B) a parallelogram that is an isosceles trapezoid
C) a pentagon with exactly one right angle
D) an isosceles obtuse triangle
E) a rhombus that is not a kite
(Essay)
4.8/5  (28)
(28)
Draw (if possible) an isosceles trapezoid with exactly one right angle. If it is not possible, explain why.
(Essay)
4.7/5  (34)
(34)
The sizes of three interior angles of a quadrilateral are 65°, 35°, and 60°. What is the size of the fourth angle of the quadrilateral?
(Multiple Choice)
4.7/5  (36)
(36)
The interior angles of a trapezoid add up to 360°, but only 270° if it is an isosceles trapezoid.
(True/False)
5.0/5  (38)
(38)
Showing 21 - 40 of 75
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)