Exam 7: Linear Programming
Exam 1: Algebra and Equations409 Questions
Exam 2: Graphs, Lines, and Inequalities255 Questions
Exam 3: Functions and Graphs323 Questions
Exam 4: Exponential and Logarithmic Functions192 Questions
Exam 5: Mathematics of Finance183 Questions
Exam 6: Systems of Linear Equations and Matrices215 Questions
Exam 7: Linear Programming203 Questions
Exam 8: Sets and Probability240 Questions
Exam 9: Counting, Probability Distributions, and Further Topics in Probability210 Questions
Exam 10: Introduction to Statistics169 Questions
Exam 11: Differential Calculus342 Questions
Exam 12: Applications of the Derivative220 Questions
Exam 13: Integral Calculus227 Questions
Exam 14: Multivariate Calculus152 Questions
Select questions type
A manufacturing company wants to maximize profits on products , and . The profit margin is for for , and for . The production requirements and departmental capacities are as follows:
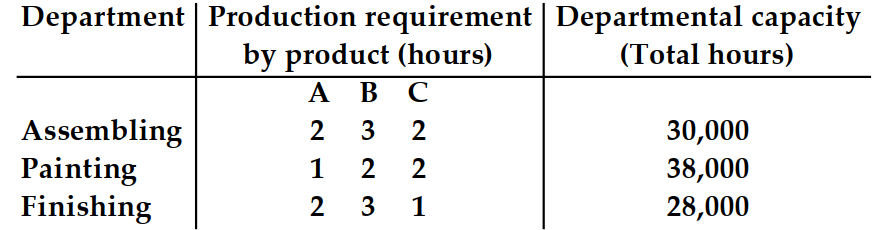 -What is the maximum profit in this model?
-What is the maximum profit in this model?
(Multiple Choice)
4.9/5  (39)
(39)
The Acme Class Ring Company designs and sells two types of rings: the VIP and the SST. They can produce up to 24rings each day using up to 60 total man hours of labor. It takes 3 man hours to make one VIP ring, versus 2 man hoursto make one SST ring.
-How many of each type of ring should be made daily to maximize the company's profit, if the profit on a VIP ring is and on an SST ring is ?
(Multiple Choice)
4.8/5  (38)
(38)
Provide an appropriate response.
-If a system has four inequalities, the number of corner points of the feasible region must be ?
(Multiple Choice)
4.9/5  (37)
(37)
A manufacturer of wooden chairs and tables must decide in advance how many of each item will be made in a givenweek. Use the table to find the system of inequalities that describes the manufacturer's weekly production.
-Use for the number of chairs and for the number of tables made per week. The number of work-hours available for construction and finishing is fixed.
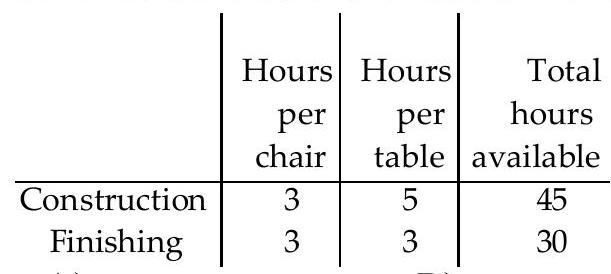
(Multiple Choice)
5.0/5  (40)
(40)
Use the two-stage method to solve.
-Find and such that
And is maximized.
(Multiple Choice)
4.8/5  (35)
(35)
The Acme Class Ring Company designs and sells two types of rings: the VIP and the SST. They can produce up to 24rings each day using up to 60 total man hours of labor. It takes 3 man hours to make one VIP ring, versus 2 man hoursto make one SST ring.
-How many of each type of ring should be made daily to maximize the company's profit, if the profit on a VIP ring is and on an SST ring is ?
(Multiple Choice)
4.7/5  (34)
(34)
The Acme Class Ring Company designs and sells two types of rings: the VIP and the SST. They can produce up to 24rings each day using up to 60 total man hours of labor. It takes 3 man hours to make one VIP ring, versus 2 man hoursto make one SST ring.
-How many of each type of ring should be made daily to maximize the company's profit, if the profit on a VIP ring is and on an SST ring is ?
(Multiple Choice)
4.8/5  (41)
(41)
Use the two-stage method to solve.
-Find and such that
+\leq50 +\geq25 2+\leq60
And is maximized.
(Multiple Choice)
4.8/5  (37)
(37)
Find the value(s) of the function on the given feasible region.
-Find the minimum of .
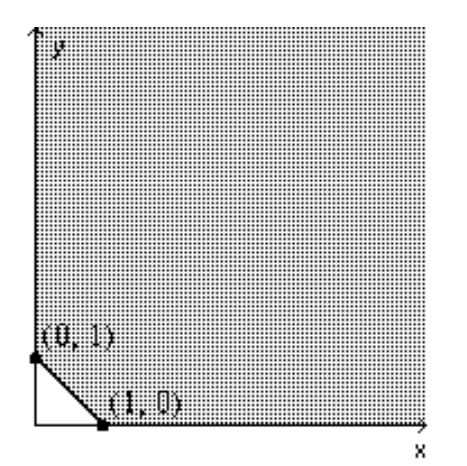
(Multiple Choice)
4.9/5  (30)
(30)
Provide an appropriate response.
-To determine the shading when graphing , the point would make a good test point. ?
(True/False)
4.9/5  (38)
(38)
Provide an appropriate response.
-Each solution of a simplex tableau corresponds to
(Short Answer)
4.9/5  (30)
(30)
Use graphical methods to solve the linear programming problem.
-Minimize
Subject to:

(Multiple Choice)
4.8/5  (40)
(40)
Solve the problem.
-An appliance store sells two types of refrigerators. Each Cool-It refrigerator sells for and each Polar sells for . Up to 260 refrigerators can be stored in the warehouse and new refrigerators are delivered only once a month. It is known that customers will buy at least 60 Cool-Its and at least 140 Polars each month. How many of each brand should the store stock and sell each month to maximize revenues?
(Multiple Choice)
4.8/5  (34)
(34)
A manufacturing company wants to maximize profits on products , and . The profit margin is for for , and for . The production requirements and departmental capacities are as follows:
 -What are the coefficients of the objective function?
-What are the coefficients of the objective function?
(Multiple Choice)
4.8/5  (45)
(45)
The Acme Class Ring Company designs and sells two types of rings: the VIP and the SST. They can produce up to 24rings each day using up to 60 total man hours of labor. It takes 3 man hours to make one VIP ring, versus 2 man hoursto make one SST ring.
-How many of each type of ring should be made daily to maximize the company's profit, if the profit on a VIP ring is and on an SST ring is ?
(Multiple Choice)
4.8/5  (41)
(41)
Showing 181 - 200 of 203
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)