Exam 7: Linear Programming
Exam 1: Algebra and Equations409 Questions
Exam 2: Graphs, Lines, and Inequalities255 Questions
Exam 3: Functions and Graphs323 Questions
Exam 4: Exponential and Logarithmic Functions192 Questions
Exam 5: Mathematics of Finance183 Questions
Exam 6: Systems of Linear Equations and Matrices215 Questions
Exam 7: Linear Programming203 Questions
Exam 8: Sets and Probability240 Questions
Exam 9: Counting, Probability Distributions, and Further Topics in Probability210 Questions
Exam 10: Introduction to Statistics169 Questions
Exam 11: Differential Calculus342 Questions
Exam 12: Applications of the Derivative220 Questions
Exam 13: Integral Calculus227 Questions
Exam 14: Multivariate Calculus152 Questions
Select questions type
A toy making company has at least 300 squares of felt, of stuffing, and of trim to make dogs and dinosaurs.
A dog uses 1 square of felt, of stuffing, and of trim. A dinosaur uses 2 squares of felt, of stuffing, and of trim.
-It costs the company to make each dog and for each dinosaur. The company wants to minimize its costs. What are the coefficients of the objective function?
(Multiple Choice)
4.8/5  (40)
(40)
The Acme Class Ring Company designs and sells two types of rings: the VIP and the SST. They can produce up to 24rings each day using up to 60 total man hours of labor. It takes 3 man hours to make one VIP ring, versus 2 man hoursto make one SST ring.
-How many of each type of ring should be made daily to maximize the company's profit, if the profit on a VIP ring is and on an SST ring is ?
(Multiple Choice)
5.0/5  (32)
(32)
A manufacturer of wooden chairs and tables must decide in advance how many of each item will be made in a givenweek. Use the table to find the system of inequalities that describes the manufacturer's weekly production.
-Use for the number of chairs and for the number of tables made per week. The number of work-hours available for construction and finishing is fixed.
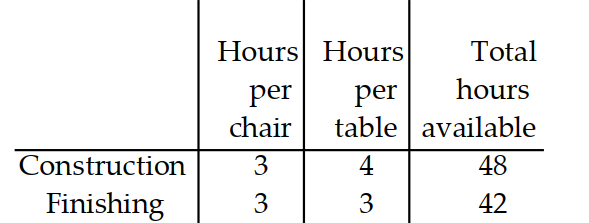
(Multiple Choice)
4.8/5  (42)
(42)
Solve the problem.
-Two foods are to be mixed. Food I contains 10 grams of carbohydrates per unit. Food II contains 5 grams of carbohydrates per unit. The total grams of carbohydrates in the mixture may not exceed 130 grams. No more than 18 units of Food I and no more than 6 units of Food II are to be used in the mixture. Each food contains 20 grams of protein per unit. How many units of each food should be used to maximize the grams of protein in the mixture?
(Multiple Choice)
4.8/5  (40)
(40)
Graph the feasible region for the system of inequalities.
-
(Multiple Choice)
4.8/5  (42)
(42)
State the linear programming problem in mathematical terms, identifying the objective function and the constraints.
-A car repair shop blends oil from two suppliers. Supplier I can supply at most 45 gal with detergent. Supplier II can supply at most with detergent. How much can be ordered from each to get at most 100 gal of oil with maximum detergent?
(Multiple Choice)
4.8/5  (44)
(44)
Use the simplex method to solve the linear programming problem.
-Maximize
Subject to:
With
(Multiple Choice)
4.8/5  (36)
(36)
Use the two-stage method to solve.
-Find and such that
And is maximized.
(Multiple Choice)
4.8/5  (34)
(34)
A manufacturer of wooden chairs and tables must decide in advance how many of each item will be made in a givenweek. Use the table to find the system of inequalities that describes the manufacturer's weekly production.
-Use -for the number of chairs and for the number of tables made per week. The number of work hours a yailable for construction and finishing is fixed.
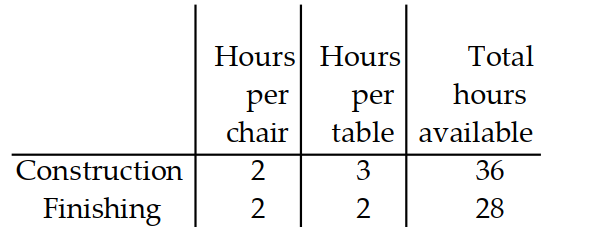
(Multiple Choice)
4.8/5  (31)
(31)
Use the simplex method to solve the linear programming problem.
-Maximize
Subject to:
With
(Multiple Choice)
4.9/5  (32)
(32)
Use the two-stage method to solve.
-Find and such that
+2\geq24 +\leq40
And is maximized.
(Multiple Choice)
4.8/5  (34)
(34)
Use the simplex method to solve the linear programming problem.
-Maximize
Subject to:
With
(Multiple Choice)
4.8/5  (38)
(38)
Use graphical methods to solve the linear programming problem.
-Maximize
Subject to:
(Multiple Choice)
4.9/5  (35)
(35)
Provide an appropriate response.
-What happens if an indicator other than the most negative one is chosen to solve a simplex tableau?
(Essay)
4.8/5  (37)
(37)
Provide an appropriate response.
-Is it possible to have a bounded feasible region that does not optimize an objective function?
(True/False)
4.7/5  (43)
(43)
Find the value(s) of the function, subject to the system of inequalities.
-Find the maximum and minimum of subject to:
, and .
(Multiple Choice)
4.8/5  (26)
(26)
Showing 101 - 120 of 203
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)