Exam 46: Linear Programming
Exam 1: Rectangular Coordinates69 Questions
Exam 2: Graphs of Equations63 Questions
Exam 3: Linear Equations in Two Variables61 Questions
Exam 4: Functions53 Questions
Exam 5: Analyzing Graphs of Functions56 Questions
Exam 6: A Library of Parent Functions50 Questions
Exam 7: Transformations of Functions32 Questions
Exam 8: Combinations of Functions Composite Functions58 Questions
Exam 9: Inverse Functions59 Questions
Exam 10: Mathematical Modeling and Variation49 Questions
Exam 11: Quadratic Functions and Models61 Questions
Exam 12: Polynomial Functions of Higher Degree63 Questions
Exam 13: Polynomial and Synthetic Division76 Questions
Exam 14: Complex Numbers59 Questions
Exam 15: Zeros of Polynomial Functions49 Questions
Exam 16: Rational Functions96 Questions
Exam 17: Nonlinear Inequalities56 Questions
Exam 18: Exponential Functions and Their Graphs59 Questions
Exam 19: Logarithmic Functions and Their Graphs64 Questions
Exam 20: Properties of Logarithms57 Questions
Exam 21: Exponential and Logarithmic Equations51 Questions
Exam 22: Exponential and Logarithmic Models56 Questions
Exam 23: Radian and Degree Measure52 Questions
Exam 24: Trigonometric Functions The Unit Circle50 Questions
Exam 25: Right Triangle Trigonometry56 Questions
Exam 26: Trigonometric Functions of Any Angle53 Questions
Exam 27: Graphs of Sine and Cosine Functions37 Questions
Exam 28: Graphs of Other Trigonometric Functions51 Questions
Exam 29: Inverse Trigonometric Functions50 Questions
Exam 30: Applications and Models52 Questions
Exam 31: Using Fundamental Identities60 Questions
Exam 32: Verifying Trigonometric Equations46 Questions
Exam 33: Solving Trigonometric Equations54 Questions
Exam 34: Sum and Difference Formulas62 Questions
Exam 35: Multiple Angle and Product to Sum Formulas50 Questions
Exam 36: Law of Sines43 Questions
Exam 37:Law of Cosines43 Questions
Exam 38:Vectors in the Plane50 Questions
Exam 39:Vectors and Dot Products67 Questions
Exam 40: Trigonometric Form of a Complex Number104 Questions
Exam 41: Linear and Nonlinear Systems of Equations58 Questions
Exam 42: Two Variable Linear Systems49 Questions
Exam 43: Multivariable Linear Systems54 Questions
Exam 44: Partial Fractions48 Questions
Exam 45: Systems of Inequalities50 Questions
Exam 46: Linear Programming50 Questions
Exam 47: Matrices and Systems of Equations65 Questions
Exam 48: Operations With Matrices59 Questions
Exam 49: The Inverse of a Square Matrix59 Questions
Exam 50: The Determinant of a Square Matrix52 Questions
Exam 51: Applications of Matrices and Determinants54 Questions
Exam 52: Sequences and Series68 Questions
Exam 53: Arithmetic Sequences and Partial Sums52 Questions
Exam 54: Geometric Sequences and Series67 Questions
Exam 55: Mathematical Induction48 Questions
Exam 56: The Binomial Theorem67 Questions
Exam 57: Counting Principles55 Questions
Exam 58: Probability47 Questions
Exam 59: Lines50 Questions
Exam 60: Introduction to Conics Parabolas124 Questions
Exam 61: Ellipses68 Questions
Exam 62: Hyperbolas62 Questions
Exam 63: Rotation of Conics52 Questions
Exam 64: Parametric Equations50 Questions
Exam 65: Polar Coordinates50 Questions
Exam 66: Polar Equations of Conics50 Questions
Exam 67: Graphs of Polar Equations49 Questions
Select questions type
Find the minimum value of the objective function and where it occurs,subject to the constraints: ?
Objective function:
?
Z = x + 4y
?
Constraints:
?
X ? 0
Y ? 0
X + 4y ? 20
X + y ? 18
?2x + 2y ? 21
(Multiple Choice)
4.8/5  (27)
(27)
Find the maximum value of the objective function and where it occurs,subject to the indicated constraints.
Objective function:
Z = 2x + 8y
Constraints:
X ≥ 0
Y ≥ 0
2x + y ≤ 12 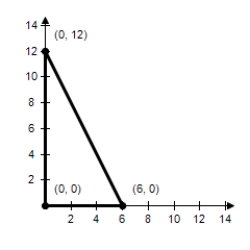
(Multiple Choice)
4.9/5  (33)
(33)
The linear programming problem has an unusual characteristic.Select a graph of the solution region for the problem and describe the unusual characteristic.Find the minimum value of the objective function (if possible)and where it occurs.
Objective function:
Z = x + y
Constraints:
X ≥ 0
Y ≥ 0
-x + y ≤ 1
-x + 5y ≤ 7
(Multiple Choice)
4.8/5  (36)
(36)
Find the maximum value of the objective function and where it occurs,subject to the indicated constraints.
Objective function:
Z = 4x + 3y
Constraints:
X ≥ 0
Y ≥ 0
X + y ≤ 4
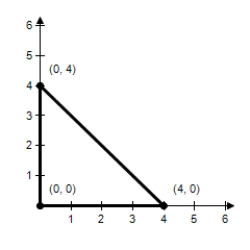
(Multiple Choice)
4.8/5  (31)
(31)
Select the region determined by the constraints.Then find the minimum value of the objective function (if possible)and where it occurs,subject to the indicated constraints.
Objective function:
Z = 7x + 6y
Constraints:
X ≥ 0
y ≥ 0
5x + 2y ≤ 20
5x + y ≥ 10
(Multiple Choice)
4.9/5  (34)
(34)
A merchant plans to sell two models of MP3 players at prices of $225 and $250.The $225 model yields a profit of $30 per unit and the $250 model yields a profit of $31 per unit.The merchant estimates that the total monthly demand will not exceed 280units.The merchant does not want to invest more than $55,776 in inventory for these products.What is the optimal inventory level for each model? What is the optimal profit?
(Multiple Choice)
4.9/5  (36)
(36)
Find the minimum and maximum values of the objective function and where it occurs,subject to the indicated constraints. Objective function:
Z = 3x + 4y
Constraints: ? 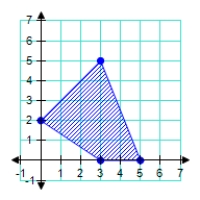
(Multiple Choice)
4.8/5  (27)
(27)
Find the maximum value of the objective function and where it occurs,subject to the constraints: ?
Objective function:
?
Z = 8x + 9y
?
Constraints:
?
X ? 0
Y ? 0
X + 4y ? 20
X + y ? 18
?2x + 2y ? 21
?
(Multiple Choice)
4.8/5  (36)
(36)
An animal shelter mixes two brands of dog food.Brand X costs $29 per bag and contains two units of nutritional element A,two units of element B,and two units of element C.Brand Y costs $22 per bag and contains one unit of nutritional element A,nine units of element B,and three units of element C.The minimum required amounts of nutrients A,B,and C are 12 units,36 units,and 24 units,respectively.What is the optimal number of bags of each brand that should be mixed? What is the optimal cost?
(Multiple Choice)
4.8/5  (36)
(36)
Find the maximum value of the objective function and where it occurs,subject to the indicated constraints.(You should graph the feasible solutions on the grid below before you attempt to find the minimum and maximum values. ) Objective function:
Z = 6x - 7y
Constraints: 
(Multiple Choice)
4.8/5  (29)
(29)
Find the maximum value of the objective function and where it occurs,subject to the constraints: ?
Objective function:
?
Z = 12x + 24y
?
Constraints:
?
X ? 0
Y ? 0
X + 4y ? 20
X + y ? 18
?2x + 2y ? 21
?
(Multiple Choice)
4.9/5  (46)
(46)
Select the region determined by the constraints.Then find the minimum value of the objective function (if possible)and where it occurs,subject to the indicated constraints.
Objective function:
Z = 9x + 8y
Constraints:
X ≥ 0
Y ≥ 0
2x + 2y ≥ 10
X + 2y ≥ 6
(Multiple Choice)
4.9/5  (43)
(43)
Find the maximum value of the objective function and where it occurs,subject to the constraints: ?
Objective function:
?
Z = 5x + y
?
Constraints:
?
X ? 0
Y ? 0
X + 4y ? 20
X + y ? 18
?2x + 2y ? 21
?
(Multiple Choice)
4.8/5  (32)
(32)
The linear programming problem has an unusual characteristic.Select a graph of the solution region for the problem and describe the unusual characteristic.Find the minimum value of the objective function (if possible)and where it occurs.
Z = x + 3y
Constraints:
X ≥ 0
Y ≥ 0
X + 2y ≤ 4
2x + y ≤ 4
(Multiple Choice)
4.8/5  (32)
(32)
The linear programming problem has an unusual characteristic.Select a graph of the solution region for the problem and describe the unusual characteristic.Find the minimum value of the objective function (if possible)and where it occurs. ?
Objective function:
?
Z = 2.5x + y
?
Constraints:
?
X ? 0
Y ? 0
3x + 5y ? 15
?5x + 2y ? 10
?
(Multiple Choice)
4.8/5  (37)
(37)
A humanitarian agency can use two models of vehicles for a refugee rescue mission.Each model A vehicle costs $1000 and each model B vehicle costs $1500.Mission strategies and objectives indicate the following constraints.A total of at least 20 vehicles must be used.A model A vehicle can hold 45 boxes of supplies.A model B vehicle can hold 24 boxes of supplies.The agency must deliver at least 690 boxes of supplies to the refugee camp.A model A vehicle can hold 17 refugees.A model B vehicle can hold 35 refugees.The agency must rescue at least 520 refugees.What is the optimal number of vehicles of each model that should be used? What is the optimal cost?
(Multiple Choice)
4.7/5  (35)
(35)
Find the minimum value of the objective function and where it occurs,subject to the indicated constraints.
Objective function:
Z = 4x + 16y
Constraints:
X ≥ 0
Y ≥ 0
2x + y ≤ 12 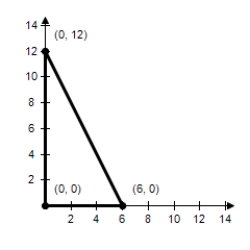
(Multiple Choice)
4.9/5  (39)
(39)
Find the minimum value of the objective function and where it occurs,subject to the constraints: ?
Objective function:
?
Z = 5x + 6y
?
Constraints:
?
X ? 0
Y ? 0
X + 4y ? 20
X + y ? 18
?2x + 2y ? 21
?
(Multiple Choice)
4.8/5  (29)
(29)
Find the maximum value of the objective function and where it occurs,subject to the constraints:
Objective function:
Z = 7x + y
Constraints:
X ≥ 0
Y ≥ 0
3x + y ≤ 15
4x + 3y ≤ 30
(Multiple Choice)
4.9/5  (33)
(33)
Find the minimum value of the objective function and where it occurs,subject to the constraints: ?
Objective function:
?
Z = 4x + 16y
?
Constraints:
?
X ? 0
Y ? 0
X + 4y ? 20
X + y ? 18
?2x + 2y ? 21
?
(Multiple Choice)
4.7/5  (30)
(30)
Showing 21 - 40 of 50
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)