Exam 6: Applications of Integration
Exam 1: Functions and Models118 Questions
Exam 2: Limits and Derivatives127 Questions
Exam 3: Differentiation Rules248 Questions
Exam 4: Applications of Differentiation273 Questions
Exam 5: Integrals239 Questions
Exam 6: Applications of Integration189 Questions
Exam 7: Differential Equations154 Questions
Exam 8: Infinite Sequences and Series341 Questions
Exam 9: Vectors and the Geometry of Space269 Questions
Exam 10: Vector Functions111 Questions
Exam 11: Partial Derivatives294 Questions
Exam 12: Multiple Integrals270 Questions
Exam 13: Vector Calculus240 Questions
Select questions type
Suppose a company has estimated that the marginal cost of manufacturing x items is (measured in dollars per unit) with a fixed start-up cost of c(0) = 10,000. Find the cost of producing the first 500 items.
Free
(Multiple Choice)
4.8/5  (28)
(28)
Correct Answer:
A
Find the volume of the solid obtained by rotating the region bounded by the curves about the x-axis.
Free
(Short Answer)
4.8/5  (38)
(38)
Correct Answer:
Find the area of the region bounded by the curves and
Free
(Multiple Choice)
4.8/5  (30)
(30)
Correct Answer:
E
A curve is written parametrically as Find the arc length of the curve from .
(Short Answer)
4.8/5  (33)
(33)
The base of a certain solid is an elliptical region with boundary curve Cross-sections perpendicular to the x-axis are squares. Find the volume of the solid.
(Short Answer)
4.8/5  (39)
(39)
A solid has a circular base of radius 1. Parallel cross-sections perpendicular to the base are equilateral triangles. Find the volume of the solid.
(Multiple Choice)
4.8/5  (38)
(38)
The tank in the figure below is full of water with a density 62.5 lb/ft3. 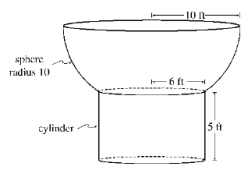 How much work is required to empty the tank by pumping water to a point 4 feet above the top of the tank?
How much work is required to empty the tank by pumping water to a point 4 feet above the top of the tank?
(Essay)
4.9/5  (36)
(36)
A force of 8 dynes is required to stretch a spring from its natural length of 10 cm to a length of 15 cm. How much work is done
(a) in stretching the spring to a length of 25 cm?
(b) in stretching the spring from a length of 20 cm to a length of 25 cm?
(Short Answer)
4.9/5  (34)
(34)
Find the volume of the solid obtained when the region bounded by the curves is rotated about the line x =1.
(Multiple Choice)
4.8/5  (33)
(33)
Find the volume of the solid obtained by rotating the region bounded by and x = 1 about the line x = 1. (Use a graphing calculator.)
(Essay)
4.8/5  (31)
(31)
A swimming pool 24 feet long and 15 feet wide has a bottom that is an inclined plane, the shallow end having a depth of 3 feet, and the deep end 10 feet. The pool is filled with water. For simplicity, take the density of water to be 60 lb/ft3. Find the hydrostatic force in pounds on the bottom of the pool.
(Multiple Choice)
4.7/5  (35)
(35)
Find the average value of the function whose graph is given below. 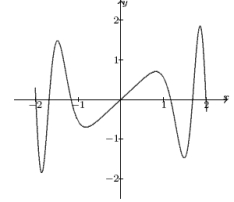
(Short Answer)
5.0/5  (36)
(36)
Find the volume of the solid obtained by rotating the region bounded by the curves about the line x = 2.
(Short Answer)
4.8/5  (35)
(35)
Let R be region bounded by the curve .(a) Find the volume of the solid obtained by rotating R about the x-axis.(b) Find the volume of the solid obtained by rotating R about the line x = 5.(c) Find the volume of the solid obtained by rotating R about the line y = -1.
(Essay)
5.0/5  (39)
(39)
A tank contains water. The end of the tank is vertical and has the shape below. Find the hydrostatic force against the end of the tank. 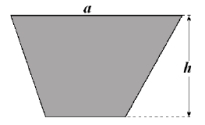
(Essay)
4.7/5  (30)
(30)
The marginal revenue for a company when sales are q units is given by . Find the increase in revenue when the sales level increases from 100 to 200 units.
(Short Answer)
4.8/5  (32)
(32)
Find the volume of the solid obtained by rotating the region bounded by the y-axis and the x-axis about the y-axis.
(Essay)
4.9/5  (37)
(37)
Showing 1 - 20 of 189
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)