Exam 1: Functions and Models

The following table contains United States population data for the years 1981-1990, as well as estimates based on the 1990 census. Year U.S. Population (millions) 1981 229.5 1982 231.6 1983 233.8 1984 235.8 1985 237.9 1986 240.1 1987 242.3 1988 244.4 1989 246.8 1990 249.5 Year U.S. Population (millions) 1991 252.2 1992 255.0 1993 257.8 1994 260.3 1995 262.8 1996 265.2 1997 267.8 1998 270.2 1999 272.7 2000 275.1 Source: U.S. Census Bureau website (a) Make a scatter plot for the data and use your scatter plot to determine a mathematical model of the U.S. population.(b) Use your model to predict the U.S. population in 2003.

Find the smallest value in the range of the function f (x) = $| 2 x | + | 2 x + 3 |$ .

Sketch a possible parametric curve defined by the following table of values. t 0 \pi 2\pi x 2 0 - -2 - 0 2 y 0 -2 0 - 2 - 0

Eliminate the parameter in the equations $2 = \tan t , y = 2 \cos ^ { 2 } t , 0 \leq t \leq 2 \pi$

A baseball slugger hits a knee-high pitch toward the outfield. Suppose that the position of the baseball after t seconds is given by : x = (v $o$ cos $\alpha$ ) t, y = (v $o$ sin $\alpha$ ) t $- 16 t ^ { 2 } + h _ { o }$ where v $o$ is the velocity in feet per second at which the ball leaves the bat at an angle $\alpha$ to the horizontal and from a height h $o$ above the ground.(a) Suppose that the ball is struck 2 feet above the ground with an initial velocity of 120 ft/sec and at an angle of 35 degrees.(i) When will the ball strike the ground? (ii) How far will the ball travel (horizontally) before it touches the ground? (iii) What is the maximum height reached by the ball? (iv) Will the ball clear the 10 ft tall outfield fence, which is 410 feet away from the point where the ball is struck? (b) If the ball is struck 2 feet above the ground at an initial velocity of 120 ft/sec and at an angle of 55 degrees: (i) When will the ball strike the ground? (ii) How far will the ball travel (horizontally) before it touches the ground? (iii) What is the maximum height reached by the ball? (iv) Will the ball clear the 10' tall outfield fence, which is 410 feet away from the point where the ball is struck?

Make a rough sketch of the graph of y = 3 $1 - x$ . Do not use a calculator.

Let f(x) = $\frac { 1 } { 2 }$ x and $( f \circ g ) ( x ) = x ^ { 2 }$ . Find g(2).

Given the graph of y = f (x): 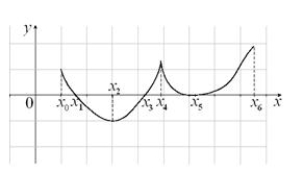(a) f is increasing.(b) f is decreasing.

An ice cream vendor is stopped on the side of a city street 100 feet from a perpendicular intersection of the street with another straight city street. A bicyclist is riding on the perpendicular street at a rate of 1320 feet/second. If the bicyclist continues to ride straight ahead at the same rate of speed, write a function for the distance, d, between the ice cream vendor and the bicyclist for time t beginning when the bicyclist is in the intersection.

Evaluate the difference quotient $\frac { f ( x ) - f ( a ) } { x - a }$ for f(x) = $\frac { 1 } { x ^ { 2 } }$ .

Relative to the graph of y = x $2$ + 2, the graph of y = (x - 2) $2$ $+$ 2 is changed in what way?

Let f (x) = 8 + x $2$ . Find each of the following: (a) f (2) $+$ f ( $- 2$ ) (b) f (x $+$ 2) (c) [f (x)] $2$ (d) f (x $2$ )

Describe the motion of a particle with position x = 2 + cos t, y = 3 + sin t as t varies in the interval [0, 2 $\pi$ ].

Let h(x) = sin $2$ x $+$ 3 sin x - 4 and g(x) = sin x. Find f(x) so that h(x) = $( f \circ g ) ( x )$

Determine an appropriate viewing rectangle for the graph of the function f (x) = $\frac { 3 x } { x ^ { 4 } + 75 }$ ..

Make a rough sketch of the graph of y = -2 $| x |$ . Do not use a calculator.

Sketch the graph of f for f (x) = $\sqrt [ 3 ] { x }$ and determine if f $- 1$ exists . If so, find a formula for f $- 1$ (x) and sketch its graph.

Find the value of $e ^ { 3 \ln 2 }$ .

(a) Sketch the curves represented by: (i) x = t/2, y = $1 - \frac { t ^ { 2 } } { 4 }$ (ii) x = sin t, y = 1 sin $2$ t (iii) x = e $t$ , y = 1 - e $2 t$ (b) Describe the differences between the three curves in part (a).(c) Produce Cartesian equations for the curves in parts (a)(i), (ii), and (iii) by eliminating the parameter. Compare your results.

Which of the following statements are true about the graph of the function y = 2 $x$ - 8? (i) It has no vertical asymptote.(ii) It has no horizontal asymptote.(iii) It has a y-intercept at -3.(iv) It has an x-intercept at 3.