Exam 14: Simple Linear Regression Analysis

An experiment was performed on a certain metal to determine if the strength is a function of heating time. The 95 percent confidence interval for the average strength of a metal sheet when the average heating time is 4 minutes is from 4.325 to 5.675. Therefore, we are confident at α = .05 that the average strength of this metal heated for four minutes is between 4.325 and 5.675 pounds per square inch. Do you agree or disagree with this statement?

An experiment was performed on a certain metal to determine if the strength is a function of heating time. Results based on 10 metal sheets are given below. ∑X = 30 ∑X² = 104 ∑Y = 40 ∑Y² = 178 ∑XY = 134 Using the simple linear regression model, find the estimated y-intercept.

When using simple regression analysis, if there is a strong correlation between the independent and dependent variables, then we can conclude that an increase in the value of the independent variable causes an increase in the value of the dependent variable.

A local tire dealer wants to predict the number of tires sold each month. He believes that the number of tires sold is a linear function of the amount of money invested in advertising. He randomly selects 6 months of data consisting of tire sales (in thousands of tires) and advertising expenditures (in thousands of dollars). Based on the data set with 6 observations, the simple linear regression equation of the least squares line is ŷ = 3 + 1x. ∑X = 24 ∑X² = 124 ∑Y = 42 ∑Y² = 338 ∑XY = 196 MSE = 4 Using the sums of the squares given above, determine the 90 percent prediction interval for tire sales in a month when the advertising expenditure is $5,000.

Regression Analysis ANOVA 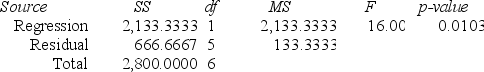Regression output A local grocery store wants to predict its daily sales in dollars. The manager believes that the amount of newspaper advertising significantly affects sales. He randomly selects 7 days of data consisting of daily grocery store sales (in thousands of dollars) and advertising expenditures (in thousands of dollars). The Excel/MegaStat output given above summarizes the results of the regression model. At a significance level of .05, test the significance of the slope and state your conclusion.

An experiment was performed on a certain metal to determine if the strength is a function of heating time. Results based on 10 metal sheets are given below. Use the simple linear regression model. ∑X = 30 ∑X² = 104 ∑Y = 40 ∑Y² = 178 ∑XY = 134 Determine the standard error.

Consider the following partial computer output from a simple linear regression analysis. 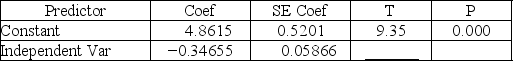S = .4862R-Sq = ________ Analysis of Variance Write the equation of the least squares line.

A local tire dealer wants to predict the number of tires sold each month. He believes that the number of tires sold is a linear function of the amount of money invested in advertising. He randomly selects 6 months of data consisting of tire sales (in thousands of tires) and advertising expenditures (in thousands of dollars). Based on the data set with 6 observations, the simple linear regression equation of the least squares line is ŷ = 3 + 1x. ∑X = 24 ∑X² = 124 ∑Y = 42 ∑Y² = 338 ∑XY = 196 MSE = 4 Using the sums of the squares given above, determine the 90 percent confidence interval for the mean value of monthly tire sales when the advertising expenditure is $5,000.

Consider the following partial computer output from a simple linear regression analysis. 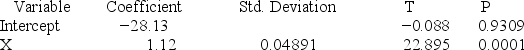R².9722 Test H₀: β₁ ≤ 0 vs. H_a: β₁ > 0.

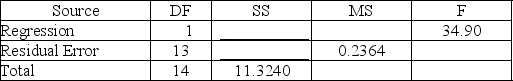
Complete the following partial ANOVA table from a simple linear regression analysis with a sample size of 16 observations. Find the F statistic to test the significance of the model at alpha = .05.

A local tire dealer wants to predict the number of tires sold each month. He believes that the number of tires sold is a linear function of the amount of money invested in advertising. He randomly selects 6 months of data consisting of tire sales (in thousands of tires) and advertising expenditures (in thousands of dollars). Based on the data set with 6 observations, the simple linear regression model yielded the following results. ∑X = 24 ∑X² = 124 ∑Y = 42 ∑Y² = 338 ∑XY = 196 Determine the value of the estimated y-intercept.

The notation Ŷ refers to the average value of the dependent variable Y.

A local tire dealer wants to predict the number of tires sold each month. He believes that the number of tires sold is a linear function of the amount of money invested in advertising. He randomly selects 6 months of data consisting of monthly tire sales (in thousands of tires) and monthly advertising expenditures (in thousands of dollars). Residuals are calculated for all of the randomly selected six months and ordered from smallest to largest. Determine the normal score for the smallest residual.

The experimental region is the range of the previously observed values of the dependent variable.

Consider the following partial computer output from a simple linear regression analysis. R².9722 Calculate the correlation coefficient.

Regression Analysis 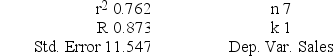ANOVA 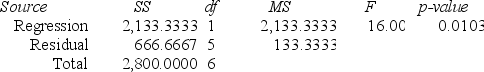Regression output A local grocery store wants to predict its daily sales in dollars. The manager believes that the amount of newspaper advertising significantly affects sales. He randomly selects 7 days of data consisting of daily grocery store sales (in thousands of dollars) and advertising expenditures (in thousands of dollars). The Excel/MegaStat output given above summarizes the results of the regression model. What is the value of the simple coefficient of determination?

Consider the following partial computer output from a simple linear regression analysis. 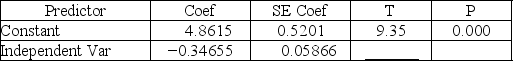S = .4862R-Sq = ________ Analysis of Variance What is the unexplained variance?

Use the least squares regression equation ŷ = 12.36 + 4.745X and determine the predicted value of y when x = 3.25.

All of the following are assumptions of the error terms in the simple linear regression model except

An experiment was performed on a certain metal to determine if the strength is a function of heating time. Results based on 10 metal sheets are given below. Use the simple linear regression model. ∑X = 30 ∑X² = 104 ∑Y = 40 ∑Y² = 178 ∑XY = 134 Calculate the coefficient of determination.