Exam 11: Statistical Inferences Based on Two Samples

When comparing two independent population means by using samples selected from two independent, normally distributed populations with equal variances, the correct test statistic to use is ________.

In a market research study conducted by a local winery on white wine preference, the following results were found. Of a sample of 500 men, 120 preferred white wine. Of a sample of 500 women, 210 preferred white wine. Set up the alternative hypothesis that will test the claim that the percentage of women who prefer white wine is more than 25 percent higher than the percentage of men who prefer white wine.

Two hospital emergency rooms use different procedures for triage of their patients. We want to test the claim that the mean waiting time of patients is the same for both hospitals. The 40 randomly selected subjects from hospital A produce a mean of 18.3 minutes. The 50 randomly selected patients from hospital B produce a mean of 25.31 minutes. Assume s_a = 2.1 minutes and s_b = 2.92 minutes. Calculate the test statistic for testing the hypothesis that there is a difference in the mean waiting time between the two hospitals. Assume unequal variances.

Two different firms design their own tests for business graduates, and an employer administers both versions to a random selection of prospective employees. Results are below. At α = .02, test the claim that both versions produce the same score. Mean difference = −4.25 Standard error of the difference = 1.411

When comparing the variances of two normally distributed populations using independent random samples, the correct test statistic to use is ________.

A fast-food company uses two management-training methods. Method 1 is a traditional method of training, and Method 2 is a new and innovative method. The company has just hired 31 new management trainees. 15 of the trainees are randomly selected and assigned to the first method, and the remaining 16 trainees are assigned to the second training method. After three months of training, the management trainees take a standardized test. The test was designed to evaluate their performance and learning from training. The sample mean score and sample standard deviation of the two methods are given below. The management wants to determine if the company should implement the new training method. 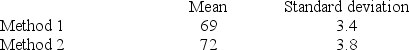Is there evidence at α = .01 to conclude that the new training method is more effective than the traditional training method?

When testing the difference between two population proportions, the ________ test statistic is used.

In an opinion survey, a random sample of 1,000 adults from the United States and 1,000 adults from Germany were asked whether they supported the death penalty. 590 American adults and 560 German adults indicated that they supported the death penalty. The researcher wants to know whether there is sufficient evidence to conclude that the proportion of adults who support the death penalty is higher in the United States than in Germany. What is the test statistic?

In testing the difference between two means from two independent populations, the sample sizes do not have to be equal.

Let p₁ represent the population proportion of U.S. senatorial and congressional (House of Representatives) Democrats who are in favor of a new modest tax on junk food. Let p₂ represent the population proportion of U.S. senatorial and congressional Republicans who are in favor of a new modest tax on junk food. Out of the 265 Democratic senators and members of Congress, 106 of them are in favor of a junk food tax. Out of the 285 Republican senators and members of Congress, only 57 are in favor of a junk food tax. At α = .01, can we conclude that the proportion of Democrats who favor a junk food tax is more than 5 percent higher than the proportion of Republicans who favor the new tax (using critical value rules)?

A fast-food company uses two management-training methods. Method 1 is a traditional method of training, and Method 2 is a new and innovative method. The company has just hired 31 new management trainees. 15 of the trainees are randomly selected and assigned to Method 1, and the remaining 16 trainees are assigned to Method 2. After three months of training, the management trainees take a standardized test. The test is designed to evaluate their performance and learning from the training. The sample mean score and sample standard deviation of the two methods are given below. Company management wants to determine whether the company should implement the new training method. 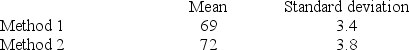Write the null and alternative hypotheses.

Find a 95 percent confidence interval for the difference between the proportions of older and younger drivers who have tickets, where 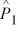= .275, 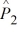= .25, n₁ = 1000, and n₂ = 1000.

In forming a confidence interval for μ₁ − μ₂, only two assumptions are required: independent samples and sample sizes of at least 30.

A market research study conducted by a local winery on white wine preference found the following results. Of a sample of 500 men, 120 preferred white wine. Of a sample of 500 women, 210 preferred white wine. What do you conclude at α = .05 about the claim that the proportion of women who prefer white wine is more than 25 percent higher than the proportion of men who prefer white wine?

Find a 95 percent confidence interval for the difference between means, where n₁ = 50, n₂ = 36, 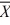₁ = 80, ₂ = 75, s₁² = 5, and s₂² = 3. Assume unequal variances.

A fast-food company uses two management-training methods. Method 1 is a traditional method of training, and Method 2 is a new and innovative method. The company has just hired 31 new management trainees. 15 of the trainees are randomly selected and assigned to the first method, and the remaining 16 trainees are assigned to the second training method. After three months of training, the management trainees take a standardized test. The test was designed to evaluate their performance and learning from training. The sample mean score and sample standard deviation of the two methods are given below. The management wants to determine if the company should implement the new training method. 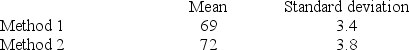What is the absolute value of the rejection point (critical value of the test statistic) at α = .01?

When testing H₀: σ₁² ≤ σ₂² and H_A: σ₁² > σ₂², where s₁² = .004, s₂² = .002, n₁ = 4, and n₂ = 7 at α = .05, what is the decision on H₀?

A coffee shop franchise owner is looking at two possible locations for a new shop. To help him decide, he looks at the number of pedestrians that go by each of the two locations in one-hour segments. At location A, counts are taken for 35 one-hour units, with a mean number of pedestrians of 421 and a sample standard deviation of 122. At the second location (B), counts are taken for 50 one-hour units, with a mean number of pedestrians of 347 and a sample standard deviation of 85. Assume the two populations variances are not known but are equal. Testing the claim that both sites have the same mean number of pedestrians at α = .01, what do you conclude?

In testing the difference between two independent population means, it is assumed that the level of measurement is ________.

We are testing the hypothesis that the proportion of winter-quarter profit growth is more than 2 percent greater for consumer industry companies (CON) than for banking companies (BKG). At α = .10, given that 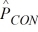= .20, 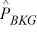= .14, n_CON = 300, and n_BKG = 400, calculate the estimated standard deviation for the model.