Exam 13: Experimental Design and Analysis of Variance
Exam 1: Data and Statistics98 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Displays62 Questions
Exam 3: Descriptive Statistics: Numerical Measures173 Questions
Exam 4: Introduction to Probability138 Questions
Exam 5: Discrete Probability Distributions123 Questions
Exam 6: Continuous Probability Distributions174 Questions
Exam 7: Sampling and Sampling Distributions133 Questions
Exam 8: Interval Estimation137 Questions
Exam 9: Hypothesis Tests148 Questions
Exam 10: Inference About Means and Proportions With Two Populations121 Questions
Exam 11: Inferences About Population Variances90 Questions
Exam 12: Comparing Multiple Proportions, Test of Independence and Goodness of Fit90 Questions
Exam 13: Experimental Design and Analysis of Variance115 Questions
Exam 14: Simple Linear Regression146 Questions
Exam 15: Multiple Regression115 Questions
Exam 16: Regression Analysis: Model Building76 Questions
Exam 17: Time Series Analysis and Forecasting68 Questions
Exam 18: Nonparametric Methods81 Questions
Exam 19: Statistical Methods for Quality Control29 Questions
Exam 20: Index Numbers52 Questions
Exam 21: Decision Analysis on Website65 Questions
Exam 22: Sample Survey on Website63 Questions
Select questions type
Information regarding random samples of annual salaries (in thousands of dollars) of doctors in three different specialties is shown below. 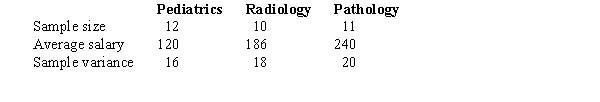 a.Compute the overall mean
a.Compute the overall mean  .
b.State the null and alternative hypotheses to be tested.
c.Show the complete ANOVA table for this test including the test statistic.
d.The null hypothesis is to be tested at 95% confidence. Determine the critical value for this test. What do you conclude?
e.Determine the p-value and use it for the test.
.
b.State the null and alternative hypotheses to be tested.
c.Show the complete ANOVA table for this test including the test statistic.
d.The null hypothesis is to be tested at 95% confidence. Determine the critical value for this test. What do you conclude?
e.Determine the p-value and use it for the test.
(Essay)
4.8/5  (36)
(36)
Exhibit 13-3
To test whether or not there is a difference between treatments A, B, and C, a sample of 12 observations has been randomly assigned to the 3 treatments. You are given the results below. 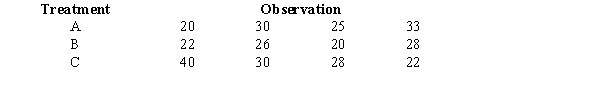 -Refer to Exhibit 13-3. The test statistic to test the null hypothesis equals
-Refer to Exhibit 13-3. The test statistic to test the null hypothesis equals
(Multiple Choice)
4.9/5  (43)
(43)
Exhibit 13-4
In a completely randomized experimental design involving five treatments, 13 observations were recorded for each of the five treatments (a total of 65 observations). The following information is provided.
SSTR = 200 (Sum Square Between Treatments)
SST = 800 (Total Sum Square)
-Refer to Exhibit 13-4. The number of degrees of freedom corresponding to between treatments is
(Multiple Choice)
4.9/5  (36)
(36)
Random samples were selected from three populations. The data obtained are shown below. Please note that the sample sizes are not equal.  a.Compute the overall mean
a.Compute the overall mean  .
b.At 95% confidence, test to see if there is a significant difference among the means.
.
b.At 95% confidence, test to see if there is a significant difference among the means.
(Essay)
4.8/5  (26)
(26)
In order to determine whether or not the means of two populations are equal,
(Multiple Choice)
4.7/5  (29)
(29)
Exhibit 13-3
To test whether or not there is a difference between treatments A, B, and C, a sample of 12 observations has been randomly assigned to the 3 treatments. You are given the results below.  -Refer to Exhibit 13-3. The null hypothesis is to be tested at the 1% level of significance. The p-value is
-Refer to Exhibit 13-3. The null hypothesis is to be tested at the 1% level of significance. The p-value is
(Multiple Choice)
4.9/5  (31)
(31)
Exhibit 13-4
In a completely randomized experimental design involving five treatments, 13 observations were recorded for each of the five treatments (a total of 65 observations). The following information is provided.
SSTR = 200 (Sum Square Between Treatments)
SST = 800 (Total Sum Square)
-Refer to Exhibit 13-4. The number of degrees of freedom corresponding to within treatments is
(Multiple Choice)
4.9/5  (27)
(27)
An ANOVA procedure is applied to data obtained from 6 samples where each sample contains 20 observations. The degrees of freedom for the critical value of F are
(Multiple Choice)
4.7/5  (36)
(36)
In ANOVA, which of the following is not affected by whether or not the population means are equal?
(Multiple Choice)
4.8/5  (29)
(29)
The heating bills for a selected sample of houses using various forms of heating are given below. (Values are in dollars.) 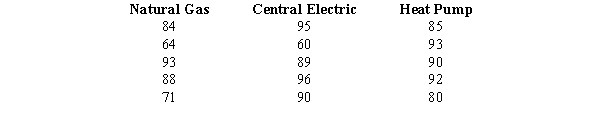 a.At = 0.05, test to see if there is a significant difference among the average heating bills of the homes. Use the p-value approach.
b.Test the above hypotheses using the critical value approach. Let = .05.
a.At = 0.05, test to see if there is a significant difference among the average heating bills of the homes. Use the p-value approach.
b.Test the above hypotheses using the critical value approach. Let = .05.
(Essay)
4.7/5  (29)
(29)
The required condition for using an ANOVA procedure on data from several populations is that the
(Multiple Choice)
4.9/5  (34)
(34)
The heating bills for a selected sample of houses using various forms of heating are given below (values are in dollars). 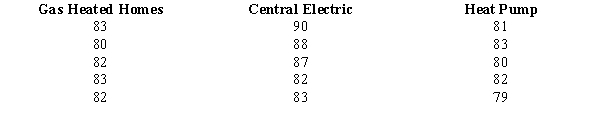 At = 0.05, test to see if there is a significant difference among the average bills of the homes. Use both the critical and p-value approaches.
At = 0.05, test to see if there is a significant difference among the average bills of the homes. Use both the critical and p-value approaches.
(Essay)
4.9/5  (36)
(36)
An experimental design that permits statistical conclusions about two or more factors is a
(Multiple Choice)
4.9/5  (41)
(41)
When an analysis of variance is performed on samples drawn from K populations, the mean square between treatments (MSTR) is
(Multiple Choice)
4.8/5  (30)
(30)
The F ratio in a completely randomized ANOVA is the ratio of
(Multiple Choice)
4.9/5  (37)
(37)
Random samples were selected from three populations. The data obtained are shown below. Please note that the sample sizes are not equal.  a.Compute the overall mean
a.Compute the overall mean  .
b.At 95% confidence using the critical value and p-value approach, test to see if there is a significant difference among the means.
.
b.At 95% confidence using the critical value and p-value approach, test to see if there is a significant difference among the means.
(Essay)
4.7/5  (46)
(46)
For four populations, the population variances are assumed to be equal. Random samples from each population provide the following data.  Using a .05 level of significance, test to see if the means for all four populations are the same.
Using a .05 level of significance, test to see if the means for all four populations are the same.
(Essay)
4.8/5  (39)
(39)
Six observations were selected from each of three populations. The data obtained is shown below.  Test at the = 0.05 level to determine if there is a significant difference in the means of the three populations. Use both the critical value and the p-value approaches.
Test at the = 0.05 level to determine if there is a significant difference in the means of the three populations. Use both the critical value and the p-value approaches.
(Essay)
4.8/5  (40)
(40)
An ANOVA procedure is used for data obtained from five populations. five samples, each comprised of 20 observations, were taken from the five populations. The numerator and denominator (respectively) degrees of freedom for the critical value of F are
(Multiple Choice)
4.8/5  (31)
(31)
Exhibit 13-4
In a completely randomized experimental design involving five treatments, 13 observations were recorded for each of the five treatments (a total of 65 observations). The following information is provided.
SSTR = 200 (Sum Square Between Treatments)
SST = 800 (Total Sum Square)
-Refer to Exhibit 13-4. The mean square within treatments (MSE) is
(Multiple Choice)
4.7/5  (34)
(34)
Showing 61 - 80 of 115
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)