Exam 9: Introduction to Hypothesis Testing

When the hypothesized proportion is close to 0.50, the spread in the sampling distribution of is greater than when the hypothesized proportion is close to 0.0 or 1.0.

For the following hypothesis test With n = 100 and p = 0.66, state the calculated value of the test statistic.

A local medical center has advertised that the mean wait for services will be less than 15 minutes. In an effort to test whether this claim can be substantiated, a random sample of 100 customers was selected and their wait times were recorded. The mean wait time was 17.0 minutes. Based on this sample result, there is sufficient evidence to reject the medical center's claim.

In a hypothesis test involving a population mean, which of the following would be an acceptable formulation?

The director of a state agency believes that the average starting salary for clerical employees in the state is less than $30,000 per year. To test her hypothesis, she has collected a simple random sample of 100 starting clerical salaries from across the state and found that the sample mean is $29,750. State the appropriate null and alternative hypotheses.

If a hypothesis test leads to incorrectly rejecting the null hypothesis, a Type II statistical error has been made.

Explain what is meant by a p-value.

The R.D. Wilson Company makes a soft drink dispensing machine that allows customers to get soft drinks from the machine in a cup with ice. When the machine is running properly, the average number of fluid ounces in the cup should be 14. Periodically the machines need to be tested to make sure that they have not gone out of adjustment. To do this, six cups are filled by the machine and a technician carefully measures the volume in each cup. In one such test, the following data were observed: 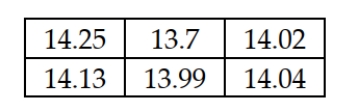Based on these sample data, which of the following is true if the significance level is .05?

A major issue facing many states is whether to legalize casino gambling. Suppose the governor of one state believes that more than 55% of the state's registered voters would favor some form of legal casino gambling. However, before backing a proposal to allow such gambling, the governor has instructed his aides to conduct a statistical test on the issue. To do this, the aides have hired a consulting firm to survey a simple random sample of 300 voters in the state. Of these 300 voters, 175 actually favored legalized gambling. Assuming that a significance level of 0.05 is used, what conclusion should the governor reach based on these sample data?

Mike runs for the president of the student government and is interested to know whether the proportion of the student body in favor of him is significantly more than 50 percent. A random sample of 100 students was taken. Fifty-five of them favored Mike. At a 0.05 level of significance, it can be concluded that the proportion of the students in favor of Mike

You are given the following null and alternative hypotheses: 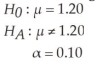If the true population mean is 1.25, determine the value of beta. Assume the population standard deviation is known to be 0.50 and the sample size is 60.

The R.D. Wilson Company makes a soft drink dispensing machine that allows customers to get soft drinks from the machine in a cup with ice. When the machine is running properly, the average number of fluid ounces in the cup should be 14. Periodically the machines need to be tested to make sure that they have not gone out of adjustment. To do this, six cups are filled by the machine and a technician carefully measures the volume in each cup. In one such test, the following data were observed: 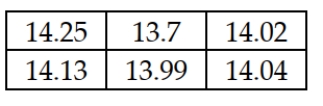Which of the following would be the correct null hypothesis if the company wishes to test the machine?

Which of the following is not a required step in finding beta?

A major issue facing many states is whether to legalize casino gambling. Suppose the governor of one state believes that more than 55% of the state's registered voters would favor some form of legal casino gambling. However, before backing a proposal to allow such gambling, the governor has instructed his aides to conduct a statistical test on the issue. To do this, the aides have hired a consulting firm to survey a simple random sample of 300 voters in the state. Of these 300 voters, 175 actually favored legalized gambling. State the appropriate null and alternative hypotheses.

If the Type I error (α) for a given test is to be decreased, then for a fixed sample size n:

A consumer group plans to test whether a new passenger car that is advertised to have a mean highway miles per gallon of at least 33 actually meets this level. They plan to test the hypothesis using a significance level of 0.05 and a sample size of n = 100 cars. It is believed that the population standard deviation is 3 mpg. Based upon this information, what is the critical value in terms of miles per gallon that would be needed prior to finding beta?

For the following hypothesis test: 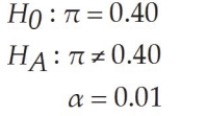With n= 64 and p= 0.42, state the decision rule in terms of the critical value of the test statistic

When someone has been accused of a crime the null hypothesis is: H0 : innocent. In this case, a Type I error would be convicting an innocent person.

Which of the following would be an appropriate null hypothesis?

If an economist wishes to determine whether there is evidence that average family income in a community near Seattle exceeds $125,000. An appropriate alternative hypothesis is: