Exam 9: Introduction to Hypothesis Testing

The null and alternate hypotheses must be opposites of each other.

The National Club Association does periodic studies on issues important to its membership. The 2012 Executive Summary of the Club Managers Association of America reported that the average country club initiation fee was $31,912. Suppose a random sample taken in 2009 of 12 country clubs produced the following initiation fees: Based on the sample information, can you conclude at the α = 0.05 level of significance that the average 2009 country club initiation fees are lower than the 2008 average? Conduct your test at the level of significance.

A large tire manufacturing company has claimed that its top line tire will average more than 80,000 miles. If a consumer group wished to test this claim, the research hypothesis would be: Ha : μ > 80,000 miles.

A house cleaning service claims that it can clean a four bedroom house in less than 2 hours. A sample of n = 16 houses is taken and the sample mean is found to be 1.97 hours and the sample standard deviation is found to be 0.1 hours. Using a 0.05 level of significance the correct conclusion is:

The loan manager for State Bank and Trust has claimed that the mean loan balance on outstanding loans at the bank is over $14,500. To test this at a significance level of 0.05, a random sample of n = 100 loan accounts is selected. Assuming that the population standard deviation is known to be $3,000, the value of that corresponds to the critical value is approximately $14,993.50.

The reason for using the t-distribution in a hypothesis test about the population mean is:

Woof Chow Dog Food Company believes that it has a market share of 25 percent. It surveys n = 100 dog owners and ask whether or not Woof Chow is their regular brand of dog food. The appropriate null and alternate hypotheses are:

Generally, it is possible to appropriately test a null and alternative hypotheses using the test statistic approach and reach a different conclusion than would be reached if the p-value approach were used.

For the following hypothesis test: With n = 80, σ = 9, and = 47.1, state the calculated value of the test statistic z.

For testing a research hypothesis, the burden of proof that a new product is no better than the original is placed on the new product, and the research hypothesis is formulated as the null hypothesis.

For the following hypothesis:

The cost of a college education has increased at a much faster rate than costs in general over the past twenty years. In order to compensate for this, many students work part- or full-time in addition to attending classes. At one university, it is believed that the average hours students work per week exceeds 20. To test this at a significance level of 0.05, a random sample of n = 20 students was selected and the following values were observed: Based on these sample data, the critical value expressed in hours:

A large tire manufacturing company has claimed that its top line tire will average more than 80,000 miles. If a consumer group wished to test this claim, they would formulate the following null and alternative hypotheses: H0 : μ ≥ 80,000 Hα : μ ≠ 80,000

A company that makes shampoo wants to test whether the average amount of shampoo per bottle is 16 ounces. The standard deviation is known to be 0.20 ounces. Assuming that the hypothesis test is to be performed using 0.10 level of significance and a random sample of n = 64 bottles, which of the following would be the correct formulation of the null and alternative hypotheses?

The U.S. Bureau of Labor Statistics (www.bls.gov) released its Consumer Expenditures report in October 2008. Among its findings is that average annual household spending on food at home was $3,624. Suppose a random sample of 137 households in Detroit was taken to determine whether the average annual expenditure on food at home was less for consumer units in Detroit than in the nation as a whole. The sample results are in the file Detroit Eats. Based on the sample results, can it be concluded at the α = 0.02 level of significance that average consumer-unit spending for food at home in Detroit is less than the national average?

A hypothesis test is to be conducted using an alpha = .01 level. This means:

You are given the following null and alternative hypotheses: 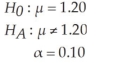If the true population mean is 1.25, calculate the power of the test. Assume the population standard deviation is known to be 0.50 and the sample size is 60.

After completing sales training for a large company, it is expected that the salesperson will generate a sale on at least 15 percent of the calls he or she makes. To make sure that the sales training process is working, a random sample of n = 400 sales calls made by sales representatives who have completed the training have been selected and the null hypothesis is to be tested at 0.05 alpha level. Suppose that a sale is made on 36 of the calls. Based on this information, what is the test statistic for this test?

A two-tailed hypothesis test is used when the null hypothesis looks like the following: H0 : = 100.

A company that makes and markets a device that is aimed at helping people quit smoking claims that at least 70 percent of the people who have used the product have quit smoking. To test this, a random sample of n = 100 product users was selected. Of these, 65 people were found to have quit smoking. Given these results, the test statistic value is z = -1.0911.