Exam 7: Sampling Distributions
Exam 1: Describing Data With Graphs94 Questions
Exam 2: Describing Data With Numerical Measures186 Questions
Exam 3: Describing Bivariate Data35 Questions
Exam 4: Probability and Probability Distributions136 Questions
Exam 5: Several Useful Discrete Distributions129 Questions
Exam 6: The Normal Probability Distribution196 Questions
Exam 7: Sampling Distributions162 Questions
Exam 8: Large-Sample Estimation173 Questions
Exam 9: Large-Sample Tests of Hypotheses210 Questions
Exam 10: Inference From Small Samples261 Questions
Exam 11: The Analysis of Variance156 Questions
Exam 12: Linear Regression and Correlation165 Questions
Exam 13: Multiple Regression Analysis178 Questions
Exam 14: Analysis of Categorical Data136 Questions
Exam 15: Nonparametric Statistics198 Questions
Select questions type
The time necessary to complete an examination has a mean of 45 minutes and a standard deviation of 4 minutes. The average time necessary for 64 randomly selected students is computed.
What is the mean of the sampling distribution of  ?
______________
What is the standard deviation of the sampling distribution of
?
______________
What is the standard deviation of the sampling distribution of 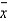 ?
______________
Can we say that the sampling distribution of
?
______________
Can we say that the sampling distribution of 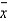 is approximately normally distributed?
______________
Why?
________________________________________________________
is approximately normally distributed?
______________
Why?
________________________________________________________
(Essay)
4.9/5  (37)
(37)
Assignable cause variation refers to variation in the output of a process that is naturally occurring, expected, and that may be the result of random causes.
(True/False)
4.8/5  (37)
(37)
Suppose a random sample of 25 students is selected from a community college where the scores in the final exam (out of 125 points) are normally distributed, with mean equal to 112 and standard deviation equal to 12.
What is the mean of the sampling distribution of the sample mean 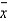 ?
______________
What is the standard deviation?
______________
Find the probability that
?
______________
What is the standard deviation?
______________
Find the probability that  exceeds 116.
______________
Find the probability that the sample mean deviates from the population mean
exceeds 116.
______________
Find the probability that the sample mean deviates from the population mean 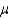 = 112 by no more than 4.
______________
= 112 by no more than 4.
______________
(Short Answer)
4.8/5  (34)
(34)
Fifty samples of size 500 were drawn from a manufacturing process and the number of defectives in each sample was counted. The average of the sample proportion was 0.032. The centerline for the p chart is:
(Multiple Choice)
4.9/5  (42)
(42)
A sampling distribution is a probability distribution that shows the likelihood of occurrence associated with all the possible values of a parameter, which values would be obtained when drawing all possible samples of a given size from a population.
(True/False)
4.8/5  (32)
(32)
The most important contribution of the Central Limit Theorem is in statistical inference.
(True/False)
4.8/5  (38)
(38)
In control process is typically defined as a process in which all output is operating within  3 standard deviation of the centerline of the process.
3 standard deviation of the centerline of the process.
(True/False)
4.7/5  (42)
(42)
A southern state has an unemployment rate of 6%. The state conducts monthly surveys in order to track the unemployment rate. In a recent month, a random sample of 700 people showed that 35 were unemployed.
If the true unemployment rate is 6%, describe the sampling distribution of 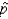 . Is the sampling distribution of the sample proportion approximately normal?
______________
What is the mean?
______________
What is the standard deviation?
______________
Find the probability that the sample unemployment rate is at most 5%.
______________
Assume the population proportion, p, is unknown. Describe the sampling distribution of
. Is the sampling distribution of the sample proportion approximately normal?
______________
What is the mean?
______________
What is the standard deviation?
______________
Find the probability that the sample unemployment rate is at most 5%.
______________
Assume the population proportion, p, is unknown. Describe the sampling distribution of  based on the most recent sample. Is the sampling distribution of the sample proportion approximately normal?
______________
What is the mean?
______________
What is the standard deviation?
______________
Based on the most recent sample, find the probability that the sample proportion will lie within 0.005 of the true proportion p of people who are unemployed.
______________
based on the most recent sample. Is the sampling distribution of the sample proportion approximately normal?
______________
What is the mean?
______________
What is the standard deviation?
______________
Based on the most recent sample, find the probability that the sample proportion will lie within 0.005 of the true proportion p of people who are unemployed.
______________
(Short Answer)
4.9/5  (32)
(32)
The publisher of a newspaper decides which articles will be submitted for the consideration of the Pulitzer Prize committee. This is an example of cluster sampling.
(True/False)
4.9/5  (49)
(49)
In a learning experiment, untrained mice are placed in a maze and the time required for each mouse to exit the maze is recorded. The average time for untrained mice to exit the maze is 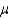 = 50 seconds and the standard deviation of their times is
= 50 seconds and the standard deviation of their times is 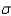 = 16 seconds. 64 randomly selected untrained mice are placed in the maze and the time necessary to exit the maze is recorded for each.
Find the mean of the sampling distribution of
= 16 seconds. 64 randomly selected untrained mice are placed in the maze and the time necessary to exit the maze is recorded for each.
Find the mean of the sampling distribution of 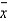 .
______________
Find the standard deviation.
______________
What is the probability that the sample mean differs from the population mean by more than 3?
______________
.
______________
Find the standard deviation.
______________
What is the probability that the sample mean differs from the population mean by more than 3?
______________
(Short Answer)
4.7/5  (43)
(43)
A group of people who, in response to some general appeal, have selected themselves to participate in a survey is called a simple random sample.
(True/False)
4.8/5  (24)
(24)
According to one study, 50% of Americans admit to overeating sweet foods when stressed. Suppose that the 50% figure is correct and that a random sample of n = 100 Americans is selected.
Does the distribution of 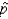 , the sample proportion of Americans who relieve stress by overeating sweet foods, have an approximately normal distribution?
______________
What is the mean?
______________
What is the standard deviation?
______________
What is the probability that the sample proportion,
, the sample proportion of Americans who relieve stress by overeating sweet foods, have an approximately normal distribution?
______________
What is the mean?
______________
What is the standard deviation?
______________
What is the probability that the sample proportion,  , exceeds 0.54?
______________
What is the probability that
, exceeds 0.54?
______________
What is the probability that 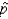 lies within the interval 0.39 to 0.59?
______________
Would it be unusual if the sample proportion were as small as 34%?
______________
lies within the interval 0.39 to 0.59?
______________
Would it be unusual if the sample proportion were as small as 34%?
______________
(Short Answer)
4.7/5  (40)
(40)
Which of the following statements about the Central Limit Theorem (CLT) is correct?
(Multiple Choice)
5.0/5  (29)
(29)
In practical business situations, it is very unlikely that a decision maker will actually construct a sampling distribution of any kind.
(True/False)
5.0/5  (25)
(25)
Statistical process control (SPC) methodology was developed to monitor, control, and improve products and services.
(True/False)
4.9/5  (32)
(32)
A sampling distribution is the distribution of the values that are included in a sample selected randomly from the population.
(True/False)
4.8/5  (25)
(25)
Suppose a sample of 120 items is drawn from a population of manufactured products and the number of defective items is recorded. Prior experience has shown that the proportion of defectives is 0.05.
Is the sampling distribution of the proportion of defectives approximately normal?
______________
What is the mean?
______________
What is the standard deviation?
______________
What is the probability that the sample proportion is less than 0.10?
______________
How would the sampling distribution of the sample proportion change if the sample size were raised to 200?
________________________________________________________
(Essay)
4.7/5  (38)
(38)
It is known that the birth weight of newborn babies in the U.S. has a mean of 7 pounds with a standard deviation of 1.6 pounds. Suppose we randomly sample 64 birth certificates from the State Health Department, and record the birth weights of these babies.
Find the mean of the sampling distribution of 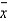 .
______________
Find the standard deviation.
______________
What is the probability the sample mean birth weight as recorded on the birth certificates will be less than 6.85 pounds?
______________
.
______________
Find the standard deviation.
______________
What is the probability the sample mean birth weight as recorded on the birth certificates will be less than 6.85 pounds?
______________
(Short Answer)
4.8/5  (28)
(28)
The distribution of scores for the third exam in a statistics course has a mean of 74 and a standard deviation of 15. A random sample of 36 exam papers is selected.
What is the probability that the sample mean is higher than 77?
______________
(Short Answer)
4.9/5  (32)
(32)
Showing 101 - 120 of 162
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)