Exam 11: The Analysis of Variance
Exam 1: Describing Data With Graphs94 Questions
Exam 2: Describing Data With Numerical Measures186 Questions
Exam 3: Describing Bivariate Data35 Questions
Exam 4: Probability and Probability Distributions136 Questions
Exam 5: Several Useful Discrete Distributions129 Questions
Exam 6: The Normal Probability Distribution196 Questions
Exam 7: Sampling Distributions162 Questions
Exam 8: Large-Sample Estimation173 Questions
Exam 9: Large-Sample Tests of Hypotheses210 Questions
Exam 10: Inference From Small Samples261 Questions
Exam 11: The Analysis of Variance156 Questions
Exam 12: Linear Regression and Correlation165 Questions
Exam 13: Multiple Regression Analysis178 Questions
Exam 14: Analysis of Categorical Data136 Questions
Exam 15: Nonparametric Statistics198 Questions
Select questions type
In a two-way factor ANOVA, if factors A and B do not interact, then neither A nor B can be considered statistically significant.
Free
(True/False)
4.7/5  (41)
(41)
Correct Answer:
False
The number of degrees of freedom for the denominator in one-way ANOVA test involving 4 population means with 15 observations sampled from each population is:
Free
(Multiple Choice)
4.8/5  (34)
(34)
Correct Answer:
C
In a two-way factor ANOVA, the variances of the populations are assumed to be equal unless the error variation is zero.
Free
(True/False)
5.0/5  (22)
(22)
Correct Answer:
False
In one-way ANOVA, the amount of total variation that is unexplained is measured by:
(Multiple Choice)
4.9/5  (38)
(38)
The test statistic of the completely randomized ANOVA design equals:
(Multiple Choice)
4.8/5  (38)
(38)
Tukey's method for paired comparisons makes the probability of declaring that a difference exists between at least one pair in a set of k treatments, when no difference exists, equal to 1 - 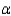 .
.
(True/False)
4.9/5  (32)
(32)
One-way ANOVA is performed on three independent samples with 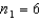 ,
, 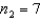 , and
, and 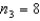 . The critical value obtained from the F-table for this test at the 2.5% level of significance equals:
. The critical value obtained from the F-table for this test at the 2.5% level of significance equals:
(Multiple Choice)
4.9/5  (33)
(33)
In an ANOVA test, the test statistic is F = 6.75. The rejection region is F > 3.97 for the 5% level of significance, F > 5.29 for the 2.5% level, and F > 7.46 for the 1% level. For this test, the p-value is:
(Multiple Choice)
4.9/5  (35)
(35)
In a two-way ANOVA, there are 4 levels for factor A, 5 levels for factor B, and 3 observations for each combination of factor A and factor B levels. The number of treatments in this experiment equals:
(Multiple Choice)
5.0/5  (39)
(39)
Physicians depend on laboratory test results when managing medical problems such as diabetes or epilepsy. In a uniformity test for glucose tolerance, three different laboratories were each sent nt = 5 identical blood samples from a person who had drunk 50 milligrams (mg) of glucose dissolved in water. The laboratory results (in mg/dl) are listed here: 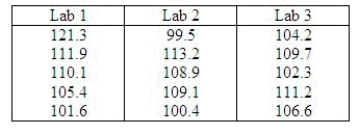 Do the data indicate a difference in the average readings for the three laboratories? Use Tukey's method for paired comparisons to rank the three treatment means. Use
Do the data indicate a difference in the average readings for the three laboratories? Use Tukey's method for paired comparisons to rank the three treatment means. Use 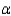 = 0.05.
What is the F-statistic?
______________
What is the p-value?
______________
Can you reject the null hypothesis?
______________
There ______________ sufficient evidence to indicate a difference in the treatment means.
= 0.05.
What is the F-statistic?
______________
What is the p-value?
______________
Can you reject the null hypothesis?
______________
There ______________ sufficient evidence to indicate a difference in the treatment means.
(Short Answer)
4.9/5  (25)
(25)
The number of cells in a two-way factor ANOVA is equal to a + b  1; where a is the number of levels of factor A and b is the number of levels of factor B.
1; where a is the number of levels of factor A and b is the number of levels of factor B.
(True/False)
4.8/5  (42)
(42)
In a two-way factor ANOVA with replications, the reason for separating out the sum of squares due to interaction between factors A and B is to increase the chance of detecting significant differences across levels of factor A and factor B.
(True/False)
4.9/5  (38)
(38)
In a two-way factor ANOVA, the sum of squares due to both factors, the interaction sum of squares, and the error sum of squares must add up to the total sum of squares.
(True/False)
4.8/5  (36)
(36)
In the analysis of variance, explained variation equals the treatments means square in one-way ANOVA.
(True/False)
4.7/5  (23)
(23)
Three tennis players, a beginner, an intermediate, and advanced, have been randomly selected from the membership of a racquet facility club in a large city. Using the same tennis ball, each player hits ten serves, one with each of three racquet models, with the three racquet models selected randomly. The speed of each serve is measured with a machine and the result recorded. Among the ANOVA models listed below, the most likely model to fit this situation is the:
(Multiple Choice)
4.9/5  (33)
(33)
In a two-way factor ANOVA with replications in which all hypotheses are to be tested at the .05 significance level, if the p-value for interaction is .0257, then we should conclude that no interaction exists between the levels of the two factors.
(True/False)
4.9/5  (37)
(37)
The F-test of the analysis of variance requires that the populations be normally distributed with equal variances.
(True/False)
4.9/5  (41)
(41)
In order to conduct a two-way factor ANOVA with replications, the number of replications r must be the same in each cell.
(True/False)
4.7/5  (35)
(35)
Showing 1 - 20 of 156
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)