Exam 3: Functions and Graphs
Exam 1: Algebra and Equations409 Questions
Exam 2: Graphs, Lines, and Inequalities255 Questions
Exam 3: Functions and Graphs323 Questions
Exam 4: Exponential and Logarithmic Functions192 Questions
Exam 5: Mathematics of Finance183 Questions
Exam 6: Systems of Linear Equations and Matrices215 Questions
Exam 7: Linear Programming203 Questions
Exam 8: Sets and Probability240 Questions
Exam 9: Counting, Probability Distributions, and Further Topics in Probability210 Questions
Exam 10: Introduction to Statistics169 Questions
Exam 11: Differential Calculus342 Questions
Exam 12: Applications of the Derivative220 Questions
Exam 13: Integral Calculus227 Questions
Exam 14: Multivariate Calculus152 Questions
Select questions type
Give the equation of the horizontal asymptote of the rational function.
-
(Multiple Choice)
4.9/5  (31)
(31)
Solve the problem.
-At a manufacturing plant, the total cost (in dollars) to produce items is .
What is the marginal cost per item?
(Multiple Choice)
4.7/5  (36)
(36)
Solve the problem.
-At a manufacturing plant, the total cost (in dollars) to produce items is
What is the average cost per item?
(Multiple Choice)
4.9/5  (34)
(34)
Find the rule of a quadratic function whose graph has the given vertex and passes through the given point.
-vertex ; point
(Multiple Choice)
4.9/5  (34)
(34)
Determine whether the following rule defines as a function of .
-The , y pairs:
(True/False)
4.7/5  (45)
(45)
Write the word or phrase that best completes each statement or answers the question.
-The table shows the number of new AIDS cases (in thousands) in the U.S. in five consecutive years.
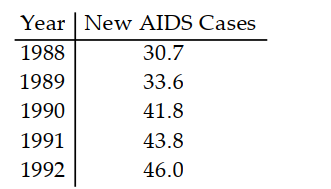 Let correspond to 1988 and let be the number of new AIDS cases (in thousands) in the U.S. in year . Using the points and , the following linear function is obtained to model the data: . What does the slope, 3.825 , indicate?
Let correspond to 1988 and let be the number of new AIDS cases (in thousands) in the U.S. in year . Using the points and , the following linear function is obtained to model the data: . What does the slope, 3.825 , indicate?
(Essay)
4.8/5  (36)
(36)
Give the equation of the vertical asymptote(s) of the rational function.
-
(Multiple Choice)
4.9/5  (32)
(32)
Solve the problem.
-An open-top box is to be made by cutting small identical squares from each corner of a 10-by - 10 -in. sheet of tin and bending up the sides. If each corner square is inches on a side, the volume of the box (in in. ) is given by: Find .
(Multiple Choice)
4.7/5  (36)
(36)
Solve the problem.
-A new car was purchased for , and 3 years later it was worth . Assume that the depreciation in value is given by a linear equation. Find the equation.
(Multiple Choice)
5.0/5  (31)
(31)
Use the vertical line test to determine if the graph is a graph of a function.
-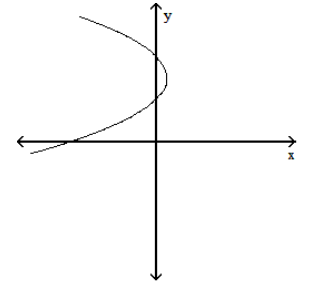
(True/False)
4.8/5  (27)
(27)
Solve the problem.
-Suppose the supply and demand for a certain videotape are given by:
Where is price and is quantity.
Find the equilibrium supply.
(Multiple Choice)
4.7/5  (32)
(32)
Solve the problem.
-Suppose that the population of a certain city during a certain time period can be approximated by:
Where is time in years since 1960. By sketching a graph of , estimate during what time period the population of the city was increasing.
(Multiple Choice)
4.8/5  (35)
(35)
Write the word or phrase that best completes each statement or answers the question
-Suppose that during a flu epidemic in a particular city, the number of people, , infected (in thousands) at the end of weeks is approximated by
What is the horizontal asymptote of the graph of this function? What does this suggest about the maximum number of people who will eventually be infected? Explain your reasoning.
(Essay)
4.9/5  (43)
(43)
Showing 221 - 240 of 323
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)