Question 1

Graph.
-$f(x)=-2(x-4)^{2}+2$

Accepted Answer

A)    
B)    
C)    
D)    
A)    
B)    
C)    
D)

Question 2

Solve the problem.
-A salesperson gets a commission of $\$ 1600$ for the first $\$ 10,000$ of sales, and then $\$ 800$ for each additional $\$ 10,000$ or fraction of $\$ 10,000$ of sales. Let $S(x)$ represent the commission on $x$ dollars of sales. Find the value of $S(\$ 65,000)$.

Accepted Answer

A)  $\$ 5200$ 
B)  $\$ 6400$ 
C)  $\$ 6800$ 
D)  $\$ 6000$ 
A)  $\$ 5200$ 
B)  $\$ 6400$ 
C)  $\$ 6800$ 
D)  $\$ 6000$

Question 3

Graph the function.
-$f(x)=|3 x-5|$

Accepted Answer

A)    
B)    
C)    
D)    
A)    
B)    
C)    
D)

Question 4

Give the equation of the horizontal asymptote of the rational function.
-$g(x)=\frac{x+2}{x^{2}-2}$

Accepted Answer

A)  $y=-2$ 
B)  $y=0$ 
C)  $y=2$ 
D)  None 
A)  $y=-2$ 
B)  $y=0$ 
C)  $y=2$ 
D)  None

Question 5

Graph the parabola.
-$f(x)=2 x^{2}-2 x+2$

Accepted Answer

A)    
B)    
C)    
D)    
A)    
B)    
C)    
D)

Question 6

Evaluate the function.
-Given that $f(x)=\sqrt{x}+\frac{3}{1-x}$, find $f(3.8)$.

Accepted Answer

A)  $\sqrt{3.8}-\frac{15}{14} \approx 0.8286$ 
B)  $\sqrt{3.8}-\frac{15}{14} \approx 13.3686$ 
C)  $\sqrt{3.8}-\frac{15}{14} \approx 3.0208$ 
D)  $\sqrt{3.8}-\frac{15}{14} \approx 0.8779$ 
A)  $\sqrt{3.8}-\frac{15}{14} \approx 0.8286$ 
B)  $\sqrt{3.8}-\frac{15}{14} \approx 13.3686$ 
C)  $\sqrt{3.8}-\frac{15}{14} \approx 3.0208$ 
D)  $\sqrt{3.8}-\frac{15}{14} \approx 0.8779$

Question 7

Find a quadratic function that models the data.
-The table shows the number of new AIDS cases among women in the U.S. in six consecutive years. Data are in thousands rounded to the nearest hundred.
 
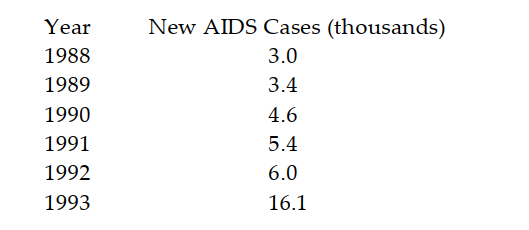Let $\mathrm{x}=1$ correspond to 1988 and let $\mathrm{f}(\mathrm{x})$ be the number of new AIDS cases (in thousands) among women in the U.S. in year $x$. Using the point $(1,3.0)$ as the vertex and $(6,16.1)$ as the other point, determine a quadratic function $f(x)=a(x-h)^{2}+k$ that models the data.

Accepted Answer

A)  $f(x)=0.764(x-1)^{2}-3.0$ 
B)  $f(x)=0.824(x-1)^{2}+3.0$ 
C)  $f(x)=0.364(x-1)^{2}+3.0$ 
D)  $f(x)=0.524(x-1)^{2}+3.0$ 
A)  $f(x)=0.764(x-1)^{2}-3.0$ 
B)  $f(x)=0.824(x-1)^{2}+3.0$ 
C)  $f(x)=0.364(x-1)^{2}+3.0$ 
D)  $f(x)=0.524(x-1)^{2}+3.0$

Question 8

For the given function, find $\frac{f(x+h)-f(x)}{h}$.
-$2-9 x$

Accepted Answer

A)  $\frac{h-18 x}{h}$ 
B)  1 
C)  -9 
D)  $\frac{-9 h-18 x}{h}$ 
A)  $\frac{h-18 x}{h}$ 
B)  1 
C)  -9 
D)  $\frac{-9 h-18 x}{h}$

Question 9

Evaluate the function.
-Given that $f(x)=\sqrt{x+5}$, find $f(-3)$

Accepted Answer

A)  $\sqrt{2} \approx 4$ 
B)  $\sqrt{5} \approx 2.2361$ 
C)  $\sqrt{2} \approx 1$ 
D)  $\sqrt{2} \approx 1.4142$ 
A)  $\sqrt{2} \approx 4$ 
B)  $\sqrt{5} \approx 2.2361$ 
C)  $\sqrt{2} \approx 1$ 
D)  $\sqrt{2} \approx 1.4142$

Question 10

Give the equation of the vertical asymptote(s) of the rational function.
-$F(x)=\frac{x-9}{x^{2}+5}$

Accepted Answer

A)  None 
B)  $x=-5$ 
C)  $x=5$ 
D)  $x=3, x=-3$ 
A)  None 
B)  $x=-5$ 
C)  $x=5$ 
D)  $x=3, x=-3$

Question 11

Give the equation of the vertical asymptote(s) of the rational function.
-$g(x)=\frac{x^{2}+8 x-4}{x^{3}-3 x^{2}+2 x}$

Accepted Answer

A)  $x=-1, x=-2$ 
B)  $x=1, x=2$ 
C)  $x=1, x=-1, x=-2$ 
D)  $x=0, x=1, x=2$ 
A)  $x=-1, x=-2$ 
B)  $x=1, x=2$ 
C)  $x=1, x=-1, x=-2$ 
D)  $x=0, x=1, x=2$

Question 12

Graph the linear function.
-$h(x)=6 x$

Accepted Answer

A)    
B)    
A)    
B)

Question 13

Find the $x$ and $y$ intercepts. If no $x$ intercepts exist, state so.
-$f(x)=x^{2}+20 x+100$

Accepted Answer

A)  $x$-intercept: 100; y-intercept: -10 
B)  $x$-intercept: -10; y-intercept: 100 
C)  $x$-intercept: 10; y-intercept: 100 
D)  x-intercept: 100; y-intercept: 10 
A)  $x$-intercept: 100; y-intercept: -10 
B)  $x$-intercept: -10; y-intercept: 100 
C)  $x$-intercept: 10; y-intercept: 100 
D)  x-intercept: 100; y-intercept: 10

Question 14

Solve the problem.
-A manufacturer bought a milling machine for $\$ 214,000$. It has a scrap value of $\$ 72,000$. The useful life can range from 5 to 10 years. The manufacturer wants to show no more than $\$ 16,279$ per year in depreciation. How many years (integer) should be used for its life?

Accepted Answer

A)  7 years 
B)  9 years 
C)  8 years 
D)  10 years 
A)  7 years 
B)  9 years 
C)  8 years 
D)  10 years

Question 15

Write the word or phrase that best completes each statement or answers the question.
-The table shows the number of new AIDS cases (in thousands) in the U.S. in five consecutive years.
   
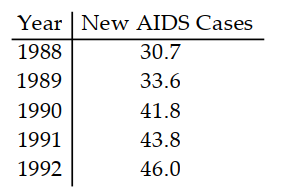Let $\mathrm{x}=1$ correspond to 1988 and let $\mathrm{f}(\mathrm{x})$ be the number of new AIDS cases (in thousands) in the U.S. in year $x$. Using the points $(1,30.7)$ and $(5,46.0)$, the following linear function is obtained to model the data: $\mathrm{f}(\mathrm{x})=3.825 \mathrm{x}+26.875$. Compare the number of new AIDS cases given by the model in 1990 with the actual number of new AIDS cases that year. How accurate is the model?

Accepted Answer

The model gives 38,350 new cas

Question 16

Write the word or phrase that best completes each statement or answers the question
-A new health food store runs an advertising campaign. Daily sales (in dollars) after $x$ days of advertising are given by
$$S(x)=\frac{2500 x+8500}{x+1}$$
By sketching the graph of this function, answer the following questions. What is the horizontal asymptote of the graph? What does this suggest about future sales? Explain your reasoning. If the advertising campaign costs $\$ 3200$ per day, at what point should it be discontinued? Why?

Accepted Answer

Answers will vary. Possible answer: The

Question 17

State the domain of the given function.
-$f(x)=9 x^{2}+9 x-9$

Accepted Answer

A)  $(-\infty, \infty)$ 
B)  all real numbers except 0 
C)  all whole numbers 
D)  $(0, \infty)$ 
A)  $(-\infty, \infty)$ 
B)  all real numbers except 0 
C)  all whole numbers 
D)  $(0, \infty)$

Question 18

Solve the problem.
-A charter flight charges a fare of $\$ 400$ per person plus $\$ 4$ per person for each unsold seat on the plane. If the plane holds 176 passengers and if $x$ represents the number of unsold seats, an expression for the total revenue received for the flight is $R(x)=(176-x)(400+4 x)$. Find the maximum revenue.

Accepted Answer

A)  $\$ 152,352$ 
B)  $\$ 76,676$ 
C)  $\$ 76,176$ 
D)  $\$ 71,676$ 
A)  $\$ 152,352$ 
B)  $\$ 76,676$ 
C)  $\$ 76,176$ 
D)  $\$ 71,676$

Question 19

Evaluate the function.
-Determine $g(a-1)$ when $g(x)=2 x-3$.

Accepted Answer

A)  $2 a-5$ 
B)  $2 a+1$ 
C)  $\frac{1}{2} a-3$ 
D)  $2 a-3$ 
A)  $2 a-5$ 
B)  $2 a+1$ 
C)  $\frac{1}{2} a-3$ 
D)  $2 a-3$

Question 20

Evaluate the function.
-Find $f(-2)$ when $f(x)=2 x^{2}-2 x+6$

Accepted Answer

A)  6 
B)  10 
C)  18 
D)  14 
A)  6 
B)  10 
C)  18 
D)  14

Graph. - $f(x)=-2(x-4)^{2}+2$ $Graph. - f(x)=-2(x-4)^{2}+2$

Solve the problem. -A salesperson gets a commission of $\$ 1600$ for the first $\$ 10,000$ of sales, and then $\$ 800$ for each additional $\$ 10,000$ or fraction of $\$ 10,000$ of sales. Let $S(x)$ represent the commission on $x$ dollars of sales. Find the value of $S(\$ 65,000)$ .

Graph the function. - $f(x)=|3 x-5|$

Give the equation of the horizontal asymptote of the rational function. - $g(x)=\frac{x+2}{x^{2}-2}$

Graph the parabola. - $f(x)=2 x^{2}-2 x+2$ $Graph the parabola. - f(x)=2 x^{2}-2 x+2$

Evaluate the function. -Given that $f(x)=\sqrt{x}+\frac{3}{1-x}$ , find $f(3.8)$ .

For the given function, find $\frac{f(x+h)-f(x)}{h}$ . - $2-9 x$

Evaluate the function. -Given that $f(x)=\sqrt{x+5}$ , find $f(-3)$

Give the equation of the vertical asymptote(s) of the rational function. - $F(x)=\frac{x-9}{x^{2}+5}$

Give the equation of the vertical asymptote(s) of the rational function. - $g(x)=\frac{x^{2}+8 x-4}{x^{3}-3 x^{2}+2 x}$

Graph the linear function. - $h(x)=6 x$

Find the $x$ and $y$ intercepts. If no $x$ intercepts exist, state so. - $f(x)=x^{2}+20 x+100$

Solve the problem. -A manufacturer bought a milling machine for $\$ 214,000$ . It has a scrap value of $\$ 72,000$ . The useful life can range from 5 to 10 years. The manufacturer wants to show no more than $\$ 16,279$ per year in depreciation. How many years (integer) should be used for its life?

State the domain of the given function. - $f(x)=9 x^{2}+9 x-9$

Evaluate the function. -Determine $g(a-1)$ when $g(x)=2 x-3$ .

Evaluate the function. -Find $f(-2)$ when $f(x)=2 x^{2}-2 x+6$

Algebra and Equations

Graphs, Lines, and Inequalities

Exponential and Logarithmic Functions

Mathematics of Finance

Systems of Linear Equations and Matrices

Linear Programming

Sets and Probability

Counting, Probability Distributions, and Further Topics in Probability

Introduction to Statistics

Differential Calculus

Applications of the Derivative

Integral Calculus

Multivariate Calculus

Filters

Exam 3: Functions and Graphs

Graph. - f(x)=−2(x−4)2+2f(x)=-2(x-4)^{2}+2f(x)=−2(x−4)2+2

Graph the function. - f(x)=∣3x−5∣f(x)=|3 x-5|f(x)=∣3x−5∣

Give the equation of the horizontal asymptote of the rational function. - g(x)=x+2x2−2g(x)=\frac{x+2}{x^{2}-2}g(x)=x2−2x+2​

Graph the parabola. - f(x)=2x2−2x+2f(x)=2 x^{2}-2 x+2f(x)=2x2−2x+2

Evaluate the function. -Given that f(x)=x+31−xf(x)=\sqrt{x}+\frac{3}{1-x}f(x)=x​+1−x3​ , find f(3.8)f(3.8)f(3.8) .

For the given function, find f(x+h)−f(x)h\frac{f(x+h)-f(x)}{h}hf(x+h)−f(x)​ . - 2−9x2-9 x2−9x

Evaluate the function. -Given that f(x)=x+5f(x)=\sqrt{x+5}f(x)=x+5​ , find f(−3)f(-3)f(−3)

Give the equation of the vertical asymptote(s) of the rational function. - F(x)=x−9x2+5F(x)=\frac{x-9}{x^{2}+5}F(x)=x2+5x−9​

Give the equation of the vertical asymptote(s) of the rational function. - g(x)=x2+8x−4x3−3x2+2xg(x)=\frac{x^{2}+8 x-4}{x^{3}-3 x^{2}+2 x}g(x)=x3−3x2+2xx2+8x−4​

Graph the linear function. - h(x)=6xh(x)=6 xh(x)=6x

Find the xxx and yyy intercepts. If no xxx intercepts exist, state so. - f(x)=x2+20x+100f(x)=x^{2}+20 x+100f(x)=x2+20x+100

State the domain of the given function. - f(x)=9x2+9x−9f(x)=9 x^{2}+9 x-9f(x)=9x2+9x−9

Evaluate the function. -Determine g(a−1)g(a-1)g(a−1) when g(x)=2x−3g(x)=2 x-3g(x)=2x−3 .

Evaluate the function. -Find f(−2)f(-2)f(−2) when f(x)=2x2−2x+6f(x)=2 x^{2}-2 x+6f(x)=2x2−2x+6

Algebra and Equations

Graphs, Lines, and Inequalities

Exponential and Logarithmic Functions

Mathematics of Finance

Systems of Linear Equations and Matrices

Linear Programming

Sets and Probability

Counting, Probability Distributions, and Further Topics in Probability

Introduction to Statistics

Differential Calculus

Applications of the Derivative

Integral Calculus

Multivariate Calculus

Filters

Graph. - $f(x)=-2(x-4)^{2}+2$ $Graph. - f(x)=-2(x-4)^{2}+2$

Graph the function. - $f(x)=|3 x-5|$

Give the equation of the horizontal asymptote of the rational function. - $g(x)=\frac{x+2}{x^{2}-2}$

Graph the parabola. - $f(x)=2 x^{2}-2 x+2$ $Graph the parabola. - f(x)=2 x^{2}-2 x+2$

Evaluate the function. -Given that $f(x)=\sqrt{x}+\frac{3}{1-x}$ , find $f(3.8)$ .

For the given function, find $\frac{f(x+h)-f(x)}{h}$ . - $2-9 x$

Evaluate the function. -Given that $f(x)=\sqrt{x+5}$ , find $f(-3)$

Give the equation of the vertical asymptote(s) of the rational function. - $F(x)=\frac{x-9}{x^{2}+5}$

Give the equation of the vertical asymptote(s) of the rational function. - $g(x)=\frac{x^{2}+8 x-4}{x^{3}-3 x^{2}+2 x}$

Graph the linear function. - $h(x)=6 x$

Find the $x$ and $y$ intercepts. If no $x$ intercepts exist, state so. - $f(x)=x^{2}+20 x+100$

State the domain of the given function. - $f(x)=9 x^{2}+9 x-9$

Evaluate the function. -Determine $g(a-1)$ when $g(x)=2 x-3$ .

Evaluate the function. -Find $f(-2)$ when $f(x)=2 x^{2}-2 x+6$