Exam 3: Functions and Graphs
Exam 1: Algebra and Equations409 Questions
Exam 2: Graphs, Lines, and Inequalities255 Questions
Exam 3: Functions and Graphs323 Questions
Exam 4: Exponential and Logarithmic Functions192 Questions
Exam 5: Mathematics of Finance183 Questions
Exam 6: Systems of Linear Equations and Matrices215 Questions
Exam 7: Linear Programming203 Questions
Exam 8: Sets and Probability240 Questions
Exam 9: Counting, Probability Distributions, and Further Topics in Probability210 Questions
Exam 10: Introduction to Statistics169 Questions
Exam 11: Differential Calculus342 Questions
Exam 12: Applications of the Derivative220 Questions
Exam 13: Integral Calculus227 Questions
Exam 14: Multivariate Calculus152 Questions
Select questions type
Give the equation of the vertical asymptote(s) of the rational function.
-
(Multiple Choice)
4.8/5  (34)
(34)
Give the equation of the vertical asymptote(s) of the rational function.
-
(Multiple Choice)
4.9/5  (24)
(24)
Use a graphing calculator to find a viewing window that shows a complete graph of the given polynomial function(that is, a graph that includes all the peaks and valleys and indicates how the curve moves away frem the axis at thefar left and far right.) There are many possible correct answers.
-
(Essay)
4.9/5  (25)
(25)
Use a graphing calculator to find a viewing window that shows a complete graph of the given polynomial function(that is, a graph that includes all the peaks and valleys and indicates how the curve moves away frem the axis at thefar left and far right.) There are many possible correct answers.
-
(Essay)
4.8/5  (34)
(34)
Solve the problem.
-At a manufacturing plant, the total cost (in dollars) to produce items is
.
What is the total cost to produce 2900 items?
(Multiple Choice)
4.7/5  (33)
(33)
Solve the problem.
-An open-top box is to be made by cutting small identical squares from each corner of a 12-by-12-in. sheet of tin and bending up the sides. If each corner square is inches on a side, the volume of the box (in in. ) is given by:
By sketching the graph of , estimate what values of result in a box with a volume greater than .
(Multiple Choice)
4.7/5  (28)
(28)
Solve the problem.
-The graph of a function is given below. Tell whether the graph could possibly be the graph of a polynomial function. If it could be the graph of a polynomial function, tell which of the following are possible degrees for the polynomial function: 3, 4, 5, 6 .
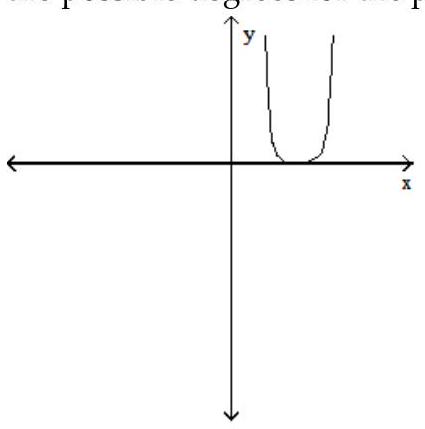
(Multiple Choice)
4.8/5  (39)
(39)
Solve the problem.
-Bob owns a watch repair shop. He has found that the cost of operating his shop is given by , where is cost and is the number of watches repaired. How many watches must he repair to have the lowest cost?
(Multiple Choice)
4.9/5  (33)
(33)
Solve the problem.
-An oil refinery can produce gasoline, heating oil, or a combination of the two. The product exchange function for gasoline, , and heating oil, , in thousands of gallons per week is:
By sketching the quadrant I portion of the graph of this function, estimate the maximum quantity of each product that can be produced per week.
(Multiple Choice)
4.9/5  (27)
(27)
Graph the function.
-A roller blade rental store charges plus per hour or fraction of an hour. Graph the ordered pairs (hours, cost).
(Multiple Choice)
4.8/5  (31)
(31)
Write the word or phrase that best completes each statement or answers the question.
-The table shows the winning time for the women's 200 -meter run at seven consecutive summer Olympic Games.
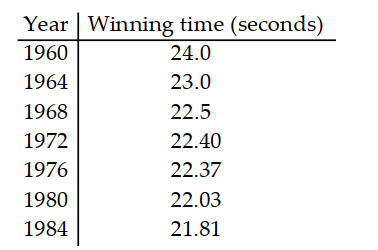 Let correspond to 1960 and let be the winning time for the women's 200 -meter run at the Olympics in year . Using the points and , the following linear function is obtained to model the data: . According to this model, will the winning time ever reach 10 seconds? If so, when? Do you think that it makes sense to use the model to make predictions for future years?
Let correspond to 1960 and let be the winning time for the women's 200 -meter run at the Olympics in year . Using the points and , the following linear function is obtained to model the data: . According to this model, will the winning time ever reach 10 seconds? If so, when? Do you think that it makes sense to use the model to make predictions for future years?
(Essay)
4.8/5  (34)
(34)
Use the vertical line test to determine if the graph is a graph of a function.
-
(True/False)
4.8/5  (36)
(36)
Solve the problem.
-Suppose a cost-benefit model is given by , where is the cost in thousands of dollars for removing percent of a given pollutant. Find the cost of removing , to the nearest dollar.
(Multiple Choice)
4.7/5  (26)
(26)
Showing 301 - 320 of 323
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)