Exam 21: Modeling Using Linear Programming

A Singapore company manufactures 50-inch and 75-inch rear projection television sets.Each 50-inch set contributes $200 to profits and each 75-inch set contributes $475 to profits.The company has purchase commitments for 500 50-inch sets and 200 75-inch sets for the next month so they want to make at least that many.Although they think they can sell all the 50-inch sets that they could currently make, they do not think they can sell more than 375 75-inch sets.Their factory capacity allows them to make only 975 sets of both sizes total.They want to know how many of each type to make so as to maximize profits. a.What is the objective function for this LP problem? b.What are the constraints involving X1, assuming that X1 corresponds to 50-inch TV sets? c.What is the optimal solution point for this problem? d.What is the optimal value of the objective function?

A computer manufacturing company wants to develop a monthly plan for shipping finished products from three of its manufacturing facilities to three regional warehouses.It is thinking about using a transportation LP formulation to exactly match capacities and requirements.Data on transportation costs (in dollars per unit), capacities, and requirements are given below: 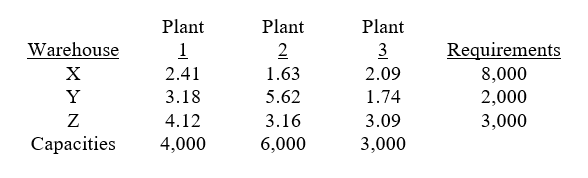a.How many variables are involved in the LP formulation? b.How many constraints are there in this problem? c.What is the constraint corresponding to plant 2?

If Activity C directly precedes both D and E on a project network and C can be crashed three (3) times in using linear programming,

For linear programming to work for project crashing decisions, a dummy activity is needed at the beginning of the project, with a duration of zero (0) time.

The constraint that requires the beginning inventory plus production minus sales to equal the ending inventory is called material-balance equation.

If R_m = increase in the total production level during Month 'm' compared to Month m-1 and D_m = decrease in the total production level during Month 'm' compared to Month m-1, which of the following statements can be correct?

$\sqrt{x}$ is linear since x is to the first power.

The normal-time project cost does not depend on what crashing decisions are made.As a result, total crash costs can be minimized for a linear programming objective.

A cement company has three factories that they identify as Alpha, Beta, and Gamma.They supply cement to three warehouses that they call X, Y, and Z.The company wants to determine how much cement should be shipped from each factory to each warehouse to minimize shipping costs.The cost to ship each 100-pound bag, along with warehouse requirements and factory capacities in bags are shown in the table below: 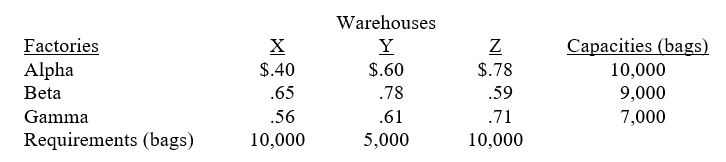a.How many decision variables are there in this problem? b.What is (are) the constraint(s) corresponding to Factory Alpha? c.How many constraints are required for this problem?

If for a given month overtime units ( O_t ) = under-time units ( U_t ),

The Pacific Computer Company makes two models of notebook personal computers: Model 410 with CD-ROM drive, and Model 540 with DVD drive.Profits on each model are $100 and $150, respectively.Weekly manufacturing data (in minutes) are given below: Manufacturing time available in department A for the coming week is 300 minutes, and for department B it is 420 minutes.Total time available during the week to assemble the fabricated units is 1600 minutes. a.What is the objective function if the company wants to maximize profits? b.What is the constraint corresponding to Department A assuming X1 corresponds to Model 410? c.What is the optimum solution point for this problem? d.What is the maximum possible total profit?

(a month's production) + (beginning inventory) - (ending inventory) - (number of lost sales for the month) =

What characterizes a linear function?

A small lumber company in the Southeast produces two types of pine boards used in home construction: 2x4s and 2x6s (dimensions in inches).They are attempting to determine how many of each to produce so as to minimize their costs on a per-minute basis.They have sales commitments to produce four 2x4s and two 2x6s per minute, but they think they shouldn't produce any more than eight 2x6s because of market demand.They are also trying to support the community by employing people.Thus they want to keep at least 12 men employed, but only need 2 men to produce each 2x4 and 1 person to produce each 2x6 per minute.It costs them $.50 to produce 2x4 and $.80 to produce a 2x6 per minute. a.What is the objective function for this LP problem? b.What is the constraint for the employment issue, assuming X1 corresponds to 2x4s? c.What is the optimal solution point for this problem? d.What is the optimal value of the objective function?

A clothing distributor has four warehouses which serve four large cities.Each warehouse has a monthly capacity of 5,000 blue jeans.They are considering using a transportation LP approach to match demand and capacity.The following table provides data on their shipping cost, capacity, and demand constraints on a per-month basis: 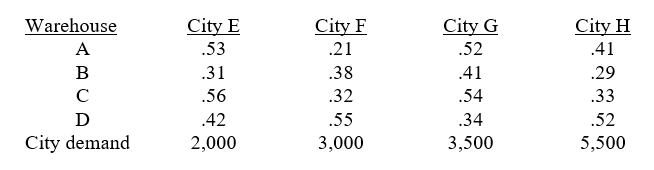a.How many variables are there in this formulation? b.How many constraints are involved in this problem? c.What is the constraint corresponding to City F?

ABC Products produces three products (A, B and C) on three machines.Machines 1 and 2 are available for 40 hours a week and Machine 3 is available for 60 hours a week.Profit contribution and standard production time in hours are given in the following table: 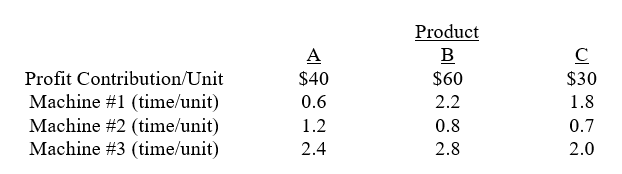Only one operator per machine is required on Machines #1 and #2.Two operators are required for Machine #3.Therefore, two hours of labor must be scheduled for each hour of Machine #3's time.To restate this requirement, two operators must be scheduled for each hour of Machine #3's operation, as well as one operator for each hour of Machine #1's operation and one operator for each hour of Machine #2's operation.A total of 110 labor hours is available for assignment to the three machines during the coming week.Other production requirements are that Product A cannot account for more than 40% of the units produced and that Product C must account for at least 25% of the units produced. a.Develop the constraint for the capacity limit of Machine #1. b.Develop the constraint for the capacity limit of Machine #3. c.Develop the constraint for the labor capacity limit. d.Develop the constraint for limiting Product A to no more than 40% of the units produced. e.Develop the constraint that ensures Product C accounts for at least 25% of the units produced.

Since price is usually set by market conditions, the blending problem use of linear programming attempts to meet demand at minimum cost.

Deciding on how much of each grade of gasoline to produce is an example of the linear programming model for blending applications.

Which of the following is generally correct regarding the transportation problem of linear programming?

Any particular combination of decision variables is referred to as a(n) ____.