Explore all topics
Start Free Trial
Need Help? Contact us
back arrowfull exam logo
search
ai logoChat with AI
Servicesarrow
Discoverarrow

Topics

Explore all topics
Discover more topics

Exam 12: Section 4: Vector-Valued Functions

arrow
Exam 1: Section 1: Preparation for Calculus16 Questionsadd course
Exam 1: Section 2: Preparation for Calculus26 Questionsadd course
Exam 1: Section 3: Preparation for Calculus23 Questionsadd course
Exam 1: Section 4: Preparation for Calculus16 Questionsadd course
Exam 1: Section 5: Preparation for Calculus25 Questionsadd course
Exam 1: Section 6: Preparation for Calculus8 Questionsadd course
Exam 2: Section 1: Limits and Their Properties10 Questionsadd course
Exam 2: Section 2: Limits and Their Properties14 Questionsadd course
Exam 2: Section 3: Limits and Their Properties25 Questionsadd course
Exam 2: Section 4: Limits and Their Properties20 Questionsadd course
Exam 2: Section 5 : Limits and Their Properties18 Questionsadd course
Exam 3: Section 1 : Differentiation20 Questionsadd course
Exam 3: Section 2: Differentiation25 Questionsadd course
Exam 3: Section 3: Differentiation26 Questionsadd course
Exam 3: Section 4 : Differentiation44 Questionsadd course
Exam 3: Section 5: Differentiation30 Questionsadd course
Exam 3: Section 6: Differentiation16 Questionsadd course
Exam 3: Section 7: Differentiation16 Questionsadd course
Exam 3: Section 8: Differentiation12 Questionsadd course
Exam 4: Section 1 : Applications of Differentiation19 Questionsadd course
Exam 4: Section 2: Applications of Differentiation17 Questionsadd course
Exam 4: Section 3: Applications of Differentiation17 Questionsadd course
Exam 4: Section 4: Applications of Differentiation26 Questionsadd course
Exam 4: Section 5: Applications of Differentiation23 Questionsadd course
Exam 4: Section 6: Applications of Differentiation22 Questionsadd course
Exam 4: Section 7: Applications of Differentiation15 Questionsadd course
Exam 4: Section 8: Applications of Differentiation16 Questionsadd course
Exam 4: Section 1: Integration19 Questionsadd course
Exam 4: Section 2: Integration17 Questionsadd course
Exam 4: Section 3: Integration19 Questionsadd course
Exam 4: Section 4: Integration18 Questionsadd course
Exam 4: Section 5: Integration31 Questionsadd course
Exam 4: Section 6: Integration18 Questionsadd course
Exam 4: Section 7: Integration27 Questionsadd course
Exam 4: Section 8: Integration16 Questionsadd course
Exam 4: Section 9: Integration20 Questionsadd course
Exam 6: Section 1: Differential Equations19 Questionsadd course
Exam 6: Section 2: Differential Equations25 Questionsadd course
Exam 6: Section 3: Differential Equations12 Questionsadd course
Exam 6: Section 4: Differential Equations14 Questionsadd course
Exam 6: Section 5: Differential Equations17 Questionsadd course
Exam 7: Section 1: Applications of Integration18 Questionsadd course
Exam 7: Section 2: Applications of Integration18 Questionsadd course
Exam 7: Section 3: Applications of Integration17 Questionsadd course
Exam 7: Section 4: Applications of Integration18 Questionsadd course
Exam 7: Section 5: Applications of Integration16 Questionsadd course
Exam 7: Section 6: Applications of Integration19 Questionsadd course
Exam 7: Section 7: Applications of Integration15 Questionsadd course
Exam 8: Section 1: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questionsadd course
Exam 8: Section 2: Integration Techniques, Lhôpitals Rule, and Improper Integrals18 Questionsadd course
Exam 8: Section 3: Integration Techniques, Lhôpitals Rule, and Improper Integrals20 Questionsadd course
Exam 8: Section 4: Integration Techniques, Lhôpitals Rule, and Improper Integrals19 Questionsadd course
Exam 8: Section 5: Integration Techniques, Lhôpitals Rule, and Improper Integrals14 Questionsadd course
Exam 8: Section 6: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questionsadd course
Exam 8: Section 7: Integration Techniques, Lhôpitals Rule, and Improper Integrals18 Questionsadd course
Exam 8: Section 8: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questionsadd course
Exam 9: Section 1: Infinite Series17 Questionsadd course
Exam 9: Section 2: Infinite Series23 Questionsadd course
Exam 9: Section 3: Infinite Series18 Questionsadd course
Exam 9: Section 4: Infinite Series21 Questionsadd course
Exam 9: Section 5: Infinite Series15 Questionsadd course
Exam 9: Section 6: Infinite Series21 Questionsadd course
Exam 9: Section 7: Infinite Series18 Questionsadd course
Exam 9: Section 8: Infinite Series18 Questionsadd course
Exam 9: Section 9: Infinite Series19 Questionsadd course
Exam 9: Section 10: Infinite Series16 Questionsadd course
Exam 10: Section 1: Conics, Parametric Equations, and Polar Coordinates26 Questionsadd course
Exam 10: Section 2: Conics, Parametric Equations, and Polar Coordinates17 Questionsadd course
Exam 10: Section 3: Conics, Parametric Equations, and Polar Coordinates22 Questionsadd course
Exam 10: Section 4: Conics, Parametric Equations, and Polar Coordinates15 Questionsadd course
Exam 10: Section 5: Conics, Parametric Equations, and Polar Coordinates18 Questionsadd course
Exam 10: Section 6: Conics, Parametric Equations, and Polar Coordinates19 Questionsadd course
Exam 11: Section 1: Vectors and the Geometry of Space20 Questionsadd course
Exam 11: Section 2: Vectors and the Geometry of Space21 Questionsadd course
Exam 11: Section 3: Vectors and the Geometry of Space18 Questionsadd course
Exam 11: Section 4: Vectors and the Geometry of Space18 Questionsadd course
Exam 11: Section 5: Vectors and the Geometry of Space21 Questionsadd course
Exam 11: Section 6: Vectors and the Geometry of Space20 Questionsadd course
Exam 11: Section 7: Vectors and the Geometry of Space19 Questionsadd course
Exam 12: Section 1: Vector-Valued Functions21 Questionsadd course
Exam 12: Section 2: Vector-Valued Functions24 Questionsadd course
Exam 12: Section 3: Vector-Valued Functions18 Questionsadd course
Exam 12: Section 4: Vector-Valued Functions20 Questionsadd course
Exam 12: Section 5: Vector-Valued Functions19 Questionsadd course
Exam 13: Section 1: Functions of Several Variables19 Questionsadd course
Exam 13: Section 2: Functions of Several Variables22 Questionsadd course
Exam 13: Section 3: Functions of Several Variables23 Questionsadd course
Exam 13: Section 4: Functions of Several Variables17 Questionsadd course
Exam 13: Section 6: Functions of Several Variables20 Questionsadd course
Exam 13: Section 7: Functions of Several Variables20 Questionsadd course
Exam 13: Section 8: Functions of Several Variables20 Questionsadd course
Exam 13: Section 9: Functions of Several Variables17 Questionsadd course
Exam 13: Section 10: Functions of Several Variables18 Questionsadd course
Exam 14: Section 1: Multiple Integration20 Questionsadd course
Exam 14: Section 2: Multiple Integration19 Questionsadd course
Exam 14: Section 3: Multiple Integration20 Questionsadd course
Exam 14: Section 4: Multiple Integration18 Questionsadd course
Exam 14: Section 5: Multiple Integration18 Questionsadd course
Exam 14: Section 6: Multiple Integration19 Questionsadd course
Exam 14: Section 7: Multiple Integration19 Questionsadd course
Exam 14: Section 8: Multiple Integration19 Questionsadd course
Exam 15: Section 1: Vector Analysis21 Questionsadd course
Exam 15: Section 2: Vector Analysis18 Questionsadd course
Exam 15: Section 3: Vector Analysis18 Questionsadd course
Exam 15: Section 4: Vector Analysis18 Questionsadd course
Exam 15: Section 5: Vector Analysis21 Questionsadd course
Exam 15: Section 6: Vector Analysis18 Questionsadd course
Exam 15: Section 7: Vector Analysis18 Questionsadd course
Exam 15: Section 8: Vector Analysis17 Questionsadd course
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags
search iconSearch Question
flashcardsStudy Flashcards
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags

Because of a storm, ground controllers instruct the pilot of a plane flying at an altitude of 1.6 miles to make a Because of a storm, ground controllers instruct the pilot of a plane flying at an altitude of 1.6 miles to make a turn and climb to an altitude of 1.8 miles. The model for the path of the plane during this maneuver is where t is the time in hours and is the distance in miles. Determine the speed of the plane. Round your answer to the nearest integer. turn and climb to an altitude of 1.8 miles. The model for the path of the plane during this maneuver is Because of a storm, ground controllers instruct the pilot of a plane flying at an altitude of 1.6 miles to make a turn and climb to an altitude of 1.8 miles. The model for the path of the plane during this maneuver is where t is the time in hours and is the distance in miles. Determine the speed of the plane. Round your answer to the nearest integer. where t is the time in hours and Because of a storm, ground controllers instruct the pilot of a plane flying at an altitude of 1.6 miles to make a 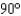turn and climb to an altitude of 1.8 miles. The model for the path of the plane during this maneuver is 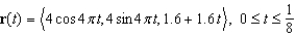where t is the time in hours and is the distance in miles. Determine the speed of the plane. Round your answer to the nearest integer. is the distance in miles. Determine the speed of the plane. Round your answer to the nearest integer.

Free
(Multiple Choice)
4.9/5
(34)
Question 1
Correct Answer:
Verified

A

Find a set of parametric equations for the tangent line to the graph at Find a set of parametric equations for the tangent line to the graph at and use the equations for the line to approximate . and use the equations for the line to approximate Find a set of parametric equations for the tangent line to the graph at and use the equations for the line to approximate . . Find a set of parametric equations for the tangent line to the graph at 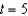and use the equations for the line to approximate .

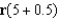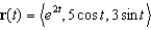
Free
(Multiple Choice)
4.8/5
(32)
Question 2
Correct Answer:
Verified

A

Find the principle unit normal vector to the curve given below at the specified point. Find the principle unit normal vector to the curve given below at the specified point.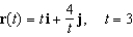

Free
(Multiple Choice)
4.9/5
(40)
Question 3
Correct Answer:
Verified

B

Verify that the space curves intersect at the given values of the parameter. Find the angle between the tangent vectors at the point of intersection. Verify that the space curves intersect at the given values of the parameter. Find the angle between the tangent vectors at the point of intersection. , , , Verify that the space curves intersect at the given values of the parameter. Find the angle between the tangent vectors at the point of intersection. , , Verify that the space curves intersect at the given values of the parameter. Find the angle between the tangent vectors at the point of intersection. , , , Verify that the space curves intersect at the given values of the parameter. Find the angle between the tangent vectors at the point of intersection. , ,

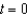
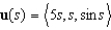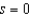
(Multiple Choice)
4.9/5
(39)
Question 4

Find Find at time for the space curve . Round your answer to three decimal places. at time Find at time for the space curve . Round your answer to three decimal places. for the space curve Find 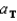at time 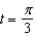for the space curve . Round your answer to three decimal places. . Round your answer to three decimal places.

(Multiple Choice)
4.9/5
(27)
Question 5

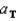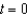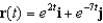Find Find at time for the plane curve . Round your answer to three decimal places. at time Find at time for the plane curve . Round your answer to three decimal places. for the plane curve Find at time for the plane curve . Round your answer to three decimal places. . Round your answer to three decimal places.

(Multiple Choice)
5.0/5
(34)
Question 6

Find the unit tangent vector to the curve given below at the specified point. Find the unit tangent vector to the curve given below at the specified point.

(Multiple Choice)
5.0/5
(26)
Question 7

Find Find at time for the space curve . at time Find at time for the space curve . for the space curve Find at time for the space curve . .

(Multiple Choice)
4.8/5
(39)
Question 8

Find the unit tangent vector Find the unit tangent vector for the line tangent to the space curve at point . for the line tangent to the space curve Find the unit tangent vector for the line tangent to the space curve at point . at point Find the unit tangent vector 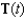for the line tangent to the space curve 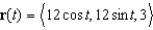at point . .

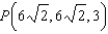(Multiple Choice)
4.8/5
(21)
Question 9

Find the principle unit normal vector to the curve given below at the specified point. Find the principle unit normal vector to the curve given below at the specified point.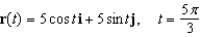

(Multiple Choice)
4.8/5
(40)
Question 10

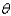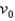Use the result, "the tangential and normal components of acceleration for a projectile fired at an angle Use the result, the tangential and normal components of acceleration for a projectile fired at an angle with the horizontal at an initial speed of are and respectively., to find the tangential component of acceleration for a projectile fired at an angle of with the horizontal at an initial speed of 152 feet per second. What is the component when the projectile is at its maximum height? 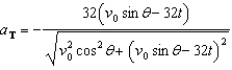with the horizontal at an initial speed of Use the result, the tangential and normal components of acceleration for a projectile fired at an angle with the horizontal at an initial speed of are and respectively., to find the tangential component of acceleration for a projectile fired at an angle of with the horizontal at an initial speed of 152 feet per second. What is the component when the projectile is at its maximum height? 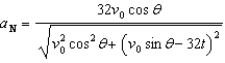are Use the result, the tangential and normal components of acceleration for a projectile fired at an angle with the horizontal at an initial speed of are and respectively., to find the tangential component of acceleration for a projectile fired at an angle of with the horizontal at an initial speed of 152 feet per second. What is the component when the projectile is at its maximum height? 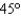and Use the result, the tangential and normal components of acceleration for a projectile fired at an angle with the horizontal at an initial speed of are and respectively., to find the tangential component of acceleration for a projectile fired at an angle of with the horizontal at an initial speed of 152 feet per second. What is the component when the projectile is at its maximum height? respectively.", to find the tangential component of acceleration for a projectile fired at an angle of Use the result, the tangential and normal components of acceleration for a projectile fired at an angle with the horizontal at an initial speed of are and respectively., to find the tangential component of acceleration for a projectile fired at an angle of with the horizontal at an initial speed of 152 feet per second. What is the component when the projectile is at its maximum height? with the horizontal at an initial speed of 152 feet per second. What is the component when the projectile is at its maximum height?

(Multiple Choice)
4.7/5
(38)
Question 11

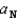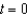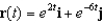Find Find at time for the plane curve . Round your answer to three decimal places. at time Find at time for the plane curve . Round your answer to three decimal places. for the plane curve Find at time for the plane curve . Round your answer to three decimal places. . Round your answer to three decimal places.

(Multiple Choice)
4.9/5
(38)
Question 12

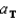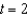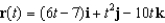Find Find at time for the space curve . Round your answer to three decimal places. at time Find at time for the space curve . Round your answer to three decimal places. for the space curve Find at time for the space curve . Round your answer to three decimal places. . Round your answer to three decimal places.

(Multiple Choice)
4.9/5
(39)
Question 13

Find the principle unit normal vector to the curve given below at the specified point. Find the principle unit normal vector to the curve given below at the specified point.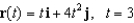

(Multiple Choice)
4.9/5
(34)
Question 14

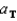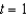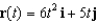Find Find at time for the plane curve . Round your answer to three decimal places. at time Find at time for the plane curve . Round your answer to three decimal places. for the plane curve Find at time for the plane curve . Round your answer to three decimal places. . Round your answer to three decimal places.

(Multiple Choice)
4.8/5
(28)
Question 15

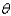Use the result, "the tangential and normal components of acceleration for a projectile fired at an angle Use the result, the tangential and normal components of acceleration for a projectile fired at an angle with the horizontal at an initial speed of are and respectively., to find the normal component of acceleration for a projectile fired at an angle of with the horizontal at an initial speed of 148 feet per second. What is the component when the projectile is at its maximum height? 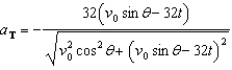with the horizontal at an initial speed of Use the result, the tangential and normal components of acceleration for a projectile fired at an angle with the horizontal at an initial speed of are and respectively., to find the normal component of acceleration for a projectile fired at an angle of with the horizontal at an initial speed of 148 feet per second. What is the component when the projectile is at its maximum height? are Use the result, the tangential and normal components of acceleration for a projectile fired at an angle with the horizontal at an initial speed of are and respectively., to find the normal component of acceleration for a projectile fired at an angle of with the horizontal at an initial speed of 148 feet per second. What is the component when the projectile is at its maximum height? and Use the result, the tangential and normal components of acceleration for a projectile fired at an angle with the horizontal at an initial speed of are and respectively., to find the normal component of acceleration for a projectile fired at an angle of with the horizontal at an initial speed of 148 feet per second. What is the component when the projectile is at its maximum height? respectively.", to find the normal component of acceleration for a projectile fired at an angle of Use the result, the tangential and normal components of acceleration for a projectile fired at an angle with the horizontal at an initial speed of are and 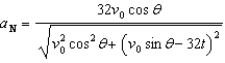respectively., to find the normal component of acceleration for a projectile fired at an angle of with the horizontal at an initial speed of 148 feet per second. What is the component when the projectile is at its maximum height? with the horizontal at an initial speed of 148 feet per second. What is the component when the projectile is at its maximum height?

(Multiple Choice)
4.8/5
(42)
Question 16

Find the unit tangent vector T(t) and then use it to find a set of parametric equations for the line tangent to the space curve given below at the given point. Find the unit tangent vector T(t) and then use it to find a set of parametric equations for the line tangent to the space curve given below at the given point.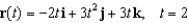

(Multiple Choice)
4.8/5
(40)
Question 17

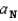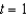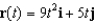Find Find at time for the plane curve . Round your answer to three decimal places. at time Find at time for the plane curve . Round your answer to three decimal places. for the plane curve Find at time for the plane curve . Round your answer to three decimal places. . Round your answer to three decimal places.

(Multiple Choice)
4.8/5
(38)
Question 18

Find the unit tangent vector to the curve given below at the specified point. Find the unit tangent vector to the curve given below at the specified point.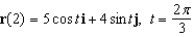

(Multiple Choice)
4.9/5
(30)
Question 19

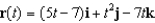Find Find at time for the space curve . Round your answer to three decimal places. at time Find at time for the space curve . Round your answer to three decimal places. for the space curve Find at time for the space curve . Round your answer to three decimal places. . Round your answer to three decimal places.

(Multiple Choice)
4.8/5
(30)
Question 20
close modal

Filters

  • Essay(0)
  • Multiple Choice(0)
  • Short Answer(0)
  • True False(0)
  • Matching(0)