Chat with AIExam 6: Section 5: Differential Equations
Exam 1: Section 1: Preparation for Calculus16 Questions
Exam 1: Section 2: Preparation for Calculus26 Questions
Exam 1: Section 3: Preparation for Calculus23 Questions
Exam 1: Section 4: Preparation for Calculus16 Questions
Exam 1: Section 5: Preparation for Calculus25 Questions
Exam 1: Section 6: Preparation for Calculus8 Questions
Exam 2: Section 1: Limits and Their Properties10 Questions
Exam 2: Section 2: Limits and Their Properties14 Questions
Exam 2: Section 3: Limits and Their Properties25 Questions
Exam 2: Section 4: Limits and Their Properties20 Questions
Exam 2: Section 5 : Limits and Their Properties18 Questions
Exam 3: Section 1 : Differentiation20 Questions
Exam 3: Section 2: Differentiation25 Questions
Exam 3: Section 3: Differentiation26 Questions
Exam 3: Section 4 : Differentiation44 Questions
Exam 3: Section 5: Differentiation30 Questions
Exam 3: Section 6: Differentiation16 Questions
Exam 3: Section 7: Differentiation16 Questions
Exam 3: Section 8: Differentiation12 Questions
Exam 4: Section 1 : Applications of Differentiation19 Questions
Exam 4: Section 2: Applications of Differentiation17 Questions
Exam 4: Section 3: Applications of Differentiation17 Questions
Exam 4: Section 4: Applications of Differentiation26 Questions
Exam 4: Section 5: Applications of Differentiation23 Questions
Exam 4: Section 6: Applications of Differentiation22 Questions
Exam 4: Section 7: Applications of Differentiation15 Questions
Exam 4: Section 8: Applications of Differentiation16 Questions
Exam 4: Section 1: Integration19 Questions
Exam 4: Section 2: Integration17 Questions
Exam 4: Section 3: Integration19 Questions
Exam 4: Section 4: Integration18 Questions
Exam 4: Section 5: Integration31 Questions
Exam 4: Section 6: Integration18 Questions
Exam 4: Section 7: Integration27 Questions
Exam 4: Section 8: Integration16 Questions
Exam 4: Section 9: Integration20 Questions
Exam 6: Section 1: Differential Equations19 Questions
Exam 6: Section 2: Differential Equations25 Questions
Exam 6: Section 3: Differential Equations12 Questions
Exam 6: Section 4: Differential Equations14 Questions
Exam 6: Section 5: Differential Equations17 Questions
Exam 7: Section 1: Applications of Integration18 Questions
Exam 7: Section 2: Applications of Integration18 Questions
Exam 7: Section 3: Applications of Integration17 Questions
Exam 7: Section 4: Applications of Integration18 Questions
Exam 7: Section 5: Applications of Integration16 Questions
Exam 7: Section 6: Applications of Integration19 Questions
Exam 7: Section 7: Applications of Integration15 Questions
Exam 8: Section 1: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questions
Exam 8: Section 2: Integration Techniques, Lhôpitals Rule, and Improper Integrals18 Questions
Exam 8: Section 3: Integration Techniques, Lhôpitals Rule, and Improper Integrals20 Questions
Exam 8: Section 4: Integration Techniques, Lhôpitals Rule, and Improper Integrals19 Questions
Exam 8: Section 5: Integration Techniques, Lhôpitals Rule, and Improper Integrals14 Questions
Exam 8: Section 6: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questions
Exam 8: Section 7: Integration Techniques, Lhôpitals Rule, and Improper Integrals18 Questions
Exam 8: Section 8: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questions
Exam 9: Section 1: Infinite Series17 Questions
Exam 9: Section 2: Infinite Series23 Questions
Exam 9: Section 3: Infinite Series18 Questions
Exam 9: Section 4: Infinite Series21 Questions
Exam 9: Section 5: Infinite Series15 Questions
Exam 9: Section 6: Infinite Series21 Questions
Exam 9: Section 7: Infinite Series18 Questions
Exam 9: Section 8: Infinite Series18 Questions
Exam 9: Section 9: Infinite Series19 Questions
Exam 9: Section 10: Infinite Series16 Questions
Exam 10: Section 1: Conics, Parametric Equations, and Polar Coordinates26 Questions
Exam 10: Section 2: Conics, Parametric Equations, and Polar Coordinates17 Questions
Exam 10: Section 3: Conics, Parametric Equations, and Polar Coordinates22 Questions
Exam 10: Section 4: Conics, Parametric Equations, and Polar Coordinates15 Questions
Exam 10: Section 5: Conics, Parametric Equations, and Polar Coordinates18 Questions
Exam 10: Section 6: Conics, Parametric Equations, and Polar Coordinates19 Questions
Exam 11: Section 1: Vectors and the Geometry of Space20 Questions
Exam 11: Section 2: Vectors and the Geometry of Space21 Questions
Exam 11: Section 3: Vectors and the Geometry of Space18 Questions
Exam 11: Section 4: Vectors and the Geometry of Space18 Questions
Exam 11: Section 5: Vectors and the Geometry of Space21 Questions
Exam 11: Section 6: Vectors and the Geometry of Space20 Questions
Exam 11: Section 7: Vectors and the Geometry of Space19 Questions
Exam 12: Section 1: Vector-Valued Functions21 Questions
Exam 12: Section 2: Vector-Valued Functions24 Questions
Exam 12: Section 3: Vector-Valued Functions18 Questions
Exam 12: Section 4: Vector-Valued Functions20 Questions
Exam 12: Section 5: Vector-Valued Functions19 Questions
Exam 13: Section 1: Functions of Several Variables19 Questions
Exam 13: Section 2: Functions of Several Variables22 Questions
Exam 13: Section 3: Functions of Several Variables23 Questions
Exam 13: Section 4: Functions of Several Variables17 Questions
Exam 13: Section 6: Functions of Several Variables20 Questions
Exam 13: Section 7: Functions of Several Variables20 Questions
Exam 13: Section 8: Functions of Several Variables20 Questions
Exam 13: Section 9: Functions of Several Variables17 Questions
Exam 13: Section 10: Functions of Several Variables18 Questions
Exam 14: Section 1: Multiple Integration20 Questions
Exam 14: Section 2: Multiple Integration19 Questions
Exam 14: Section 3: Multiple Integration20 Questions
Exam 14: Section 4: Multiple Integration18 Questions
Exam 14: Section 5: Multiple Integration18 Questions
Exam 14: Section 6: Multiple Integration19 Questions
Exam 14: Section 7: Multiple Integration19 Questions
Exam 14: Section 8: Multiple Integration19 Questions
Exam 15: Section 1: Vector Analysis21 Questions
Exam 15: Section 2: Vector Analysis18 Questions
Exam 15: Section 3: Vector Analysis18 Questions
Exam 15: Section 4: Vector Analysis18 Questions
Exam 15: Section 5: Vector Analysis21 Questions
Exam 15: Section 6: Vector Analysis18 Questions
Exam 15: Section 7: Vector Analysis18 Questions
Exam 15: Section 8: Vector Analysis17 Questions
Select questions type
A 200-gallon tank is half full of distilled water. At time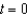 
, a solution containing 0.5 pound of concentrate per gallon enters the tank at the rate of 5 gallons per minute, and the well-stirred mixture is withdrawn at the rate of 3 gallons per minute. At the time the tank is full, how many pounds of concentrate will it contain? Round your answer to two decimal places.
, a solution containing 0.5 pound of concentrate per gallon enters the tank at the rate of 5 gallons per minute, and the well-stirred mixture is withdrawn at the rate of 3 gallons per minute. At the time the tank is full, how many pounds of concentrate will it contain? Round your answer to two decimal places.
Free
(Multiple Choice)
4.9/5 
(38)
(38) Correct Answer:
Verified
VerifiedE
Find the particular solution of the differential equation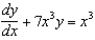 
passing through the point 
.
passing through the point 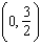.Free
(Multiple Choice)
4.8/5 (38)
(38) Correct Answer:Verified
VerifiedD
A 300-gallon tank is half full of distilled water. At time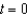 
, a solution containing 0.5 pound of concentrate per gallon enters the tank at the rate of 8 gallons per minute, and the well-stirred mixture is withdrawn at the rate of 6 gallons per minute. At what time will the tank be full?
, a solution containing 0.5 pound of concentrate per gallon enters the tank at the rate of 8 gallons per minute, and the well-stirred mixture is withdrawn at the rate of 6 gallons per minute. At what time will the tank be full?Free
(Multiple Choice)
4.8/5 (43)
(43) Correct Answer:Verified
VerifiedC
A 200-gallon tank is full of a solution containing 25 pounds of concentrate. Starting at time 
, distilled water is added to the tank at a rate of 10 gallons per minute, and the well-stirred solution is withdrawn at the same rate. Find the time at which the amount of concentrate in the tank reaches 15 pounds. Round your answer to one decimal place.
, distilled water is added to the tank at a rate of 10 gallons per minute, and the well-stirred solution is withdrawn at the same rate. Find the time at which the amount of concentrate in the tank reaches 15 pounds. Round your answer to one decimal place. (Multiple Choice)
4.7/5 (28)
(28) 
.Find the particular solution of the differential equation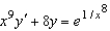 
that satisfies the initial condition 
.
that satisfies the initial condition 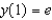. (Multiple Choice)
4.7/5 (32)
(32) 
Find the particular solution of the differential equation 
that satisfies the boundary condition 
.
that satisfies the boundary condition 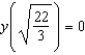. (Multiple Choice)
4.8/5 (42)
(42) A 300-gallon tank is full of a solution containing 35 pounds of concentrate. Starting at time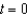 
distilled water is added to the tank at a rate of 30 gallons per minute, and the well-stirred solution is withdrawn at the same rate. Find the amount of concentrate Q in the solution as a function of t.
distilled water is added to the tank at a rate of 30 gallons per minute, and the well-stirred solution is withdrawn at the same rate. Find the amount of concentrate Q in the solution as a function of t. (Multiple Choice)
4.9/5 (24)
(24) Find the particular solution of the differential equation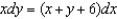 
that satisfies the boundary condition 
.
that satisfies the boundary condition 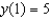. (Multiple Choice)
4.9/5 (34)
(34) A 100-gallon tank is full of a solution containing 25 pounds of concentrate. Starting at time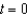 
, distilled water is added to the tank at a rate of 10 gallons per minute, and the well-stirred solution is withdrawn at the same rate. Find the quantity of the concentrate in the solution as 
.
, distilled water is added to the tank at a rate of 10 gallons per minute, and the well-stirred solution is withdrawn at the same rate. Find the quantity of the concentrate in the solution as 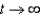. (Multiple Choice)
4.9/5 (27)
(27) Find the particular solution of the differential equation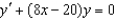 
that satisfies the boundary condition 
.
that satisfies the boundary condition 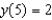. (Multiple Choice)
4.9/5 (34)
(34) Assume an object weighing 7 pounds is dropped from a height of 9,000 feet, where the air resistance is proportional to the velocity. Round numerical answers in your answer to two places. (i) Write the velocity as a function of time if the object's velocity after 6 seconds is 3.50 feet per second.
(ii) What is the limiting value of the velocity function?
(Multiple Choice)
4.8/5 (33)
(33) Use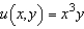 
as a integrating factor to find the general solution of the differential equation 
.
as a integrating factor to find the general solution of the differential equation 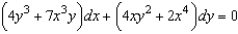. (Multiple Choice)
4.9/5 (39)
(39) 
Suppose an eight-pound object is dropped from a height of 5000 feet, where the air resistance is proportional to the velocity. Write the velocity as a function of time if its velocity after 7 seconds is approximately -75 feet per second. Use a graphing utility or a computer algebra system. Round numerical answers in your answer to four places.
(Multiple Choice)
4.8/5 (41)
(41) 
.
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)