Explore all topics
Start Free Trial
Need Help? Contact us
back arrowfull exam logo
search
ai logoChat with AI
Servicesarrow
Discoverarrow

Topics

Explore all topics
Discover more topics

Exam 4: Section 6: Integration

arrow
Exam 1: Section 1: Preparation for Calculus16 Questionsadd course
Exam 1: Section 2: Preparation for Calculus26 Questionsadd course
Exam 1: Section 3: Preparation for Calculus23 Questionsadd course
Exam 1: Section 4: Preparation for Calculus16 Questionsadd course
Exam 1: Section 5: Preparation for Calculus25 Questionsadd course
Exam 1: Section 6: Preparation for Calculus8 Questionsadd course
Exam 2: Section 1: Limits and Their Properties10 Questionsadd course
Exam 2: Section 2: Limits and Their Properties14 Questionsadd course
Exam 2: Section 3: Limits and Their Properties25 Questionsadd course
Exam 2: Section 4: Limits and Their Properties20 Questionsadd course
Exam 2: Section 5 : Limits and Their Properties18 Questionsadd course
Exam 3: Section 1 : Differentiation20 Questionsadd course
Exam 3: Section 2: Differentiation25 Questionsadd course
Exam 3: Section 3: Differentiation26 Questionsadd course
Exam 3: Section 4 : Differentiation44 Questionsadd course
Exam 3: Section 5: Differentiation30 Questionsadd course
Exam 3: Section 6: Differentiation16 Questionsadd course
Exam 3: Section 7: Differentiation16 Questionsadd course
Exam 3: Section 8: Differentiation12 Questionsadd course
Exam 4: Section 1 : Applications of Differentiation19 Questionsadd course
Exam 4: Section 2: Applications of Differentiation17 Questionsadd course
Exam 4: Section 3: Applications of Differentiation17 Questionsadd course
Exam 4: Section 4: Applications of Differentiation26 Questionsadd course
Exam 4: Section 5: Applications of Differentiation23 Questionsadd course
Exam 4: Section 6: Applications of Differentiation22 Questionsadd course
Exam 4: Section 7: Applications of Differentiation15 Questionsadd course
Exam 4: Section 8: Applications of Differentiation16 Questionsadd course
Exam 4: Section 1: Integration19 Questionsadd course
Exam 4: Section 2: Integration17 Questionsadd course
Exam 4: Section 3: Integration19 Questionsadd course
Exam 4: Section 4: Integration18 Questionsadd course
Exam 4: Section 5: Integration31 Questionsadd course
Exam 4: Section 6: Integration18 Questionsadd course
Exam 4: Section 7: Integration27 Questionsadd course
Exam 4: Section 8: Integration16 Questionsadd course
Exam 4: Section 9: Integration20 Questionsadd course
Exam 6: Section 1: Differential Equations19 Questionsadd course
Exam 6: Section 2: Differential Equations25 Questionsadd course
Exam 6: Section 3: Differential Equations12 Questionsadd course
Exam 6: Section 4: Differential Equations14 Questionsadd course
Exam 6: Section 5: Differential Equations17 Questionsadd course
Exam 7: Section 1: Applications of Integration18 Questionsadd course
Exam 7: Section 2: Applications of Integration18 Questionsadd course
Exam 7: Section 3: Applications of Integration17 Questionsadd course
Exam 7: Section 4: Applications of Integration18 Questionsadd course
Exam 7: Section 5: Applications of Integration16 Questionsadd course
Exam 7: Section 6: Applications of Integration19 Questionsadd course
Exam 7: Section 7: Applications of Integration15 Questionsadd course
Exam 8: Section 1: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questionsadd course
Exam 8: Section 2: Integration Techniques, Lhôpitals Rule, and Improper Integrals18 Questionsadd course
Exam 8: Section 3: Integration Techniques, Lhôpitals Rule, and Improper Integrals20 Questionsadd course
Exam 8: Section 4: Integration Techniques, Lhôpitals Rule, and Improper Integrals19 Questionsadd course
Exam 8: Section 5: Integration Techniques, Lhôpitals Rule, and Improper Integrals14 Questionsadd course
Exam 8: Section 6: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questionsadd course
Exam 8: Section 7: Integration Techniques, Lhôpitals Rule, and Improper Integrals18 Questionsadd course
Exam 8: Section 8: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questionsadd course
Exam 9: Section 1: Infinite Series17 Questionsadd course
Exam 9: Section 2: Infinite Series23 Questionsadd course
Exam 9: Section 3: Infinite Series18 Questionsadd course
Exam 9: Section 4: Infinite Series21 Questionsadd course
Exam 9: Section 5: Infinite Series15 Questionsadd course
Exam 9: Section 6: Infinite Series21 Questionsadd course
Exam 9: Section 7: Infinite Series18 Questionsadd course
Exam 9: Section 8: Infinite Series18 Questionsadd course
Exam 9: Section 9: Infinite Series19 Questionsadd course
Exam 9: Section 10: Infinite Series16 Questionsadd course
Exam 10: Section 1: Conics, Parametric Equations, and Polar Coordinates26 Questionsadd course
Exam 10: Section 2: Conics, Parametric Equations, and Polar Coordinates17 Questionsadd course
Exam 10: Section 3: Conics, Parametric Equations, and Polar Coordinates22 Questionsadd course
Exam 10: Section 4: Conics, Parametric Equations, and Polar Coordinates15 Questionsadd course
Exam 10: Section 5: Conics, Parametric Equations, and Polar Coordinates18 Questionsadd course
Exam 10: Section 6: Conics, Parametric Equations, and Polar Coordinates19 Questionsadd course
Exam 11: Section 1: Vectors and the Geometry of Space20 Questionsadd course
Exam 11: Section 2: Vectors and the Geometry of Space21 Questionsadd course
Exam 11: Section 3: Vectors and the Geometry of Space18 Questionsadd course
Exam 11: Section 4: Vectors and the Geometry of Space18 Questionsadd course
Exam 11: Section 5: Vectors and the Geometry of Space21 Questionsadd course
Exam 11: Section 6: Vectors and the Geometry of Space20 Questionsadd course
Exam 11: Section 7: Vectors and the Geometry of Space19 Questionsadd course
Exam 12: Section 1: Vector-Valued Functions21 Questionsadd course
Exam 12: Section 2: Vector-Valued Functions24 Questionsadd course
Exam 12: Section 3: Vector-Valued Functions18 Questionsadd course
Exam 12: Section 4: Vector-Valued Functions20 Questionsadd course
Exam 12: Section 5: Vector-Valued Functions19 Questionsadd course
Exam 13: Section 1: Functions of Several Variables19 Questionsadd course
Exam 13: Section 2: Functions of Several Variables22 Questionsadd course
Exam 13: Section 3: Functions of Several Variables23 Questionsadd course
Exam 13: Section 4: Functions of Several Variables17 Questionsadd course
Exam 13: Section 6: Functions of Several Variables20 Questionsadd course
Exam 13: Section 7: Functions of Several Variables20 Questionsadd course
Exam 13: Section 8: Functions of Several Variables20 Questionsadd course
Exam 13: Section 9: Functions of Several Variables17 Questionsadd course
Exam 13: Section 10: Functions of Several Variables18 Questionsadd course
Exam 14: Section 1: Multiple Integration20 Questionsadd course
Exam 14: Section 2: Multiple Integration19 Questionsadd course
Exam 14: Section 3: Multiple Integration20 Questionsadd course
Exam 14: Section 4: Multiple Integration18 Questionsadd course
Exam 14: Section 5: Multiple Integration18 Questionsadd course
Exam 14: Section 6: Multiple Integration19 Questionsadd course
Exam 14: Section 7: Multiple Integration19 Questionsadd course
Exam 14: Section 8: Multiple Integration19 Questionsadd course
Exam 15: Section 1: Vector Analysis21 Questionsadd course
Exam 15: Section 2: Vector Analysis18 Questionsadd course
Exam 15: Section 3: Vector Analysis18 Questionsadd course
Exam 15: Section 4: Vector Analysis18 Questionsadd course
Exam 15: Section 5: Vector Analysis21 Questionsadd course
Exam 15: Section 6: Vector Analysis18 Questionsadd course
Exam 15: Section 7: Vector Analysis18 Questionsadd course
Exam 15: Section 8: Vector Analysis17 Questionsadd course
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags
search iconSearch Question
flashcardsStudy Flashcards
Select questions type
  • Essay
  • Multiple Choice
  • Short Answer
  • True False
  • Matching
  • Select Tags

Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral. subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral. Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral.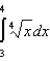

Free
(Multiple Choice)
5.0/5
(29)
Question 1
Correct Answer:
Verified

C

Find the smallest n such that the error estimate in the approximation of the definite integral 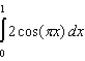Find the smallest n such that the error estimate in the approximation of the definite integral is less than 0.00001 using Simpson's Rule. is less than 0.00001 using Simpson's Rule.

Free
(Multiple Choice)
4.8/5
(36)
Question 2
Correct Answer:
Verified

E

Use Simpson's Rule to approximate the value of the definite integral Use Simpson's Rule to approximate the value of the definite integral 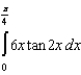with . Round your answer to four decimal places. with Use Simpson's Rule to approximate the value of the definite integral with . Round your answer to four decimal places. . Round your answer to four decimal places.

Free
(Multiple Choice)
4.9/5
(32)
Question 3
Correct Answer:
Verified

E

Use the Trapezoid Rule to approximate the value of the definite integral Use the Trapezoid Rule to approximate the value of the definite integral wth . Round your answer to four decimal places. wth Use the Trapezoid Rule to approximate the value of the definite integral wth . Round your answer to four decimal places. . Round your answer to four decimal places.

(Multiple Choice)
4.8/5
(36)
Question 4

Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral. subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral. Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using 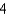subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral.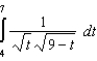

(Multiple Choice)
4.8/5
(37)
Question 5

Find the smallest n such that the error estimate from the error formula in the approximation of the definite integral Find the smallest n such that the error estimate from the error formula in the approximation of the definite integral is less than 0.00001 using the Trapezoidal Rule. is less than 0.00001 using the Trapezoidal Rule.

(Multiple Choice)
4.8/5
(34)
Question 6

Use Simpson's Rule to approximate the value of the definite integral Use Simpson's Rule to approximate the value of the definite integral 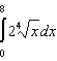with . Round your answer to four decimal places. with Use Simpson's Rule to approximate the value of the definite integral with . Round your answer to four decimal places. . Round your answer to four decimal places.

(Multiple Choice)
4.8/5
(33)
Question 7

Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral. subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral. Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral.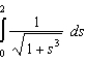

(Multiple Choice)
4.8/5
(26)
Question 8

Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral. subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral. Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral.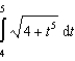

(Multiple Choice)
4.8/5
(39)
Question 9

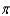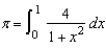Use Simpson's Rule with Use Simpson's Rule with to approximate using the equation . Round your answer to five decimal places. to approximate Use Simpson's Rule with to approximate using the equation . Round your answer to five decimal places. using the equation Use Simpson's Rule with to approximate using the equation . Round your answer to five decimal places. . Round your answer to five decimal places.

(Multiple Choice)
4.8/5
(29)
Question 10

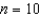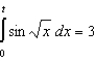Use a computer algebra system and Simpson's Rule with Use a computer algebra system and Simpson's Rule with to approximate t in the integral equation . Round your answer to three decimal places. to approximate t in the integral equation Use a computer algebra system and Simpson's Rule with to approximate t in the integral equation . Round your answer to three decimal places. . Round your answer to three decimal places.

(Multiple Choice)
4.8/5
(45)
Question 11

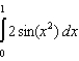Find the smallest n such that the error estimate in the approximation of the definite integral Find the smallest n such that the error estimate in the approximation of the definite integral is less than 0.00001 using the Trapezoidal Rule. Use a graphing utility to estimate the maximum of the absolute value of the second derivative. is less than 0.00001 using the Trapezoidal Rule. Use a graphing utility to estimate the maximum of the absolute value of the second derivative.

(Multiple Choice)
4.9/5
(29)
Question 12

Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral. subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral. Apply the Trapezoidal Rule and Simpson's Rule to approximate the value of the definite integral using 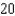subintervals. Round your answer to six decimal places and compare the result with the exact value of the definite integral.

(Multiple Choice)
4.9/5
(27)
Question 13

Estimate the error in using (a) the Trapezoidal Rule and (b) Simpson's Rule with Estimate the error in using (a) the Trapezoidal Rule and (b) Simpson's Rule with when approximating the following integral. when approximating the following integral. 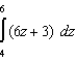Estimate the error in using (a) the Trapezoidal Rule and (b) Simpson's Rule with when approximating the following integral.

(Multiple Choice)
4.8/5
(30)
Question 14

Use the error formula to estimate the error in approximating the integral Use the error formula to estimate the error in approximating the integral 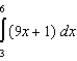with using Trapezoidal Rule. with Use the error formula to estimate the error in approximating the integral with using Trapezoidal Rule. using Trapezoidal Rule.

(Multiple Choice)
4.8/5
(39)
Question 15

Use the error formula to estimate the error in approximating the integral Use the error formula to estimate the error in approximating the integral 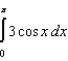with using Simpson's Rule. Round your answer to six decimal places. with Use the error formula to estimate the error in approximating the integral with using Simpson's Rule. Round your answer to six decimal places. using Simpson's Rule. Round your answer to six decimal places.

(Multiple Choice)
4.8/5
(30)
Question 16

Use the Trapezoidal Rule to approximate the value of the definite integral Use the Trapezoidal Rule to approximate the value of the definite integral with . Round your answer to four decimal places. with Use the Trapezoidal Rule to approximate the value of the definite integral with . Round your answer to four decimal places. . Round your answer to four decimal places.

(Multiple Choice)
4.8/5
(42)
Question 17

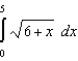Find the smallest n such that the error estimate in the approximation of the definite integral Find the smallest n such that the error estimate in the approximation of the definite integral is less than 0.00001 using Simpson's Rule. is less than 0.00001 using Simpson's Rule.

(Multiple Choice)
4.8/5
(36)
Question 18
close modal

Filters

  • Essay(0)
  • Multiple Choice(0)
  • Short Answer(0)
  • True False(0)
  • Matching(0)