Exam 3: Section 7: Differentiation
Exam 1: Section 1: Preparation for Calculus16 Questions
Exam 1: Section 2: Preparation for Calculus26 Questions
Exam 1: Section 3: Preparation for Calculus23 Questions
Exam 1: Section 4: Preparation for Calculus16 Questions
Exam 1: Section 5: Preparation for Calculus25 Questions
Exam 1: Section 6: Preparation for Calculus8 Questions
Exam 2: Section 1: Limits and Their Properties10 Questions
Exam 2: Section 2: Limits and Their Properties14 Questions
Exam 2: Section 3: Limits and Their Properties25 Questions
Exam 2: Section 4: Limits and Their Properties20 Questions
Exam 2: Section 5 : Limits and Their Properties18 Questions
Exam 3: Section 1 : Differentiation20 Questions
Exam 3: Section 2: Differentiation25 Questions
Exam 3: Section 3: Differentiation26 Questions
Exam 3: Section 4 : Differentiation44 Questions
Exam 3: Section 5: Differentiation30 Questions
Exam 3: Section 6: Differentiation16 Questions
Exam 3: Section 7: Differentiation16 Questions
Exam 3: Section 8: Differentiation12 Questions
Exam 4: Section 1 : Applications of Differentiation19 Questions
Exam 4: Section 2: Applications of Differentiation17 Questions
Exam 4: Section 3: Applications of Differentiation17 Questions
Exam 4: Section 4: Applications of Differentiation26 Questions
Exam 4: Section 5: Applications of Differentiation23 Questions
Exam 4: Section 6: Applications of Differentiation22 Questions
Exam 4: Section 7: Applications of Differentiation15 Questions
Exam 4: Section 8: Applications of Differentiation16 Questions
Exam 4: Section 1: Integration19 Questions
Exam 4: Section 2: Integration17 Questions
Exam 4: Section 3: Integration19 Questions
Exam 4: Section 4: Integration18 Questions
Exam 4: Section 5: Integration31 Questions
Exam 4: Section 6: Integration18 Questions
Exam 4: Section 7: Integration27 Questions
Exam 4: Section 8: Integration16 Questions
Exam 4: Section 9: Integration20 Questions
Exam 6: Section 1: Differential Equations19 Questions
Exam 6: Section 2: Differential Equations25 Questions
Exam 6: Section 3: Differential Equations12 Questions
Exam 6: Section 4: Differential Equations14 Questions
Exam 6: Section 5: Differential Equations17 Questions
Exam 7: Section 1: Applications of Integration18 Questions
Exam 7: Section 2: Applications of Integration18 Questions
Exam 7: Section 3: Applications of Integration17 Questions
Exam 7: Section 4: Applications of Integration18 Questions
Exam 7: Section 5: Applications of Integration16 Questions
Exam 7: Section 6: Applications of Integration19 Questions
Exam 7: Section 7: Applications of Integration15 Questions
Exam 8: Section 1: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questions
Exam 8: Section 2: Integration Techniques, Lhôpitals Rule, and Improper Integrals18 Questions
Exam 8: Section 3: Integration Techniques, Lhôpitals Rule, and Improper Integrals20 Questions
Exam 8: Section 4: Integration Techniques, Lhôpitals Rule, and Improper Integrals19 Questions
Exam 8: Section 5: Integration Techniques, Lhôpitals Rule, and Improper Integrals14 Questions
Exam 8: Section 6: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questions
Exam 8: Section 7: Integration Techniques, Lhôpitals Rule, and Improper Integrals18 Questions
Exam 8: Section 8: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questions
Exam 9: Section 1: Infinite Series17 Questions
Exam 9: Section 2: Infinite Series23 Questions
Exam 9: Section 3: Infinite Series18 Questions
Exam 9: Section 4: Infinite Series21 Questions
Exam 9: Section 5: Infinite Series15 Questions
Exam 9: Section 6: Infinite Series21 Questions
Exam 9: Section 7: Infinite Series18 Questions
Exam 9: Section 8: Infinite Series18 Questions
Exam 9: Section 9: Infinite Series19 Questions
Exam 9: Section 10: Infinite Series16 Questions
Exam 10: Section 1: Conics, Parametric Equations, and Polar Coordinates26 Questions
Exam 10: Section 2: Conics, Parametric Equations, and Polar Coordinates17 Questions
Exam 10: Section 3: Conics, Parametric Equations, and Polar Coordinates22 Questions
Exam 10: Section 4: Conics, Parametric Equations, and Polar Coordinates15 Questions
Exam 10: Section 5: Conics, Parametric Equations, and Polar Coordinates18 Questions
Exam 10: Section 6: Conics, Parametric Equations, and Polar Coordinates19 Questions
Exam 11: Section 1: Vectors and the Geometry of Space20 Questions
Exam 11: Section 2: Vectors and the Geometry of Space21 Questions
Exam 11: Section 3: Vectors and the Geometry of Space18 Questions
Exam 11: Section 4: Vectors and the Geometry of Space18 Questions
Exam 11: Section 5: Vectors and the Geometry of Space21 Questions
Exam 11: Section 6: Vectors and the Geometry of Space20 Questions
Exam 11: Section 7: Vectors and the Geometry of Space19 Questions
Exam 12: Section 1: Vector-Valued Functions21 Questions
Exam 12: Section 2: Vector-Valued Functions24 Questions
Exam 12: Section 3: Vector-Valued Functions18 Questions
Exam 12: Section 4: Vector-Valued Functions20 Questions
Exam 12: Section 5: Vector-Valued Functions19 Questions
Exam 13: Section 1: Functions of Several Variables19 Questions
Exam 13: Section 2: Functions of Several Variables22 Questions
Exam 13: Section 3: Functions of Several Variables23 Questions
Exam 13: Section 4: Functions of Several Variables17 Questions
Exam 13: Section 6: Functions of Several Variables20 Questions
Exam 13: Section 7: Functions of Several Variables20 Questions
Exam 13: Section 8: Functions of Several Variables20 Questions
Exam 13: Section 9: Functions of Several Variables17 Questions
Exam 13: Section 10: Functions of Several Variables18 Questions
Exam 14: Section 1: Multiple Integration20 Questions
Exam 14: Section 2: Multiple Integration19 Questions
Exam 14: Section 3: Multiple Integration20 Questions
Exam 14: Section 4: Multiple Integration18 Questions
Exam 14: Section 5: Multiple Integration18 Questions
Exam 14: Section 6: Multiple Integration19 Questions
Exam 14: Section 7: Multiple Integration19 Questions
Exam 14: Section 8: Multiple Integration19 Questions
Exam 15: Section 1: Vector Analysis21 Questions
Exam 15: Section 2: Vector Analysis18 Questions
Exam 15: Section 3: Vector Analysis18 Questions
Exam 15: Section 4: Vector Analysis18 Questions
Exam 15: Section 5: Vector Analysis21 Questions
Exam 15: Section 6: Vector Analysis18 Questions
Exam 15: Section 7: Vector Analysis18 Questions
Exam 15: Section 8: Vector Analysis17 Questions
Select questions type
The radius r of a sphere is increasing at a rate of 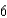 inches per minute. Find the rate of change of the volume when r =
inches per minute. Find the rate of change of the volume when r = 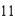 inches.
inches.
Free
(Multiple Choice)
4.8/5  (40)
(40)
Correct Answer:
C
A ladder 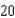 feet long is leaning against the wall of a house (see figure). The base of the ladder is pulled away from the wall at a rate of
feet long is leaning against the wall of a house (see figure). The base of the ladder is pulled away from the wall at a rate of 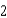 feet per second. How fast is the top of the ladder moving down the wall when its base is
feet per second. How fast is the top of the ladder moving down the wall when its base is 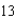 feet from the wall? Round your answer to two decimal places.
feet from the wall? Round your answer to two decimal places. 
Free
(Multiple Choice)
4.9/5  (35)
(35)
Correct Answer:
C
A ladder 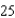 feet long is leaning against the wall of a house (see figure). The base of the ladder is pulled away from the wall at a rate of
feet long is leaning against the wall of a house (see figure). The base of the ladder is pulled away from the wall at a rate of 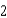 feet per second. Consider the triangle formed by the side of the house, the ladder, and the ground. Find the rate at which the area of the triangle is changed when the base of the ladder is
feet per second. Consider the triangle formed by the side of the house, the ladder, and the ground. Find the rate at which the area of the triangle is changed when the base of the ladder is 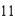 feet from the wall. Round your answer to two decimal places.
feet from the wall. Round your answer to two decimal places. 
Free
(Multiple Choice)
4.9/5  (35)
(35)
Correct Answer:
D
A ladder 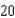 feet long is leaning against the wall of a house (see figure). The base of the ladder is pulled away from the wall at a rate of
feet long is leaning against the wall of a house (see figure). The base of the ladder is pulled away from the wall at a rate of 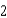 feet per second. Find the rate at which the angle between the ladder and the wall of the house is changing when the base of the ladder is
feet per second. Find the rate at which the angle between the ladder and the wall of the house is changing when the base of the ladder is 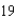 feet from the wall. Round your answer to three decimal places.
feet from the wall. Round your answer to three decimal places. 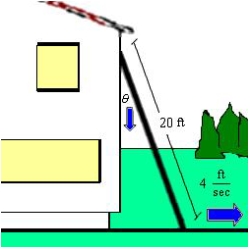
(Multiple Choice)
4.8/5  (36)
(36)
A conical tank (with vertex down) is 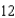 feet across the top and
feet across the top and 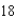 feet deep. If water is flowing into the tank at a rate of
feet deep. If water is flowing into the tank at a rate of 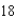 cubic feet per minute, find the rate of change of the depth of the water when the water is
cubic feet per minute, find the rate of change of the depth of the water when the water is  feet deep.
feet deep.
(Multiple Choice)
4.9/5  (33)
(33)
A spherical balloon is inflated with gas at the rate of 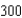 cubic centimeters per minute. How fast is the radius of the balloon increasing at the instant the radius is
cubic centimeters per minute. How fast is the radius of the balloon increasing at the instant the radius is 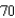 centimeters?
centimeters?
(Multiple Choice)
4.8/5  (29)
(29)
All edges of a cube are expanding at a rate of 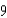 centimeters per second. How fast is the volume changing when each edge is
centimeters per second. How fast is the volume changing when each edge is 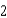 centimeters?
centimeters?
(Multiple Choice)
4.9/5  (37)
(37)
Assume that x and y are both differentiable functions of t. Find 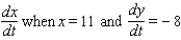 for the equation
for the equation 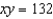 .
.
(Multiple Choice)
5.0/5  (30)
(30)
A point is moving along the graph of the function  such that
such that 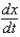 =
= 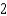 centimeters per second. Find
centimeters per second. Find 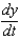 when
when 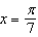 .
.
(Multiple Choice)
4.9/5  (34)
(34)
A point is moving along the graph of the function 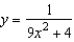 such that
such that 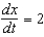 centimeters per second. Find
centimeters per second. Find  when x =
when x =  .
.
(Multiple Choice)
4.8/5  (30)
(30)
A man 6 feet tall walks at a rate of 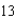 feet per second away from a light that is 15 feet above the ground (see figure). When he is
feet per second away from a light that is 15 feet above the ground (see figure). When he is  feet from the base of the light, at what rate is the length of his shadow changing?
feet from the base of the light, at what rate is the length of his shadow changing? 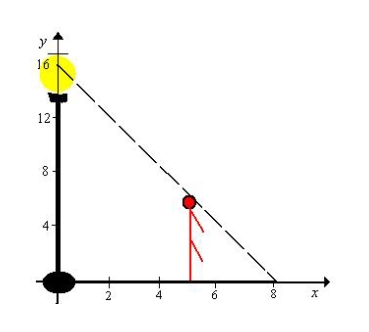
(Multiple Choice)
4.9/5  (35)
(35)
The radius, r, of a circle is decreasing at a rate of 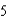 centimeters per minute. Find the rate of change of area, A, when the radius is
centimeters per minute. Find the rate of change of area, A, when the radius is 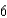 .
.
(Multiple Choice)
4.8/5  (38)
(38)
An airplane is flying in still air with an airspeed of 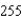 miles per hour. If it is climbing at an angle of
miles per hour. If it is climbing at an angle of 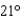 , find the rate at which it is gaining altitude. Round your answer to four decimal places.
, find the rate at which it is gaining altitude. Round your answer to four decimal places.
(Multiple Choice)
4.9/5  (29)
(29)
A man 6 feet tall walks at a rate of 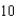 feet per second away from a light that is 15 feet above the ground (see figure). When he is
feet per second away from a light that is 15 feet above the ground (see figure). When he is 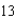 feet from the base of the light, at what rate is the tip of his shadow moving?
feet from the base of the light, at what rate is the tip of his shadow moving? 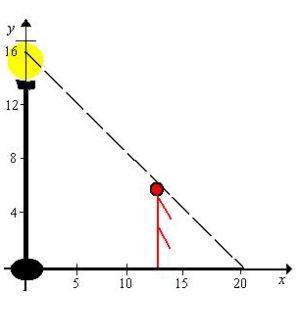
(Multiple Choice)
4.8/5  (38)
(38)
Assume that x and y are both differentiable functions of t . Find 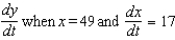 for the equation
for the equation 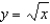 .
.
(Multiple Choice)
4.9/5  (42)
(42)
Find the rate of change of the distance  between the origin and a moving point on the graph of
between the origin and a moving point on the graph of 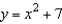 if
if 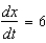 centimeters per second.
centimeters per second.
(Multiple Choice)
4.8/5  (34)
(34)
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)