Exam 6: Continuous Probability Distributions
Exam 1: Data and Statistics98 Questions
Exam 2: Descriptive Statistics: Tabular and Graphical Displays62 Questions
Exam 3: Descriptive Statistics: Numerical Measures173 Questions
Exam 4: Introduction to Probability138 Questions
Exam 5: Discrete Probability Distributions123 Questions
Exam 6: Continuous Probability Distributions174 Questions
Exam 7: Sampling and Sampling Distributions133 Questions
Exam 8: Interval Estimation137 Questions
Exam 9: Hypothesis Tests148 Questions
Exam 10: Inference About Means and Proportions With Two Populations121 Questions
Exam 11: Inferences About Population Variances90 Questions
Exam 12: Comparing Multiple Proportions, Test of Independence and Goodness of Fit90 Questions
Exam 13: Experimental Design and Analysis of Variance115 Questions
Exam 14: Simple Linear Regression146 Questions
Exam 15: Multiple Regression115 Questions
Exam 16: Regression Analysis: Model Building76 Questions
Exam 17: Time Series Analysis and Forecasting68 Questions
Exam 18: Nonparametric Methods81 Questions
Exam 19: Statistical Methods for Quality Control29 Questions
Exam 20: Index Numbers52 Questions
Exam 21: Decision Analysis on Website65 Questions
Exam 22: Sample Survey on Website63 Questions
Select questions type
Exhibit 6-2
The weight of football players is normally distributed with a mean of 200 pounds and a standard deviation of 25 pounds.
-Refer to Exhibit 6-2. The probability of a player weighing less than 250 pounds is
(Multiple Choice)
4.8/5  (29)
(29)
Exhibit 6-10
A professor at a local university noted that the exam grades of her students were normally distributed with a mean of 73 and a standard deviation of 11.
-Refer to Exhibit 6-10. Students who made 57.93 or lower on the exam failed the course. What percent of students failed the course?
(Multiple Choice)
4.9/5  (28)
(28)
For a standard normal distribution, the probability of obtaining a z value of less than 1.6 is
(Multiple Choice)
4.8/5  (29)
(29)
Z is a standard normal random variable. The P (-1.20 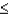 Z
Z  1.50) equals
1.50) equals
(Multiple Choice)
5.0/5  (36)
(36)
Consider a binomial probability experiment with n = 3 and p = 0.1. Then, the probability of x = 0 is
(Multiple Choice)
4.8/5  (21)
(21)
A manufacturing process produces items whose weights are normally distributed. It is known that 22.57% of all the items produced weigh between 100 grams up to the mean and 49.18% weigh from the mean up to 190 grams. Determine the mean and the standard deviation.
(Short Answer)
4.8/5  (31)
(31)
Given that Z is a standard normal random variable, what is the probability that -2.08 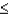 Z
Z 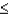 1.46?
1.46?
(Multiple Choice)
4.8/5  (41)
(41)
A major department store has determined that its customers charge an average of $500 per month, with a standard deviation of $80. Assume the amounts of charges are normally distributed.
a.What percentage of customers charges more than $380 per month?
b.What percentage of customers charges less than $340 per month?
c.What percentage of customers charges between $644 and $700 per month?
(Short Answer)
4.9/5  (30)
(30)
The salaries at a corporation are normally distributed with an average salary of $19,000 and a standard deviation of $4,000.
a.What is the probability that an employee will have a salary between $12,520 and $13,480?
b.What is the probability that an employee will have a salary more than $11,880?
c.What is the probability that an employee will have a salary less than $28,440?
(Short Answer)
4.9/5  (38)
(38)
The Mathematics part of the SAT scores of students at UTC are normally distributed with a mean of 500 and a standard deviation of 75.
a.If 2.28 percent of the students who had the highest scores received scholarships, what was the minimum score among those who received scholarships? Do not round your answer.
b.It is known that 6.3 percent of students who applied to UTC were not accepted. What is the highest score of those who were denied acceptance? Do not round your answer.
c.What percentage of students had scores between 575 and 650?
(Short Answer)
4.9/5  (33)
(33)
The random variable x is known to be uniformly distributed between 70 and 90. The probability of x having a value between 80 to 95 is
(Multiple Choice)
4.8/5  (42)
(42)
Exhibit 6-3
Consider the continuous random variable X, which has a uniform distribution over the interval from 20 to 28.
-Refer to Exhibit 6-3. The mean of X is
(Multiple Choice)
4.9/5  (30)
(30)
"DRUGS R US" is a large manufacturer of various kinds of liquid vitamins. The quality control department has noted that the bottles of vitamins marked 6 ounces vary in content with a standard deviation of 0.3 ounces. Assume the contents of the bottles are normally distributed.
a.What percentage of all bottles produced contains more than 6.51 ounces of vitamins?
b.What percentage of all bottles produced contains less than 5.415 ounces?
c.What percentage of bottles produced contains between 5.46 to 6.495 ounces?
d.Ninety-five percent of the bottles will contain at least how many ounces?
e.What percentage of the bottles contains between 6.3 and 6.6 ounces?
(Essay)
4.8/5  (31)
(31)
The time it takes a mechanic to change the oil in a car is exponentially distributed with a mean of 5 minutes.
a.What is the probability density function for the time it takes to change the oil?
b.What is the probability that it will take a mechanic less than 6 minutes to change the oil?
c.What is the probability that it will take a mechanic between 3 and 5 minutes to change the oil?
d.What is the variance of the time it takes to change the oil?
(Essay)
4.7/5  (27)
(27)
In a normal distribution, it is known that 27.34% of all the items are included from 100 up to the mean, and another 45.99% of all the items are included from the mean up to 145. Determine the mean and the standard deviation of the distribution.
(Short Answer)
4.8/5  (34)
(34)
In a standard normal distribution, the range of values of z is from
(Multiple Choice)
4.8/5  (34)
(34)
Exhibit 6-3
Consider the continuous random variable X, which has a uniform distribution over the interval from 20 to 28.
-Refer to Exhibit 6-3. The probability density function has what value in the interval between 20 and 28?
(Multiple Choice)
4.7/5  (40)
(40)
Showing 21 - 40 of 174
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)