Exam 6: Continuous Probability Distributions

Exhibit 6-8 The life expectancy of a particular brand of tire is normally distributed with a mean of 40,000 and a standard deviation of 5,000 miles. -Refer to Exhibit 6-8. What percentage of tires will have a life of 34,000 to 46,000 miles?

Given that Z is a standard normal random variable, what is the value of Z if the are to the left of Z is 0.0559?

A negative value of Z indicates that

Larger values of the standard deviation result in a normal curve that is

The contents of soft drink bottles are normally distributed with a mean of twelve ounces and a standard deviation of one ounce. a.What is the probability that a randomly selected bottle will contain more than ten ounces of soft drink? b.What is the probability that a randomly selected bottle will contain between 9.5 and 11 ounces? c.What percentage of the bottles will contain less than 10.5 ounces of soft drink?

For a normal distribution, a positive value of z indicates that

For the standard normal probability distribution, the area to the left of the mean is

The driving time for an individual from his home to his work is uniformly distributed between 300 to 480 seconds. a.Determine the probability density function. b.Compute the probability that the driving time will be less than or equal to 435 seconds. c.Determine the expected driving time. d.Compute the variance. e.Compute the standard deviation.

The price of a bond is uniformly distributed between $80 and $85. a.What is the probability that the bond price will be at least $83? b.What is the probability that the bond price will be between $81 to $90? c.Determine the expected price of the bond. d.Compute the standard deviation for the bond price.

X is a normally distributed random variable with a mean of 8 and a standard deviation of 4. The probability that X is between 1.48 and 15.56 is

Exhibit 6-1 The assembly time for a product is uniformly distributed between 6 to 10 minutes. -Refer to Exhibit 6-1. The probability density function has what value in the interval between 6 and 10?

The center of a normal curve is

Z is a standard normal random variable. What is the value of Z if the area to the right of Z is 0.1112?

For any continuous random variable, the probability that the random variable takes avalue less than zero

Z is a standard normal random variable. What is the value of Z if the area between -Z and Z is 0.754?

The average life expectancy of dishwashers produced by a company is 6 years with a standard deviation of 8 months. Assume that the lives of dishwashers are normally distributed. a.What is the probability that a randomly selected dishwasher will have a life expectancy of at least 7 years? b.Dishwashers that fail operating in less than 4 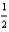years will be replaced free of charge. What percent of dishwashers are expected to be replaced free of charge? c.What are the minimum and the maximum life expectancy of the middle 95% of the dishwashers' lives? Give your answer in months. d.If 155 of this year's dishwasher production fail operating in less than 4 years and 4 months, how many dishwashers were produced this year?

The uniform, normal, and exponential distributions are

Exhibit 6-3 Consider the continuous random variable X, which has a uniform distribution over the interval from 20 to 28. -Refer to Exhibit 6-3. The probability that X will take on a value of at least 26 is

The uniform probability distribution is used with

The length of time patients must wait to see a doctor in a local clinic is uniformly distributed between 15 minutes and 2 1/2 hours. a.What is the probability of a patient waiting exactly 50 minutes? b.What is the probability that a patient would have to wait between 45 minutes and 2 hours? c.Compute the probability that a patient would have to wait over 2 hours. d.Determine the expected waiting time and its standard deviation.