Exam 1: Functions and Their Graphs
Exam 1: Functions and Their Graphs301 Questions
Exam 2: Linear and Quadratic Functions301 Questions
Exam 3: Polynomial and Rational Functions350 Questions
Exam 4: Exponential and Logarithmic Functions518 Questions
Exam 5: Trigonometric Functions366 Questions
Exam 6: Analytic Trigonometry402 Questions
Exam 7: Applications of Trigonometric Functions103 Questions
Exam 8: Polar Coordinates; Vectors270 Questions
Exam 9: Analytic Geometry197 Questions
Exam 10: Systems of Equations and Inequalities235 Questions
Exam 11: Sequences; Induction; the Binomial Theorem238 Questions
Exam 12: Counting and Probability108 Questions
Exam 13: A Preview of Calculus: the Limit, Derivative, and Integral of a Function145 Questions
Exam 14: Review228 Questions
Select questions type
The graph of a function f is given. Use the graph to answer the question.
-For what numbers x is f(x) > 0? 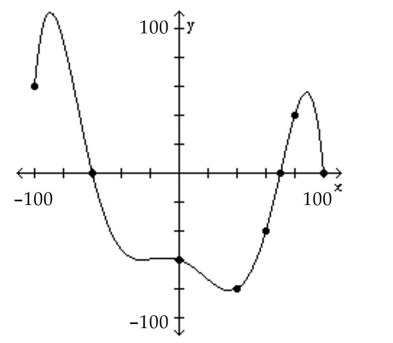
(Multiple Choice)
4.9/5  (30)
(30)
Choose the one alternative that best completes the statement or answers the question.
Graph the function by starting with the graph of the basic function and then using the techniques of shifting,
compressing, stretching, and/or reflecting.
-
 A)
A)
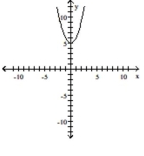 B)
B)
 C)
C)
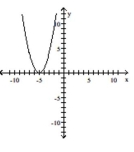 D)
D)
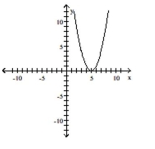
(Multiple Choice)
4.7/5  (22)
(22)
The graph of a function f is given. Use the graph to answer the question.
-Is f(8) positive or negative? 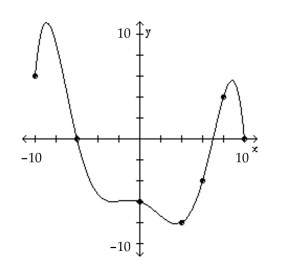
(Multiple Choice)
4.8/5  (31)
(31)
Determine algebraically whether the function is even, odd, or neither.
-
(Multiple Choice)
4.7/5  (33)
(33)
For the function, find the average rate of change of f from 1 to x:
-
(Multiple Choice)
4.9/5  (35)
(35)
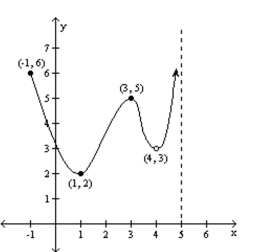
For the graph of the function y = f(x), find the absolute maximum and the absolute minimum, if it exists.
-
(Multiple Choice)
4.8/5  (23)
(23)
The graph of a function f is given. Use the graph to answer the question.
-Find the numbers, if any, at which f has a local minimum. What are the local minima? 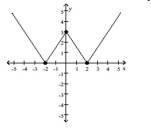
(Multiple Choice)
4.8/5  (34)
(34)
Write the word or phrase that best completes each statement or answers the question.
-An electric company has the following rate schedule for electricity usage in single-family residences: Monthly service charge \ 4.93 Per kilowatt service charge 1st 300 kilowatts (\ 0.11589/ Over 300 kilowatts (\ 0.13321/ What is the charge for using 300 kilowatts in one month?
What is the charge for using 375 kilowatts in one month?
Construct a function that gives the monthly charge C for x kilowatts of electricity.
(Essay)
4.8/5  (38)
(38)
Determine whether the equation defines y as a function of x.
-
(Multiple Choice)
4.7/5  (23)
(23)
Solve the problem.
-A farmer's silo is the shape of a cylinder with a hemisphere as the roof. If the height of the silo is 66 feet and the radius of the hemisphere is r feet, express the volume of the silo as a function of r. A)
B)
C)
D)
(Multiple Choice)
4.8/5  (39)
(39)
Use a graphing utility to graph the function over the indicated interval and approximate any local maxima and local
minima. Determine where the function is increasing and where it is decreasing. If necessary, round answers to two
decimal places.
-
(Multiple Choice)
4.7/5  (34)
(34)
For the given functions f and g, find the requested function and state its domain.
-
Find .
(Multiple Choice)
4.9/5  (40)
(40)
Showing 201 - 220 of 301
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)