Exam 16: Sound and Hearing
Exam 2: Motion Along a Straight Line55 Questions
Exam 3: Motion in Two or Three Dimensions59 Questions
Exam 4: Newtons Laws of Motion50 Questions
Exam 5: Applying Newtons Laws139 Questions
Exam 6: Work and Kinetic Energy109 Questions
Exam 7: Potential Energy and Energy Conservation50 Questions
Exam 8: Momentum, Impulse, and Collisions99 Questions
Exam 9: Rotation of Rigid Bodies26 Questions
Exam 10: Dynamics of Rotational Motion49 Questions
Exam 11: Equilibrium and Elasticity50 Questions
Exam 12: Fluid Mechanics54 Questions
Exam 13: Gravitation52 Questions
Exam 14: Periodic Motion109 Questions
Exam 15: Mechanical Waves50 Questions
Exam 16: Sound and Hearing121 Questions
Exam 17: Temperature and Heat60 Questions
Exam 18: Thermal Properties of Matter41 Questions
Exam 19: The First Law of Thermodynamics55 Questions
Exam 20: The Second Law of Thermodynamics52 Questions
Exam 21: Electric Charge and Electric Field54 Questions
Exam 22: Gausss Law54 Questions
Exam 23: Electric Potential88 Questions
Exam 24: Capacitance and Dielectrics70 Questions
Exam 25: Current, Resistance, and Electromotive Force44 Questions
Exam 26: Direct-Current Circuits51 Questions
Exam 27: Magnetic Field and Magnetic Forces105 Questions
Exam 28: Sources of Magnetic Field82 Questions
Exam 29: Electromagnetic Induction51 Questions
Exam 30: Inductance88 Questions
Exam 31: Alternating Current51 Questions
Exam 32: Electromagnetic Waves Optics53 Questions
Exam 33: The Nature and Propagation of Light31 Questions
Exam 34: Geometric Optics89 Questions
Exam 35: Interference59 Questions
Select questions type
Molecular speeds: The root-mean-square speed (thermal speed) for a certain gas at 100°C is 0.500 km/s. If the temperature of the gas is now increased to 200°C, the root-mean-square (thermal) speed will be closest to
Free
(Multiple Choice)
4.8/5  (32)
(32)
Correct Answer:
A
Ideal gas law: Sometimes an experiment requires a certain pure gas to be used at reduced pressure. One way to achieve this is to purchase a sealed glass container filled with the gas, and to introduce the gas into a vacuum by attaching the glass container to the vacuum chamber and breaking the tip of the glass container using a metallic bean and a magnet. If the volume of the glass container is 1.0 L and it is at a pressure of 1.0 × 105 Pa and if the vacuum chamber has a volume of 2.0 L, what will the pressure be after the gas, which is to be assumed to be an ideal gas, is released into the vacuum chamber and the temperature has returned to its original value? (Note that the glass container remains part of the system.)
Free
(Multiple Choice)
4.9/5  (34)
(34)
Correct Answer:
A
Molecular speeds: A sample of an ideal gas is slowly compressed to one-half its original volume with no change in pressure. If the original root-mean-square speed (thermal speed) of the gas molecules was V, the new speed is
Free
(Multiple Choice)
4.7/5  (23)
(23)
Correct Answer:
E
Molecular speeds: Eleven molecules have speeds 16, 17, 18, . . . , 26 m/s. Calculate the root-mean-square of this group of molecules.
(Multiple Choice)
4.8/5  (38)
(38)
Ideal gas law: The figure shows a 50-kg frictionless cylindrical piston that floats on 0.68 mol of compressed air at 30°C. How far does the piston move if the temperature is increased to 300°C? 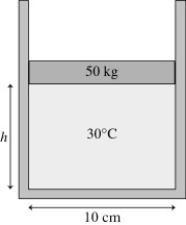
(Multiple Choice)
4.8/5  (31)
(31)
Molecular speeds: A 5.0-liter gas tank holds 1.7 moles of monatomic helium (He) and 1.10 mole of diatomic oxygen (O2), at a temperature of 260 K. The ATOMIC masses of helium and oxygen are 4.0 g/mol and 16.0 g/mol, respectively. What is the ratio of the root-mean-square (thermal) speed of helium to that of oxygen?
(Multiple Choice)
4.7/5  (34)
(34)
Thermal expansion: Two identical concrete slabs lie flat and in contact with each other as shown in the figure. If the temperature increases by 40°C, the lower edges opposite the contact edges remained fixed in position, and the lower edges of the contact side remain in contact, at what angle will the slabs be tilted? The coefficient of thermal expansion of the concrete is 10 × 10-6/K. 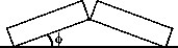
(Multiple Choice)
4.8/5  (31)
(31)
Calorimetry: How many grams of ice at - 13°C must be added to 711 grams of water that is initially at a temperature of 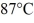 to produce water at a final temperature of
to produce water at a final temperature of 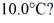 Assume that no heat is lost to the surroundings and that the container has negligible mass. The specific heat of liquid water is 4190 J/kg · °C and of ice is 2050 J/kg · °C. For water the normal melting point is 0.00°C and the heat of fusion is 334 × 103 J/kg. The normal boiling point is 100°C and the heat of vaporization is 2.26 × 106 J/kg.
Assume that no heat is lost to the surroundings and that the container has negligible mass. The specific heat of liquid water is 4190 J/kg · °C and of ice is 2050 J/kg · °C. For water the normal melting point is 0.00°C and the heat of fusion is 334 × 103 J/kg. The normal boiling point is 100°C and the heat of vaporization is 2.26 × 106 J/kg.
(Short Answer)
4.8/5  (39)
(39)
Molecular speeds: A container is filled with a mixture of helium (light molecules) and oxygen (heavy molecules) gases. A thermometer in the container reads 22°C. Which gas molecules have the greater average kinetic energy?
(Multiple Choice)
4.8/5  (26)
(26)
Molecular speeds: A 0.10- 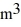 gas tank holds 5.0 moles of nitrogen gas (N2), at a temperature of
gas tank holds 5.0 moles of nitrogen gas (N2), at a temperature of 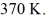 The atomic mass of nitrogen is 14 g/mol, the molecular radius is 3.0 × 10-10 m, and the Boltzmann constant is 1.38 × 10-23 J/K. The root-mean-square speed (thermal speed) of the molecules is closest to
The atomic mass of nitrogen is 14 g/mol, the molecular radius is 3.0 × 10-10 m, and the Boltzmann constant is 1.38 × 10-23 J/K. The root-mean-square speed (thermal speed) of the molecules is closest to
(Multiple Choice)
4.8/5  (38)
(38)
Calorimetry: A person makes ice tea by adding ice to 1.8 kg of hot tea, initially at 80°C. How many kilograms of ice, initially at 0.00°C, are required to bring the mixture to 10°C? The heat of fusion of ice is 334 kJ/kg, and we can assume that tea has essentially the same thermal properties as water, so its specific heat is 4190 J/(kg ∙ K).
(Multiple Choice)
4.9/5  (31)
(31)
Calorimetry: An 80-g aluminum calorimeter contains 380 g of water at an equilibrium temperature of 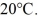 A
A 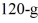 piece of metal, initially at
piece of metal, initially at  is added to the calorimeter. The final temperature at equilibrium is 32°C. Assume there is no external heat exchange. The specific heats of aluminum and water are 910 J/kg · K and 4190 J/kg · K, respectively. The specific heat of the metal is closest to
is added to the calorimeter. The final temperature at equilibrium is 32°C. Assume there is no external heat exchange. The specific heats of aluminum and water are 910 J/kg · K and 4190 J/kg · K, respectively. The specific heat of the metal is closest to
(Multiple Choice)
4.7/5  (36)
(36)
Conduction of heat: Some properties of glass are listed here. Density: 2300 kg/m3
Specific heat: 840 J/kg · °C
Coefficient of linear thermal expansion: 8.5 × 10-6 (°C)-1
Thermal conductivity: 0.80 W/(m · °C)
A glass window pane is 2.7 m high, 2.4 m wide, and 2.0 mm thick. The temperature at the inner surface of the glass is 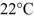 and at the outer surface 4.0°C. How much heat is lost each hour through the window under steady state conditions?
and at the outer surface 4.0°C. How much heat is lost each hour through the window under steady state conditions?
(Multiple Choice)
4.8/5  (32)
(32)
Conduction of heat: Under steady state conditions, a piece of wood 350 mm by 350 mm and 15 mm thick conducts heat through its thickness and loses no appreciable heat through its well-insulated sides. The rate of heat flow is measured to be 14.0 W when the temperature difference across its thickness is 28°C. Determine the thermal conductivity of this wood.
(Multiple Choice)
4.9/5  (37)
(37)
Molecular speeds: A sealed container holds 0.020 moles of nitrogen (N2) gas at a pressure of 1.5 atmospheres and a temperature of 290 K. The atomic mass of nitrogen is 14 g/mol. The Boltzmann constant is 1.38 × 10-23 J/K and the ideal gas constant is R = 8.314 J/mol ∙ K = 0.0821 L ∙ atm/mol ∙ K. The average translational kinetic energy of a nitrogen molecule is closest to
(Multiple Choice)
4.9/5  (28)
(28)
Radiation: A solid metal sphere is 15.0 cm in diameter and has surface of uniform color. When its surface is at 112°C, you measure that it radiates energy at a rate of 71.3 W. What is the emissivity of the surface of this object? Any heat that enters the sphere from the outside environment is negligible. The Stefan-Boltzmann constant is 5.670 × 10-8 W/m2 · K4.
(Short Answer)
4.9/5  (43)
(43)
Molecular speeds: A sample of an ideal gas is slowly compressed to one-half its original volume with no change in temperature. What happens to the average speed of the molecules in the sample?
(Multiple Choice)
4.9/5  (40)
(40)
Molecular speeds: What is the average kinetic energy of an ideal gas molecule at 569°C? (The Boltzmann constant is 1.38 × 10-23 J/K.)
(Multiple Choice)
4.8/5  (37)
(37)
Molecular speeds: What is the average translational kinetic energy per molecule of an ideal gas at a temperature of 300 K? The Boltzmann constant is 1.38 × 10-23 J/K.
(Multiple Choice)
4.8/5  (33)
(33)
Ideal gas law: An ideal gas is at a pressure 1.00 × 105 N/m2 and occupies a volume 2.00 m3. If the gas is compressed to a volume 1.00 m3 while the temperature remains constant, what will be the new pressure in the gas?
(Multiple Choice)
4.9/5  (41)
(41)
Showing 1 - 20 of 121
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)