Exam 8: Momentum, Impulse, and Collisions
Exam 2: Motion Along a Straight Line55 Questions
Exam 3: Motion in Two or Three Dimensions59 Questions
Exam 4: Newtons Laws of Motion50 Questions
Exam 5: Applying Newtons Laws139 Questions
Exam 6: Work and Kinetic Energy109 Questions
Exam 7: Potential Energy and Energy Conservation50 Questions
Exam 8: Momentum, Impulse, and Collisions99 Questions
Exam 9: Rotation of Rigid Bodies26 Questions
Exam 10: Dynamics of Rotational Motion49 Questions
Exam 11: Equilibrium and Elasticity50 Questions
Exam 12: Fluid Mechanics54 Questions
Exam 13: Gravitation52 Questions
Exam 14: Periodic Motion109 Questions
Exam 15: Mechanical Waves50 Questions
Exam 16: Sound and Hearing121 Questions
Exam 17: Temperature and Heat60 Questions
Exam 18: Thermal Properties of Matter41 Questions
Exam 19: The First Law of Thermodynamics55 Questions
Exam 20: The Second Law of Thermodynamics52 Questions
Exam 21: Electric Charge and Electric Field54 Questions
Exam 22: Gausss Law54 Questions
Exam 23: Electric Potential88 Questions
Exam 24: Capacitance and Dielectrics70 Questions
Exam 25: Current, Resistance, and Electromotive Force44 Questions
Exam 26: Direct-Current Circuits51 Questions
Exam 27: Magnetic Field and Magnetic Forces105 Questions
Exam 28: Sources of Magnetic Field82 Questions
Exam 29: Electromagnetic Induction51 Questions
Exam 30: Inductance88 Questions
Exam 31: Alternating Current51 Questions
Exam 32: Electromagnetic Waves Optics53 Questions
Exam 33: The Nature and Propagation of Light31 Questions
Exam 34: Geometric Optics89 Questions
Exam 35: Interference59 Questions
Select questions type
Moment of inertia: A slender uniform rod 100.00 cm long is used as a meter stick. Two parallel axes that are perpendicular to the rod are considered. The first axis passes through the 50-cm mark and the second axis passes through the 30-cm mark. What is the ratio of the moment of inertia through the second axis to the moment of inertia through the first axis?
Free
(Multiple Choice)
4.9/5  (34)
(34)
Correct Answer:
A
Rotational kinetic energy: A uniform solid sphere of mass M and radius R rotates with an angular speed ω about an axis through its center. A uniform solid cylinder of mass M, radius R, and length 2R rotates through an axis running through the central axis of the cylinder. What must be the angular speed of the cylinder so it will have the same rotational kinetic energy as the sphere?
Free
(Multiple Choice)
4.9/5  (42)
(42)
Correct Answer:
D
Conservation of angular momentum: A uniform disk has a mass of 3.7 kg and a radius of 0.40 m. The disk is mounted on frictionless bearings and is used as a turntable. The turntable is initially rotating at 30 rpm. A thin-walled hollow cylinder has the same mass and radius as the disk. It is released from rest, just above the turntable, and on the same vertical axis. The hollow cylinder slips on the turntable for 0.20 s until it acquires the same final angular velocity as the turntable. What is the final angular momentum of the system?
Free
(Multiple Choice)
4.8/5  (42)
(42)
Correct Answer:
A
Torque: A force 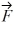 = 3.00 N
= 3.00 N  - 2.00 N
- 2.00 N  acts as a location
acts as a location  = 1.00 m
= 1.00 m  + 2.00 m
+ 2.00 m  on an object. What is the torque that this force applies about an axis through the origin perpendicular to the xy-plane?
on an object. What is the torque that this force applies about an axis through the origin perpendicular to the xy-plane?
(Multiple Choice)
4.9/5  (32)
(32)
Angular momentum: Three solid, uniform, cylindrical flywheels, each of mass 65.0 kg and radius 1.47 m rotate independently around a common axis. Two of the flywheels rotate in one direction at 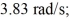 the other rotates in the opposite direction at 3.42 rad/s. Calculate the magnitude of the net angular momentum of the system.
the other rotates in the opposite direction at 3.42 rad/s. Calculate the magnitude of the net angular momentum of the system.
(Multiple Choice)
4.7/5  (40)
(40)
Rotational dynamics about a fixed axis: The rotating systems shown in the figure differ only in that the two identical movable masses are positioned a distance r from the axis of rotation (left), or a distance r/2 from the axis of rotation (right). You release the hanging blocks simultaneously from rest, and call tL the time taken by the block on the left and tR the time taken by the block on the right to reach the bottom, respectively. The bar, pulley, and rope have negligible mass, the rope does not slip, and there is no friction in the axle of the pulley. Under these conditions 
(Multiple Choice)
4.8/5  (38)
(38)
Moment of inertia: A dumbbell-shaped object is composed by two equal masses, m, connected by a rod of negligible mass and length r. If I1 is the moment of inertia of this object with respect to an axis passing through the center of the rod and perpendicular to it and I2 is the moment of inertia with respect to an axis passing through one of the masses, it follows that
(Multiple Choice)
4.8/5  (28)
(28)
Basic rotational quantities: A horizontal disk rotates about a vertical axis through its center. Point P is midway between the center and the rim of the disk, and point Q is on the rim. If the disk turns with constant angular velocity, which of the following statements about it are true? (There may be more than one correct choice.)
(Multiple Choice)
5.0/5  (28)
(28)
Rotational dynamics about a fixed axis: In the figure, a mass of 31.77 kg is attached to a light string that is wrapped around a cylindrical spool of radius 10.0 cm and moment of inertia 4.00 kg · m2. The spool is suspended from the ceiling, and the mass is then released from rest a distance 5.70 m above the floor. How long does it take to reach the floor? 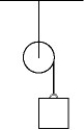
(Multiple Choice)
4.8/5  (29)
(29)
Rotational kinematics with constant angular acceleration: A 4.50-kg wheel that is 34.5 cm in diameter rotates through an angle of 13.8 rad as it slows down uniformly from 22.0 rad/s to 13.5 rad/s. What is the magnitude of the angular acceleration of the wheel?
(Multiple Choice)
4.8/5  (41)
(41)
Rolling: A solid sphere, solid cylinder, and a hollow pipe all have equal masses and radii and are of uniform density. If the three are released simultaneously at the top of an inclined plane and roll without slipping, which one will reach the bottom first?
(Multiple Choice)
4.7/5  (40)
(40)
Rolling: A ball is released from rest on a no-slip surface, as shown in the figure. After reaching its lowest point, the ball begins to rise again, this time on a frictionless surface as shown in the figure. When the ball reaches its maximum height on the frictionless surface, it is 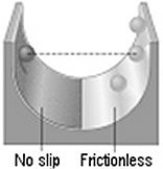
(Multiple Choice)
4.9/5  (39)
(39)
Precession: An object is rotating with an angular momentum 4.00 kg ∙ m2/s while being acted on by a constant torque 3.00 N∙m . What is the angular speed of precession of the angular velocity of the object?
(Multiple Choice)
4.7/5  (31)
(31)
Precession: In the figure, the rotor of a gyroscope is a uniform disk that has a radius of 13.8 cm and a moment of inertia about its axis of 4.0 × 10-3 kg∙m2. The length of the rotor shaft is 6.9 cm. The ball pivot at P is frictionless. At a given instant, the rotor shaft is horizontal and the rotor is rotating with an angular velocity of 90 rad/s about its axis OP, as shown. The rotor is viewed from point Q on the axis. The precessional linear velocity of point O, including the direction seen from point Q, is closest to 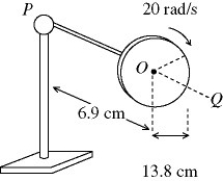
(Multiple Choice)
4.9/5  (29)
(29)
Rolling: A uniform solid sphere is rolling without slipping along a horizontal surface with a speed of 5.50 m/s when it starts up a ramp that makes an angle of 25.0° with the horizontal. What is the speed of the sphere after it has rolled 3.00 m up the ramp, measured along the surface of the ramp?
(Multiple Choice)
4.7/5  (29)
(29)
Basic rotational quantities: When you ride a bicycle, in what direction is the angular velocity of the wheels?
(Multiple Choice)
4.9/5  (34)
(34)
Rotational dynamics about a fixed axis: In an experiment, a student brings up the rotational speed of a piece of laboratory apparatus to 30.0 rpm. She then allows the apparatus to slow down uniformly on its own, and counts 240 revolutions before the apparatus comes to a stop. The moment of inertia of the apparatus is known to be 0.0850 kg ∙ m2. What is the magnitude of the retarding torque on the apparatus?
(Multiple Choice)
4.9/5  (33)
(33)
Conservation of angular momentum: A 5.0-m radius playground merry-go-round with a moment of inertia of 2000 kg ∙ m2 is rotating freely with an angular speed of 1.0 rad/s. Two people, each having a mass of 60 kg, are standing right outside the edge of the merry-go-round and step on it with negligible speed. What is the angular speed of the merry-go-round right after the two people have stepped on?
(Multiple Choice)
4.8/5  (40)
(40)
Conservation of angular momentum: A turntable has a radius of 0.80 m and a moment of inertia of 2.0 kg · m2. The turntable is rotating with an angular velocity of 1.5 rad/s about a vertical axis though its center on frictionless bearings. A very small 0.40-kg ball is projected horizontally toward the turntable axis with a velocity of 3.0 m/s. The ball is caught by a very small and very light cup-shaped mechanism on the rim of the turntable (see figure). What is the angular velocity of the turntable just after the ball is caught? 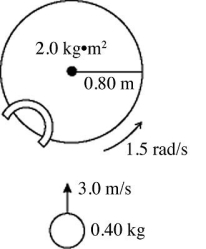
(Multiple Choice)
4.8/5  (35)
(35)
Conservation of angular momentum: A potter's wheel, with rotational inertia 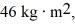 is spinning freely at 40 rpm. The potter drops a lump of clay onto the wheel, where it sticks a distance 1.2 m from the rotational axis. If the subsequent angular speed of the wheel and clay is 32 rpm what is the mass of the clay?
is spinning freely at 40 rpm. The potter drops a lump of clay onto the wheel, where it sticks a distance 1.2 m from the rotational axis. If the subsequent angular speed of the wheel and clay is 32 rpm what is the mass of the clay?
(Multiple Choice)
5.0/5  (26)
(26)
Showing 1 - 20 of 99
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)