Exam 4: Probability and Probability Distributions
Exam 1: Describing Data With Graphs94 Questions
Exam 2: Describing Data With Numerical Measures186 Questions
Exam 3: Describing Bivariate Data35 Questions
Exam 4: Probability and Probability Distributions136 Questions
Exam 5: Several Useful Discrete Distributions129 Questions
Exam 6: The Normal Probability Distribution196 Questions
Exam 7: Sampling Distributions162 Questions
Exam 8: Large-Sample Estimation173 Questions
Exam 9: Large-Sample Tests of Hypotheses210 Questions
Exam 10: Inference From Small Samples261 Questions
Exam 11: The Analysis of Variance156 Questions
Exam 12: Linear Regression and Correlation165 Questions
Exam 13: Multiple Regression Analysis178 Questions
Exam 14: Analysis of Categorical Data136 Questions
Exam 15: Nonparametric Statistics198 Questions
Select questions type
Invariably, Venn diagrams illustrate the intersection of two events.
(True/False)
4.8/5  (44)
(44)
The number of homes sold by a real estate agency in a three week period is an example of a continuous random variable.
(True/False)
4.8/5  (43)
(43)
Let x be a random variable with the following distribution:
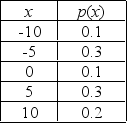 Round your answer to three decimal places, if necessary.
a. Find the expected value of x.
______________
b. Find the standard deviation of x.
______________
c. What is the probability that x is further than one standard deviation from the mean?
______________
Round your answer to three decimal places, if necessary.
a. Find the expected value of x.
______________
b. Find the standard deviation of x.
______________
c. What is the probability that x is further than one standard deviation from the mean?
______________
(Short Answer)
4.7/5  (34)
(34)
The conditional probability of event B, given that event A has occurred is defined by: 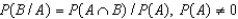 .
.
(True/False)
4.8/5  (45)
(45)
Margaret selected two M&M candies at random from a bowl containing three M&Ms, one red, one yellow, and one orange.
a. Describe the sample space.
__________________________________________
b. Suppose Margaret draws the first M&M, likes it, and eats it before drawing the second M&M. What is the sample space in this case?
__________________________________________
(Short Answer)
4.8/5  (44)
(44)
The addition law of probability theory is used to compute the probability for the occurrence of a union of two or more events; namely, given two events A and B, 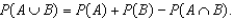
(True/False)
4.8/5  (38)
(38)
Suppose P(A) = 0.4, P(B) = 0.3, and P(A  B) = 0. Which one of the following statements correctly defines the relationship between events A and B?
B) = 0. Which one of the following statements correctly defines the relationship between events A and B?
(Multiple Choice)
4.9/5  (35)
(35)
At a local pet adoption center for dogs and cats, it is known that if a person adopts a pet, there is a 0.45 probability that it will be a cat and a 0.55 probability that it will be a dog. If a cat is adopted, the probability that it is female is 0.60. If a dog is adopted, the probability that it is female is 0.35. An adopted pet is selected at random and is found to be male. What is the probability that the adopted pet is a dog?
______________
(Short Answer)
4.8/5  (29)
(29)
The probability distribution of the number of accidents in Grand Rapids, Michigan, each day is given by  This distribution is an example of:
This distribution is an example of:
(Multiple Choice)
4.9/5  (33)
(33)
Statistics reasons from the population to the sample, whereas probability acts in reverse, moving from the sample to the population.
(True/False)
4.8/5  (34)
(34)
An event is a collection of one or more simple events of an experiment.
(True/False)
4.8/5  (31)
(31)
A sample is selected from one of two populations, S1 and S2, with probabilities P(S1) = 0.80 and P(S2) = 0.20. If the sample has been selected from S1, the probability of observing an event A is P(A / S1) = 0.15. Similarly, If the sample has been selected from S2, the probability of observing A is P(A / S2) = 0.25.
Round your answer to four decimal places, if necessary
a. If a sample is randomly selected from one of the two populations, what is the probability that event A occurs?
______________
If a sample is randomly selected and event A is observed, what is the probability that the sample was selected:
b. From population S1?
______________
c. From population S2?
______________
(Short Answer)
4.7/5  (37)
(37)
Screening tests (e.g., AIDS testing) are evaluated on the probability of a false negative or a false positive, and both of these are:
(Multiple Choice)
4.8/5  (44)
(44)
If the mean of the random variable x is larger than the mean of the random variable y, then the variance of x must be larger than the variance of y.
(True/False)
4.9/5  (31)
(31)
Two events A and B are said to be independent if P(A  B) = P(A) + P(B).
B) = P(A) + P(B).
(True/False)
4.9/5  (34)
(34)
The complement of an event A, denoted by 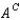 , consists of all the simple events in the sample space S that are not in A.
, consists of all the simple events in the sample space S that are not in A.
(True/False)
4.7/5  (33)
(33)
When Cynthia enters a grocery store, there are three simple events: buy nothing, buy a small amount, or buy a large amount. In this situation, if Cynthia buys a small amount, she cannot also buy a large amount or buy nothing. Thus the three events are:
(Multiple Choice)
4.8/5  (31)
(31)
Four different kinds of radar systems, designed to detect or monitor the airspace around a major airport for high flying objects, low flying objects, runway traffic, and wind shear, operate independently. If each system has probability 0.90 of functioning correctly, find the probability at least one radar system will fail.
______________
(Short Answer)
4.9/5  (28)
(28)
Different events that have no outcomes in common are mutually exclusive events.
(True/False)
4.7/5  (33)
(33)
Showing 61 - 80 of 136
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)