Exam 42: Nuclear Physics
Exam 1: Units, Physical Quantities, and Vectors107 Questions
Exam 2: Motion Along a Straight Line59 Questions
Exam 3: Motion in Two or Three Dimensions50 Questions
Exam 4: Newtons Laws of Motion44 Questions
Exam 5: Applying Newtons Laws95 Questions
Exam 6: Work and Kinetic Energy54 Questions
Exam 7: Potential Energy and Energy Conservation55 Questions
Exam 8: Momentum, Impulse, and Collisions50 Questions
Exam 9: Rotation of Rigid Bodies26 Questions
Exam 10: Equilibrium and Elasticity50 Questions
Exam 11: Fluid Mechanics50 Questions
Exam 12: Gravitation50 Questions
Exam 13: Periodic Motion50 Questions
Exam 14: Mechanical Waves44 Questions
Exam 15: Sound and Hearing66 Questions
Exam 16: Temperature and Heat63 Questions
Exam 17: Thermal Properties of Matter58 Questions
Exam 18: The First Law of Thermodynamics52 Questions
Exam 19: The Second Law of Thermodynamics50 Questions
Exam 20: Electric Charge and Electric Field58 Questions
Exam 21: Gausss Law41 Questions
Exam 22: Electric Potential55 Questions
Exam 23: Capacitance and Dielectrics52 Questions
Exam 24: Current, Resistance, and Electromotive Force50 Questions
Exam 25: Direct-Current Circuits53 Questions
Exam 26: Magnetic Field and Magnetic Forces36 Questions
Exam 27: Sources of Magnetic Field51 Questions
Exam 28: Electromagnetic Induction39 Questions
Exam 29: Inductance26 Questions
Exam 30: Alternating Current49 Questions
Exam 31: Electromagnetic Waves47 Questions
Exam 32: The Nature and Propagation of Light28 Questions
Exam 33: Geometric Optics81 Questions
Exam 34: Interference33 Questions
Exam 35: Diffraction49 Questions
Exam 36: Relativity51 Questions
Exam 37: Photons: Light Waves Behaving As Particles38 Questions
Exam 38: Particles Behaving As Waves52 Questions
Exam 39: Quantum Mechanics40 Questions
Exam 40: Atomic Structure41 Questions
Exam 41: Molecules and Condensed Matter31 Questions
Exam 42: Nuclear Physics89 Questions
Exam 43: Particle Physics and Cosmology44 Questions
Select questions type
An ancient rock is found to contain 40Ar gas, indicating that 77% of the 40K in the rock has decayed since the rock solidified. Any argon would have boiled out of liquid rock. The half-life of 40K is 1.25 billion years. How long ago did the rock solidify?
Free
(Multiple Choice)
4.9/5  (32)
(32)
Correct Answer:
A
Calculate the amount of energy that is released in the fusion reaction 2H + 2H → 4He, given the masses:
2H: 2.014102 u
4He: 4.002603 u
(1 u = 931.5 MeV/c2)
Free
(Multiple Choice)
4.7/5  (37)
(37)
Correct Answer:
A
If a nucleus decays by β+ decay to a daughter nucleus, which of the following statements about this decay are correct? (There may be more than one correct choice.)
Free
(Multiple Choice)
4.9/5  (36)
(36)
Correct Answer:
B, C, D
A 57-kg researcher absorbs 6.3 × 108 neutrons in a workday. The energy of the neutrons is 2.6 MeV. The relative biological efficiency (RBE) for fast neutrons is 10. What is the equivalent dosage of the radiation exposure, in mrem, of this worker?
(Multiple Choice)
4.9/5  (34)
(34)
Two deuterium nuclei, 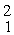 H, fuse to produce a tritium nucleus,
H, fuse to produce a tritium nucleus, 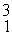 H, and a hydrogen nucleus. A neutral deuterium atom has a mass of 2.014102 u; a neutral tritium atom has a mass of 3.016049 u; a neutral hydrogen atom has a mass of 1.007825 u; a neutron has a mass of 1.008665 u; and a proton has a mass of 1.007276 u. How much energy is released in the process? (1 u = 931.494 MeV/c2)
H, and a hydrogen nucleus. A neutral deuterium atom has a mass of 2.014102 u; a neutral tritium atom has a mass of 3.016049 u; a neutral hydrogen atom has a mass of 1.007825 u; a neutron has a mass of 1.008665 u; and a proton has a mass of 1.007276 u. How much energy is released in the process? (1 u = 931.494 MeV/c2)
(Multiple Choice)
4.9/5  (32)
(32)
How many days are required for a radioactive sample, with a half-life of 5.7 d and an initial activity of 1.07 × 105 Bq, to decay to an activity of 100 Bq?
(Multiple Choice)
4.7/5  (41)
(41)
Plutonium-239 decays into uranium-235 plus an alpha particle. The energy released in the process is 5.24 MeV. Given the following mass values 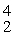 He: 4.002603 u
He: 4.002603 u 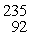 U: 235.043924 u
What is the mass of
U: 235.043924 u
What is the mass of 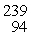 Pu in atomic mass units? (1 u = 931.494 MeV/c2)
Pu in atomic mass units? (1 u = 931.494 MeV/c2)
(Multiple Choice)
5.0/5  (47)
(47)
The primary source of the energy radiated by a star, such as the sun, is
(Multiple Choice)
4.8/5  (26)
(26)
The decay rate of an isotope is initially R0, but after one half-life has gone by, the rate is R0/2. At the end of the NEXT half-life, what will the decay rate be?
(Multiple Choice)
4.7/5  (28)
(28)
The reaction energy (Q value) for a particular reaction is -2.4 MeV, and the reaction's threshold energy is 9.60 MeV. What is the ratio of the mass of the incident particle to the mass of the stationary target nucleus?
(Multiple Choice)
4.9/5  (35)
(35)
The half-life of cobalt-60 is 5.3 years, while that of strontium-90 is 28 years. Suppose that samples of cobalt-60 and strontium-90 are such that they initially have the same activity (number of decays per second). What is true about the initial numbers of cobalt-60 and strontium-90 nuclei in these samples?
(Multiple Choice)
4.7/5  (34)
(34)
A radioactive isotope decays by β- emission with a half-life of 1.0 min. During the first 1.0 min, a particular sample emits 1000 β- particles. During the next 1.0 min, the number of β- particles this sample will emit will be closest to
(Multiple Choice)
4.7/5  (41)
(41)
A fusion reaction releases energy because the binding energy of the resulting nucleus
(Multiple Choice)
4.8/5  (36)
(36)
Modern nuclear bomb tests have created an extra high level of 14C in our atmosphere. Suppose that future archaeologists date samples from our era, but do not know about this testing. Will their dates be too young, too old, or still correct? If correct they are correct, why?
(Multiple Choice)
4.8/5  (34)
(34)
The half-life of cobalt-60 is 5.3 years, while that of strontium-90 is 28 years. Suppose you have a sample of each, such that they initially contain equal numbers of atoms of these nuclides. How will the activities (number of decays per second) of the samples compare?
(Multiple Choice)
4.8/5  (35)
(35)
When a neutron (n) collides with a uranium-235 nucleus it can induce a variety of fission reactions. One such reaction is  U + n →
U + n → 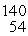 Xe +
Xe + 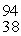 Sr + 2n. How much energy is released in this reaction, given the following mass values:
Sr + 2n. How much energy is released in this reaction, given the following mass values: 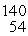 Xe: 139.921620 u
Xe: 139.921620 u 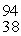 Sr : 93.915367 u
Sr : 93.915367 u 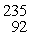 U: 235.043924 u
N: 1.008665 u
(1 u = 931.494 MeV/c2)
U: 235.043924 u
N: 1.008665 u
(1 u = 931.494 MeV/c2)
(Multiple Choice)
4.9/5  (35)
(35)
An isotope of Tc having a half-life of 6.0 h is used in bone scans. If a certain amount of this Tc is injected into the body, how long does it take for its initial decay rate to decrease BY 99%?
(Multiple Choice)
4.9/5  (40)
(40)
The detonation of a certain nuclear device results in a mass decrease of 2.60 g between the initial and the final ingredients. How much energy is released by this detonation? (c = 3.00 x 108 m/s)
(Multiple Choice)
4.7/5  (26)
(26)
How much energy is released when 1.40 μg of 3H have decayed to 3He? The mass of 3He is 3.016029 u, the mass of 3H is 3.016049 u, and 1 u = 931.494 MeV/c2.
(Multiple Choice)
4.8/5  (35)
(35)
Showing 1 - 20 of 89
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)