Exam 14: Section 4: Multiple Integration
Exam 1: Section 1: Preparation for Calculus16 Questions
Exam 1: Section 2: Preparation for Calculus26 Questions
Exam 1: Section 3: Preparation for Calculus23 Questions
Exam 1: Section 4: Preparation for Calculus16 Questions
Exam 1: Section 5: Preparation for Calculus25 Questions
Exam 1: Section 6: Preparation for Calculus8 Questions
Exam 2: Section 1: Limits and Their Properties10 Questions
Exam 2: Section 2: Limits and Their Properties14 Questions
Exam 2: Section 3: Limits and Their Properties25 Questions
Exam 2: Section 4: Limits and Their Properties20 Questions
Exam 2: Section 5 : Limits and Their Properties18 Questions
Exam 3: Section 1 : Differentiation20 Questions
Exam 3: Section 2: Differentiation25 Questions
Exam 3: Section 3: Differentiation26 Questions
Exam 3: Section 4 : Differentiation44 Questions
Exam 3: Section 5: Differentiation30 Questions
Exam 3: Section 6: Differentiation16 Questions
Exam 3: Section 7: Differentiation16 Questions
Exam 3: Section 8: Differentiation12 Questions
Exam 4: Section 1 : Applications of Differentiation19 Questions
Exam 4: Section 2: Applications of Differentiation17 Questions
Exam 4: Section 3: Applications of Differentiation17 Questions
Exam 4: Section 4: Applications of Differentiation26 Questions
Exam 4: Section 5: Applications of Differentiation23 Questions
Exam 4: Section 6: Applications of Differentiation22 Questions
Exam 4: Section 7: Applications of Differentiation15 Questions
Exam 4: Section 8: Applications of Differentiation16 Questions
Exam 4: Section 1: Integration19 Questions
Exam 4: Section 2: Integration17 Questions
Exam 4: Section 3: Integration19 Questions
Exam 4: Section 4: Integration18 Questions
Exam 4: Section 5: Integration31 Questions
Exam 4: Section 6: Integration18 Questions
Exam 4: Section 7: Integration27 Questions
Exam 4: Section 8: Integration16 Questions
Exam 4: Section 9: Integration20 Questions
Exam 6: Section 1: Differential Equations19 Questions
Exam 6: Section 2: Differential Equations25 Questions
Exam 6: Section 3: Differential Equations12 Questions
Exam 6: Section 4: Differential Equations14 Questions
Exam 6: Section 5: Differential Equations17 Questions
Exam 7: Section 1: Applications of Integration18 Questions
Exam 7: Section 2: Applications of Integration18 Questions
Exam 7: Section 3: Applications of Integration17 Questions
Exam 7: Section 4: Applications of Integration18 Questions
Exam 7: Section 5: Applications of Integration16 Questions
Exam 7: Section 6: Applications of Integration19 Questions
Exam 7: Section 7: Applications of Integration15 Questions
Exam 8: Section 1: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questions
Exam 8: Section 2: Integration Techniques, Lhôpitals Rule, and Improper Integrals18 Questions
Exam 8: Section 3: Integration Techniques, Lhôpitals Rule, and Improper Integrals20 Questions
Exam 8: Section 4: Integration Techniques, Lhôpitals Rule, and Improper Integrals19 Questions
Exam 8: Section 5: Integration Techniques, Lhôpitals Rule, and Improper Integrals14 Questions
Exam 8: Section 6: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questions
Exam 8: Section 7: Integration Techniques, Lhôpitals Rule, and Improper Integrals18 Questions
Exam 8: Section 8: Integration Techniques, Lhôpitals Rule, and Improper Integrals15 Questions
Exam 9: Section 1: Infinite Series17 Questions
Exam 9: Section 2: Infinite Series23 Questions
Exam 9: Section 3: Infinite Series18 Questions
Exam 9: Section 4: Infinite Series21 Questions
Exam 9: Section 5: Infinite Series15 Questions
Exam 9: Section 6: Infinite Series21 Questions
Exam 9: Section 7: Infinite Series18 Questions
Exam 9: Section 8: Infinite Series18 Questions
Exam 9: Section 9: Infinite Series19 Questions
Exam 9: Section 10: Infinite Series16 Questions
Exam 10: Section 1: Conics, Parametric Equations, and Polar Coordinates26 Questions
Exam 10: Section 2: Conics, Parametric Equations, and Polar Coordinates17 Questions
Exam 10: Section 3: Conics, Parametric Equations, and Polar Coordinates22 Questions
Exam 10: Section 4: Conics, Parametric Equations, and Polar Coordinates15 Questions
Exam 10: Section 5: Conics, Parametric Equations, and Polar Coordinates18 Questions
Exam 10: Section 6: Conics, Parametric Equations, and Polar Coordinates19 Questions
Exam 11: Section 1: Vectors and the Geometry of Space20 Questions
Exam 11: Section 2: Vectors and the Geometry of Space21 Questions
Exam 11: Section 3: Vectors and the Geometry of Space18 Questions
Exam 11: Section 4: Vectors and the Geometry of Space18 Questions
Exam 11: Section 5: Vectors and the Geometry of Space21 Questions
Exam 11: Section 6: Vectors and the Geometry of Space20 Questions
Exam 11: Section 7: Vectors and the Geometry of Space19 Questions
Exam 12: Section 1: Vector-Valued Functions21 Questions
Exam 12: Section 2: Vector-Valued Functions24 Questions
Exam 12: Section 3: Vector-Valued Functions18 Questions
Exam 12: Section 4: Vector-Valued Functions20 Questions
Exam 12: Section 5: Vector-Valued Functions19 Questions
Exam 13: Section 1: Functions of Several Variables19 Questions
Exam 13: Section 2: Functions of Several Variables22 Questions
Exam 13: Section 3: Functions of Several Variables23 Questions
Exam 13: Section 4: Functions of Several Variables17 Questions
Exam 13: Section 6: Functions of Several Variables20 Questions
Exam 13: Section 7: Functions of Several Variables20 Questions
Exam 13: Section 8: Functions of Several Variables20 Questions
Exam 13: Section 9: Functions of Several Variables17 Questions
Exam 13: Section 10: Functions of Several Variables18 Questions
Exam 14: Section 1: Multiple Integration20 Questions
Exam 14: Section 2: Multiple Integration19 Questions
Exam 14: Section 3: Multiple Integration20 Questions
Exam 14: Section 4: Multiple Integration18 Questions
Exam 14: Section 5: Multiple Integration18 Questions
Exam 14: Section 6: Multiple Integration19 Questions
Exam 14: Section 7: Multiple Integration19 Questions
Exam 14: Section 8: Multiple Integration19 Questions
Exam 15: Section 1: Vector Analysis21 Questions
Exam 15: Section 2: Vector Analysis18 Questions
Exam 15: Section 3: Vector Analysis18 Questions
Exam 15: Section 4: Vector Analysis18 Questions
Exam 15: Section 5: Vector Analysis21 Questions
Exam 15: Section 6: Vector Analysis18 Questions
Exam 15: Section 7: Vector Analysis18 Questions
Exam 15: Section 8: Vector Analysis17 Questions
Select questions type
Find the mass of the lamina bounded by the graphs of the equations 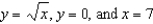 for the density
for the density 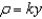 .
.
Free
(Multiple Choice)
4.8/5  (39)
(39)
Correct Answer:
C
Find the mass of the triangular lamina with vertices 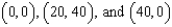 for the density
for the density 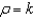 .
.
Free
(Multiple Choice)
4.8/5  (34)
(34)
Correct Answer:
C
Find the center of mass of the lamina bounded by the graphs of the equations 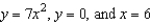 for the density
for the density 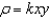 .
.
Free
(Multiple Choice)
4.9/5  (31)
(31)
Correct Answer:
B
Determine the location of the horizontal axis 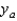 for figure (b) at which a vertical gate in a dam is to be hinged so that there is no moment causing rotation under the indicated loading (see figure (a)). The model for
for figure (b) at which a vertical gate in a dam is to be hinged so that there is no moment causing rotation under the indicated loading (see figure (a)). The model for 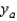 is
is 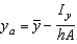 where
where 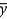 is the y-coordinate of the centroid of the gate,
is the y-coordinate of the centroid of the gate, 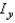 is the moment of inertia of the gate about the line
is the moment of inertia of the gate about the line 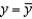 , h is the depth of the centroid below the surface, and A is the area of the gate.
, h is the depth of the centroid below the surface, and A is the area of the gate. 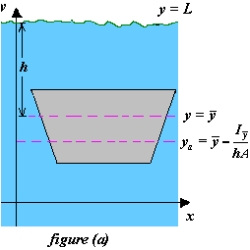

(Multiple Choice)
4.8/5  (49)
(49)
Find the center of mass of the lamina bounded by the graphs of the equations 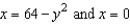 for the density
for the density 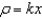 .
.
(Multiple Choice)
4.8/5  (25)
(25)
Find the mass of the lamina bounded by the graphs of the equations 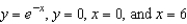 for the density
for the density 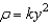 .
.
(Multiple Choice)
4.9/5  (33)
(33)
Find the center of mass of the rectangular lamina with vertices 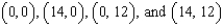 for the density
for the density 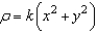 .
.
(Multiple Choice)
4.8/5  (36)
(36)
Determine the location of the horizontal axis  for figure (b) at which a vertical gate in a dam is to be hinged so that there is no moment causing rotation under the indicated loading (see figure (a)). The model for
for figure (b) at which a vertical gate in a dam is to be hinged so that there is no moment causing rotation under the indicated loading (see figure (a)). The model for 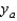 is
is  where
where 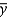 is the y-coordinate of the centroid of the gate,
is the y-coordinate of the centroid of the gate, 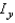 is the moment of inertia of the gate about the line
is the moment of inertia of the gate about the line 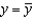 , h is the depth of the centroid below the surface, and A is the area of the gate.
, h is the depth of the centroid below the surface, and A is the area of the gate. 
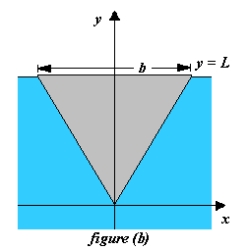
(Multiple Choice)
4.8/5  (33)
(33)
Set up the double integral required to find the moment of inertia I, about the line 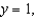 of the lamina bounded by the graphs of the equations
of the lamina bounded by the graphs of the equations  for the density
for the density 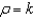 . Use a computer algebra system to evaluate the double integral.
. Use a computer algebra system to evaluate the double integral.
(Multiple Choice)
4.8/5  (26)
(26)
Find the mass of the lamina described by the inequalities  given that its density is
given that its density is 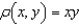 . (Hint: Some of the integrals are simpler in polar coordinates.)
. (Hint: Some of the integrals are simpler in polar coordinates.)
(Multiple Choice)
4.9/5  (31)
(31)
Find the mass of the triangular lamina with vertices 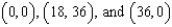 for the density
for the density 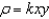 .
.
(Multiple Choice)
4.9/5  (32)
(32)
Find the mass of the lamina bounded by the graphs of the equations  for the density
for the density  .
.
(Multiple Choice)
4.9/5  (35)
(35)
Find the mass and moments of inertia of the lamina, with given density, bounded by the graphs of the equations given below and use them to find the radii of gyration about the axes. 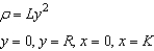
(Multiple Choice)
4.9/5  (38)
(38)
Set up and evaluate a double integral required to find the moment of inertia, I, about the given line, of the lamina bounded by the graphs of the following equations. Use a computer algebra system to evaluate the double integral. 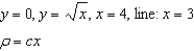
(Multiple Choice)
4.8/5  (30)
(30)
Find the mass and moments of inertia of the lamina, of given density, bounded by the graphs of the equations given below and use them to find the radii of gyration about the axes. 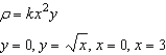
(Multiple Choice)
4.9/5  (30)
(30)
Find the mass of the lamina described by the inequalities 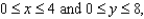 given that its density is
given that its density is 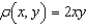 . (Hint: Some of the integrals are simpler in polar coordinates.)
. (Hint: Some of the integrals are simpler in polar coordinates.)
(Multiple Choice)
4.9/5  (30)
(30)
Find the center of mass of the lamina bounded by the graphs of the equations  for the density
for the density 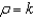 .
.
(Multiple Choice)
4.9/5  (33)
(33)
Find the center of mass of the rectangular lamina with vertices 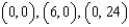 and
and 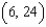 for the density
for the density  .
.
(Multiple Choice)
4.9/5  (40)
(40)
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)