Exam 11: Equilibrium and Elasticity
Exam 2: Motion Along a Straight Line55 Questions
Exam 3: Motion in Two or Three Dimensions59 Questions
Exam 4: Newtons Laws of Motion50 Questions
Exam 5: Applying Newtons Laws139 Questions
Exam 6: Work and Kinetic Energy109 Questions
Exam 7: Potential Energy and Energy Conservation50 Questions
Exam 8: Momentum, Impulse, and Collisions99 Questions
Exam 9: Rotation of Rigid Bodies26 Questions
Exam 10: Dynamics of Rotational Motion49 Questions
Exam 11: Equilibrium and Elasticity50 Questions
Exam 12: Fluid Mechanics54 Questions
Exam 13: Gravitation52 Questions
Exam 14: Periodic Motion109 Questions
Exam 15: Mechanical Waves50 Questions
Exam 16: Sound and Hearing121 Questions
Exam 17: Temperature and Heat60 Questions
Exam 18: Thermal Properties of Matter41 Questions
Exam 19: The First Law of Thermodynamics55 Questions
Exam 20: The Second Law of Thermodynamics52 Questions
Exam 21: Electric Charge and Electric Field54 Questions
Exam 22: Gausss Law54 Questions
Exam 23: Electric Potential88 Questions
Exam 24: Capacitance and Dielectrics70 Questions
Exam 25: Current, Resistance, and Electromotive Force44 Questions
Exam 26: Direct-Current Circuits51 Questions
Exam 27: Magnetic Field and Magnetic Forces105 Questions
Exam 28: Sources of Magnetic Field82 Questions
Exam 29: Electromagnetic Induction51 Questions
Exam 30: Inductance88 Questions
Exam 31: Alternating Current51 Questions
Exam 32: Electromagnetic Waves Optics53 Questions
Exam 33: The Nature and Propagation of Light31 Questions
Exam 34: Geometric Optics89 Questions
Exam 35: Interference59 Questions
Select questions type
Energy in SHM: A 0.25 kg ideal harmonic oscillator has a total mechanical energy of 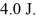 If the oscillation amplitude is
If the oscillation amplitude is 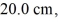 what is the oscillation frequency?
what is the oscillation frequency?
(Multiple Choice)
4.7/5  (39)
(39)
Energy in SHM: If we double only the mass of a vibrating ideal mass-and-spring system, the mechanical energy of the system
(Multiple Choice)
5.0/5  (39)
(39)
Mass on a spring: A 2.00-kg object is attached to an ideal massless horizontal spring of spring constant 100.0 N/m and is at rest on a frictionless horizontal table. The spring is aligned along the x-axis and is fixed to a peg in the table. Suddenly this mass is struck by another 2.00-kg object traveling along the x-axis at 3.00 m/s, and the two masses stick together. What are the amplitude and period of the oscillations that result from this collision?
(Multiple Choice)
4.7/5  (36)
(36)
Simple pendulum: A frictionless simple pendulum on Earth has a period of 1.75 s. On Planet X its period is 2.14 s. What is the acceleration due to gravity on Planet X?
(Short Answer)
4.8/5  (40)
(40)
Simple pendulum: In the figure, a 0.24-kg ball is suspended from a very light string 9.79 m long and is pulled slightly to the left. As the ball swings without friction through the lowest part of its motion it encounters an ideal massless spring attached to the wall. The spring pushes against the ball and eventually the ball is returned to its original starting position. Find the time for one complete cycle of this motion if the spring constant of the spring is 21 N/m. (Assume that once the pendulum ball hits the spring there is no effect due to the vertical movement of the ball.) 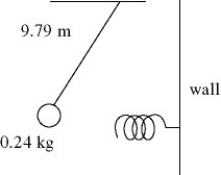
(Short Answer)
4.9/5  (44)
(44)
Mass on a spring: A 0.28-kg block on a horizontal frictionless surface is attached to an ideal massless spring whose spring constant is 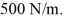 The block is pulled from its equilibrium position at x = 0.00 m to a displacement x = +0.080 m and is released from rest. The block then executes simple harmonic motion along the horizontal x-axis. When the displacement is
The block is pulled from its equilibrium position at x = 0.00 m to a displacement x = +0.080 m and is released from rest. The block then executes simple harmonic motion along the horizontal x-axis. When the displacement is 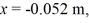 find the acceleration of the block.
find the acceleration of the block.
(Multiple Choice)
4.8/5  (38)
(38)
Simple harmonic motion: An object is executing simple harmonic motion. What is true about the acceleration of this object? (There may be more than one correct choice.)
(Multiple Choice)
4.9/5  (27)
(27)
Mass on a spring: A 12.0-N object is oscillating in simple harmonic motion at the end of an ideal vertical spring. Its vertical position y as a function of time t is given by y(t) = 4.50 cm cos[(19.5 s-1)t - π/8].
(a) What is the spring constant of the spring?
(b) What is the maximum acceleration of the object?
(c) What is the maximum speed that the object reaches?
(d) How long does it take the object to go from its highest point to its lowest point?
(Essay)
4.7/5  (30)
(30)
Mass on a spring: A 2.25-kg object is attached to a horizontal an ideal massless spring on a frictionless table. What should be the spring constant of this spring so that the maximum acceleration of the object will be g when it oscillates with amplitude of 4.50 cm?
(Short Answer)
4.9/5  (37)
(37)
Mass on a spring: A 2.0 kg block on a frictionless table is connected to two ideal massless springs with spring constants k1 and k2 whose opposite ends are fixed to walls, as shown in the figure. What is angular frequency of the oscillation if 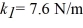 and
and 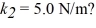
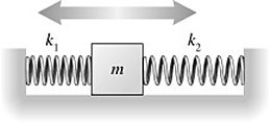
(Multiple Choice)
4.8/5  (37)
(37)
Showing 41 - 50 of 50
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)