Exam 10: Conics and Calculus
Exam 1: Graphs and Models114 Questions
Exam 2: A Preview of Calculus92 Questions
Exam 3: The Derivative and the Tangent Line Problem191 Questions
Exam 4: Extrema on an Interval147 Questions
Exam 5: Antiderivatives and Indefinite Integration167 Questions
Exam 6: Slope Fields and Eulers Method85 Questions
Exam 7: Area of a Region Between Two Curves120 Questions
Exam 8: Basic Integration Rules127 Questions
Exam 9: Sequences179 Questions
Exam 10: Conics and Calculus120 Questions
Exam 11: Vectors in the Plane125 Questions
Exam 12: Vector-Valued Functions83 Questions
Exam 13: Introduction to Functions of Several Variables124 Questions
Exam 14: Iterated Integrals and Area in the Plane118 Questions
Exam 15: Vector Fields108 Questions
Exam 16: Exact First-Order Equations45 Questions
Select questions type
Write the corresponding rectangular equation for the curve represented by the parametric equations
by eliminating the parameter.
(Multiple Choice)
4.8/5  (33)
(33)
Find a polar equation for the parabola with its focus at the pole and vertex
(Multiple Choice)
4.8/5  (26)
(26)
Find the area of the common interior of the polar equations and by sketching the graph of the equations using the graphing utility.
(Multiple Choice)
4.9/5  (33)
(33)
Find the area of the surface generated by revolving the curve about the given axis.
(i) -axis;(ii) -axis
(Multiple Choice)
4.7/5  (40)
(40)
Find the center, foci, vertices, and eccentricity of the ellipse.
(Multiple Choice)
4.9/5  (39)
(39)
Find a polar equation for the parabola with its focus at the pole, eccentricity e = 1, and directrix .
(Multiple Choice)
4.8/5  (31)
(31)
Find the distance from the pole to the directrix for the conic
(Multiple Choice)
4.9/5  (30)
(30)
The path of a projectile is modeled by the parametric equations where and are measured in feet. Use a graphing utility to approximate the range of the projectile. Round your answer to two decimal places.
(Multiple Choice)
4.7/5  (28)
(28)
Suppose a cable of a suspension bridge is suspended (in the shape of a parabola) between two towers that are 240 meters apart and 40 meters above the roadway as shown in the figure Given below. The cable touches the roadway midway between the towers. Find an equation for the Parabolic shape of cable.
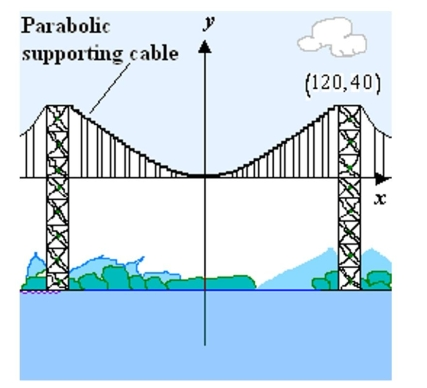
(Multiple Choice)
4.7/5  (38)
(38)
Find a set of parametric equations for the rectangular equation the condition at the point .
(Multiple Choice)
4.8/5  (37)
(37)
Find the area of the surface generated by revolving the curve -axis on the interval . Round your answer to two decimal places.
(Multiple Choice)
4.8/5  (38)
(38)
The parametric equations for the path of a projectile launched at a height h feet above the ground, at an angle with the horizontal and having an initial velocity of feet per second is given by and . The center field fence in a ballpark is 10 feet high and 400 feet from home plate. The ball is hit 3 feet above the ground. It leaves the bat at an angle of degrees with the horizontal at a speed of 100 miles per hour as shown in the figure. Find the minimum angle at which the ball must leave the bat in order for the hit to be a home run using the parametric equations and . Round your answer to one decimal place.
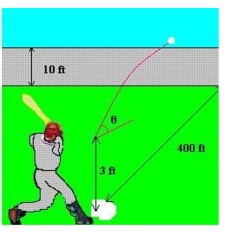
(Multiple Choice)
4.9/5  (30)
(30)
Find the area of the surface generated by revolving the curve -axis on the interval . Round your answer to two decimal places.
(Multiple Choice)
4.7/5  (32)
(32)
Suppose a church window is bounded above by a parabola and below by the arc of a circle as shown in figure given below. Find the surface area of the window. Round your answer to Three decimal places. 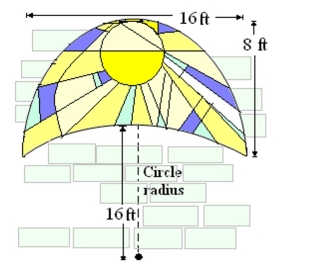
(Multiple Choice)
4.8/5  (32)
(32)
Showing 81 - 100 of 120
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)