Exam 10: Approximating Functions Using Series
Exam 1: A Library of Functions110 Questions
Exam 2: Key Concept: the Derivative92 Questions
Exam 3: Short-Cuts to Differentiation175 Questions
Exam 4: Using the Derivative108 Questions
Exam 5: Key Concept- the Definite Integral62 Questions
Exam 6: Constructing Antiderivatives90 Questions
Exam 7: Integration179 Questions
Exam 8: Using the Definite Integral104 Questions
Exam 9: Sequences and Series70 Questions
Exam 10: Approximating Functions Using Series71 Questions
Exam 11: Differential Equations135 Questions
Exam 12: Functions of Several Variables93 Questions
Exam 13: A Fundamental Tool- Vectors107 Questions
Exam 14: Differentiating Functions of Several Variables129 Questions
Exam 15: Optimization- Local and Global Extrema77 Questions
Exam 16: Integrating Functions of Several Variables76 Questions
Exam 17: Parameterization and Vector Fields86 Questions
Exam 18: Line Integrals78 Questions
Exam 19: Flux Integrals and Divergence52 Questions
Exam 20: The Curl and Stokes Theorem84 Questions
Exam 21: Parameters, Coordinates, Integrals23 Questions
Select questions type
Solve for x.Round to 2 decimal places.
Free
(Short Answer)
4.9/5  (32)
(32)
Correct Answer:
0.88
The function h(x)is a continuous differentiable function whose graph is drawn below.The accompanying table provides some information about h(x)and its derivatives. 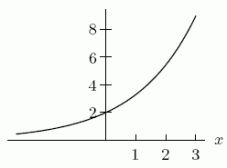 ( ) ( ) ( ) ( ) 0 2 1 0.50 0.25 1 3.29 1.64 0.82 0.41 2 5.43 2.71 1.35 0.67 3 8.96 4.48 2.24 1.12 h(x), h'(x), h"(x)and h"'(x)are all increasing functions.Suppose we use a tangent line approximation at zero to approximate h(0.1).Find a good upper bound for the error.
( ) ( ) ( ) ( ) 0 2 1 0.50 0.25 1 3.29 1.64 0.82 0.41 2 5.43 2.71 1.35 0.67 3 8.96 4.48 2.24 1.12 h(x), h'(x), h"(x)and h"'(x)are all increasing functions.Suppose we use a tangent line approximation at zero to approximate h(0.1).Find a good upper bound for the error.
Free
(Multiple Choice)
4.9/5  (47)
(47)
Correct Answer:
B
Use the derivative of the Taylor series about 0 for to find the Taylor series about 0 for .
(Multiple Choice)
4.9/5  (39)
(39)
Find an expression for the general term of the Taylor series for .
(Multiple Choice)
4.8/5  (41)
(41)
Find the Taylor series centered at (i.e.the Maclaurin series)for .
(Multiple Choice)
4.7/5  (43)
(43)
According to the theory of relativity, the energy, E, of a body of mass m is given as a function of its speed, v, by , where c is a constant, the speed of light.Assuming v < c, expand E as a series in v/c, as far as the second non-zero term.
(Multiple Choice)
4.8/5  (32)
(32)
Find the second harmonic of the function -\pi< 0< x\leq0 x\leq\pi .
(Multiple Choice)
4.8/5  (40)
(40)
The function g has the Taylor approximation and the graph given below: 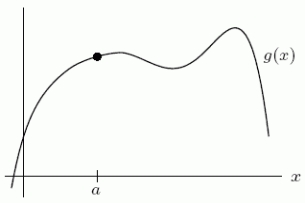 Is c0 positive, negative, or zero?
Is c0 positive, negative, or zero?
(Short Answer)
4.7/5  (30)
(30)
Use the first three nonzero terms of the Taylor polynomial to approximate .Give your answer to 5 decimal places.
(Multiple Choice)
4.9/5  (38)
(38)
Find the Taylor polynomial of degree 3 around x = 0 for the function and use it to approximate .Give your answer to 4 decimal places.
(Short Answer)
4.9/5  (43)
(43)
Find the fourth term of the Taylor series for the function about .
(Multiple Choice)
4.7/5  (32)
(32)
Construct the Taylor polynomial approximation of degree 3 to the function about the point x = 0.Use it to approximate the value to 5 decimal places.How does the approximation compare to the actual value?
(Short Answer)
4.9/5  (42)
(42)
Fill in the blanks: Fourier polynomials give good __________ approximations to a function.Taylor polynomials give good _____________ approximations to a function.
(Short Answer)
4.9/5  (39)
(39)
Use the Maclaurin series for to find the Maclaurin series for .
(Multiple Choice)
4.8/5  (41)
(41)
Use the binomial series to find the coefficient of the term in the expansion of .
(Short Answer)
4.7/5  (38)
(38)
The graph of the function is a bell-shaped curve similar to a normal probability density function.Is the Maclaurin series for ?
(True/False)
4.8/5  (37)
(37)
Recognize as a Taylor series evaluated at a particular value of x and find the sum to 4 decimal places.
(Short Answer)
4.7/5  (38)
(38)
Showing 1 - 20 of 71
Filters
- Essay(0)
- Multiple Choice(0)
- Short Answer(0)
- True False(0)
- Matching(0)